Pentagonal pyramid (original) (raw)

From Wikipedia, the free encyclopedia

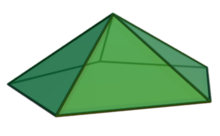
Pyramid with a pentagon base

Pentagonal pyramid
Type PyramidJohnson_J_1 – _J_2 – _J_3
Faces 5 triangles1 pentagon
Edges 10
Vertices 6
Vertex configuration 5 × ( 3 2 × 5 ) + 1 × 3 5 {\displaystyle 5\times (3^{2}\times 5)+1\times 3^{5}} {\displaystyle 5\times (3^{2}\times 5)+1\times 3^{5}}[1]
Symmetry group C 5 v {\displaystyle C_{5\mathrm {v} }} {\displaystyle C_{5\mathrm {v} }}
Dihedral angle (degrees) As a Johnson solid:triangle-to-triangle: 138.19°triangle-to-pentagon: 37.37°
Dual polyhedron self-dual
Properties convex,elementary (Johnson solid)
Net

In geometry, pentagonal pyramid is a pyramid with a pentagon base and five triangular faces, having a total of six faces. It is categorized as a Johnson solid if all of the edges are equal in length, forming equilateral triangular faces and a regular pentagonal base. The pentagonal pyramid can be found in many polyhedrons, including their construction. It also occurs in stereochemistry in pentagonal pyramidal molecular geometry.

A pentagonal pyramid has six vertices, ten edges, and six faces. One of its faces is pentagon, a base of the pyramid; five others are triangles.[2] Five of the edges make up the pentagon by connecting its five vertices, and the other five edges are known as the lateral edges of the pyramid, meeting at the sixth vertex called the apex.[3] A pentagonal pyramid is said to be regular if its base is circumscribed in a circle that forms a regular pentagon, and it is said to be right if its altitude is erected perpendicularly to the base's center.[4]

Like other right pyramids with a regular polygon as a base, this pyramid has pyramidal symmetry of cyclic group C 5 v {\displaystyle C_{5\mathrm {v} }} {\displaystyle C_{5\mathrm {v} }}: the pyramid is left invariant by rotations of one, two, three, and four in five of a full turn around its axis of symmetry, the line connecting the apex to the center of the base. It is also mirror symmetric relative to any perpendicular plane passing through a bisector of the base.[1] It can be represented as the wheel graph W 5 {\displaystyle W_{5}} {\displaystyle W_{5}}, meaning its skeleton can be interpreted as a pentagon in which its five vertices connects a vertex in the center called the universal vertex.[5] It is self-dual, meaning its dual polyhedron is the pentagonal pyramid itself.[6]

3D model of a pentagonal pyramid

When all edges are equal in length, the five triangular faces are equilateral and the base is a regular pentagon. Because this pyramid remains convex and all of its faces are regular polygons, it is classified as the second Johnson solid J 2 {\displaystyle J_{2}} {\displaystyle J_{2}}.[7] The dihedral angle between two adjacent triangular faces is approximately 138.19° and that between the triangular face and the base is 37.37°.[1] It is elementary polyhedra, meaning it cannot be separated by a plane to create two small convex polyhedrons with regular faces.[8] Given that a {\displaystyle a} {\displaystyle a} is the length of all edges of the pentagonal pyramid. A polyhedron's surface area is the sum of the areas of its faces. Therefore, the surface area of a pentagonal pyramid is the sum of the four triangles and one pentagon area. The volume of every pyramid equals one-third of the area of its base multiplied by its height. That is, the volume of a pentagonal pyramid is one-third of the product of the height and a pentagonal pyramid's area.[9] In the case of Johnson solid with edge length a {\displaystyle a} {\displaystyle a}, its surface area A {\displaystyle A} {\displaystyle A} and volume V {\displaystyle V} {\displaystyle V} are:[10] A = a 2 2 5 2 ( 10 + 5 + 75 + 30 5 ) ≈ 3.88554 a 2 , V = 5 + 5 24 a 3 ≈ 0.30150 a 3 . {\displaystyle {\begin{aligned}A&={\frac {a^{2}}{2}}{\sqrt {{\frac {5}{2}}\left(10+{\sqrt {5}}+{\sqrt {75+30{\sqrt {5}}}}\right)}}\approx 3.88554a^{2},\\V&={\frac {5+{\sqrt {5}}}{24}}a^{3}\approx 0.30150a^{3}.\end{aligned}}} {\displaystyle {\begin{aligned}A&={\frac {a^{2}}{2}}{\sqrt {{\frac {5}{2}}\left(10+{\sqrt {5}}+{\sqrt {75+30{\sqrt {5}}}}\right)}}\approx 3.88554a^{2},\\V&={\frac {5+{\sqrt {5}}}{24}}a^{3}\approx 0.30150a^{3}.\end{aligned}}}

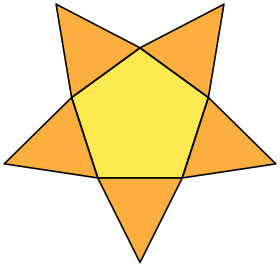
Pentagonal pyramids can be found in a small stellated dodecahedron

Pentagonal pyramids can be found as components of many polyhedrons. Attaching its base to the pentagonal face of another polyhedron is an example of the construction process known as augmentation, and attaching it to prisms or antiprisms is known as elongation or gyroelongation, respectively.[11] Examples of polyhedrons are the pentakis dodecahedron is constructed from the dodecahedron by attaching the base of pentagonal pyramids onto each pentagonal face, small stellated dodecahedron is constructed from a regular dodecahedron stellated by pentagonal pyramids, and regular icosahedron constructed from a pentagonal antiprism by attaching two pentagonal pyramids onto its pentagonal bases.[12] Some Johnson solids are constructed by either augmenting pentagonal pyramids or augmenting other shapes with pentagonal pyramids: elongated pentagonal pyramid J 9 {\displaystyle J_{9}} {\displaystyle J_{9}}, gyroelongated pentagonal pyramid J 11 {\displaystyle J_{11}} {\displaystyle J_{11}}, pentagonal bipyramid J 13 {\displaystyle J_{13}} {\displaystyle J_{13}}, elongated pentagonal bipyramid J 16 {\displaystyle J_{16}} {\displaystyle J_{16}}, augmented dodecahedron J 58 {\displaystyle J_{58}} {\displaystyle J_{58}}, parabiaugmented dodecahedron J 59 {\displaystyle J_{59}} {\displaystyle J_{59}}, metabiaugmented dodecahedron J 60 {\displaystyle J_{60}} {\displaystyle J_{60}}, and triaugmented dodecahedron J 61 {\displaystyle J_{61}} {\displaystyle J_{61}}.[13] Relatedly, the removal of a pentagonal pyramid from polyhedra is an example known as diminishment; metabidiminished icosahedron J 62 {\displaystyle J_{62}} {\displaystyle J_{62}} and tridiminished icosahedron J 63 {\displaystyle J_{63}} {\displaystyle J_{63}} are the examples in which their constructions begin by removing pentagonal pyramids from a regular icosahedron.[14]

In stereochemistry, an atom cluster can have a pentagonal pyramidal geometry. This molecule has a main-group element with one active lone pair, which can be described by a model that predicts the geometry of molecules known as VSEPR theory.[15] An example of a molecule with this structure include nido-cage carbonate CB5H9.[16]

  1. ^ a b c Johnson (1966).
  2. ^
  3. ^ Smith (2000), p. 98.
  4. ^
  5. ^ Pisanski & Servatius (2013), p. 21.
  6. ^ Wohlleben (2019), p. 485–486.
  7. ^ Uehara (2020), p. 62.
  8. ^
  9. ^ Calter & Calter (2011), p. 198.
  10. ^ Berman (1971).
  11. ^ Slobodan, Obradović & Ðukanović (2015).
  12. ^
  1. ^ Rajwade (2001), pp. 84–88. See Table 12.3, where P n {\displaystyle P_{n}} {\displaystyle P_{n}} denotes the n {\displaystyle n} {\displaystyle n}-sided prism and A n {\displaystyle A_{n}} {\displaystyle A_{n}} denotes the n {\displaystyle n} {\displaystyle n}-sided antiprism.
  2. ^ Gailiunas (2001).
  3. ^ Petrucci, Harwood & Herring (2002), p. 414.
  4. ^ Macartney (2017), p. 482.