Jonathan Shewchuk's papers (original) (raw)
 |
(Meditations at the Edge: Paper & Spirit, Peter and Donna Thomas.) Jonathan's papers |
|---|
If you're in Soda Hall, you'll find copies of most of my papers hanging outside my office (625). Take the ones you want.FAR AND AWAY MY MOST POPULAR PAPER is my introduction to the conjugate gradient method. This report is an exercise in trying to make a difficult subject as transparent and easy to understand as humanly possible. It includes sixty-six illustrations and as much intuition as I can provide. How could fifteen lines of pseudocode take fifty pages to explain?
Also available is a set of full-page figures from the paper, which may be printed on transparencies for classroom use.
 Jonathan Richard Shewchuk, An Introduction to the Conjugate Gradient Method Without the Agonizing Pain, August 1994. Abstract, PostScript (1,716k, 58 pages), PDF (516k, 58 pages), PostScript of classroom figures (1,409k, 37 pages). PDF of classroom figures (394k, 37 pages).
Jonathan Richard Shewchuk, An Introduction to the Conjugate Gradient Method Without the Agonizing Pain, August 1994. Abstract, PostScript (1,716k, 58 pages), PDF (516k, 58 pages), PostScript of classroom figures (1,409k, 37 pages). PDF of classroom figures (394k, 37 pages).
Delaunay Mesh Generation
“I hate meshes. I cannot believe how hard this is. Geometry is hard.”
— David Baraff, Senior Research Scientist, Pixar Animation Studios
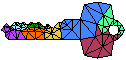 DELAUNAY REFINEMENT MESH GENERATION ALGORITHMSconstruct meshes of triangles or tetrahedra (“elements”) that are suitable for applications like interpolation, rendering, terrain databases, geographic information systems, and most demandingly, the solution of partial differential equations by the finite element method. Delaunay refinement algorithms operate by maintaining a Delaunay or constrained Delaunay triangulation which is refined by inserting additional vertices until the mesh meets constraints on element quality and size. These algorithms simultaneously offer theoretical bounds on element quality, edge lengths, and spatial grading of element sizes; the ability to triangulate general straight-line domains (and not just polygons/polyhedra with holes); and truly satisfying performance in practice.
DELAUNAY REFINEMENT MESH GENERATION ALGORITHMSconstruct meshes of triangles or tetrahedra (“elements”) that are suitable for applications like interpolation, rendering, terrain databases, geographic information systems, and most demandingly, the solution of partial differential equations by the finite element method. Delaunay refinement algorithms operate by maintaining a Delaunay or constrained Delaunay triangulation which is refined by inserting additional vertices until the mesh meets constraints on element quality and size. These algorithms simultaneously offer theoretical bounds on element quality, edge lengths, and spatial grading of element sizes; the ability to triangulate general straight-line domains (and not just polygons/polyhedra with holes); and truly satisfying performance in practice.
The following papers include theoretical treatments of Delaunay refinement and discussions of the implementation details of my two-dimensional mesh generator and Delaunay triangulator, Triangle, and my three-dimensional mesh generator and Delaunay tetrahedralizer,Pyramid. See the Triangle page for information about what Triangle can do, or to obtain the C source code.
 Delaunay Refinement Algorithms for Triangular Mesh Generation, Computational Geometry: Theory and Applications 22(1-3):21-74, May 2002. PostScript (5,128k, 54 pages), PDF (1,046k, 54 pages). My ultimate article on two-dimensional Delaunay refinement, including a full theoretical treatment plus extensive pseudocode. This is the first one to read if you want to implement a triangular Delaunay refinement mesh generator. (See Chapter 5 of my dissertation for data structures, though.)
Delaunay Refinement Algorithms for Triangular Mesh Generation, Computational Geometry: Theory and Applications 22(1-3):21-74, May 2002. PostScript (5,128k, 54 pages), PDF (1,046k, 54 pages). My ultimate article on two-dimensional Delaunay refinement, including a full theoretical treatment plus extensive pseudocode. This is the first one to read if you want to implement a triangular Delaunay refinement mesh generator. (See Chapter 5 of my dissertation for data structures, though.) Delaunay Refinement Mesh Generation, Ph.D. thesis, Technical Report CMU-CS-97-137, School of Computer Science, Carnegie Mellon University, Pittsburgh, Pennsylvania, 18 May 1997. Abstract (with BibTeX citation), compressed PostScript (1,768k, 207 pages)(expands to 10,435k when uncompressed), PDF (2,635k, 207 pages). Includes extensive treatment of Delaunay triangulations, two- and three-dimensional Delaunay refinement algorithms, and implementation details. However, Chapter 3 on two-dimensional Delaunay refinement is superseded by the much-improved article above.
Delaunay Refinement Mesh Generation, Ph.D. thesis, Technical Report CMU-CS-97-137, School of Computer Science, Carnegie Mellon University, Pittsburgh, Pennsylvania, 18 May 1997. Abstract (with BibTeX citation), compressed PostScript (1,768k, 207 pages)(expands to 10,435k when uncompressed), PDF (2,635k, 207 pages). Includes extensive treatment of Delaunay triangulations, two- and three-dimensional Delaunay refinement algorithms, and implementation details. However, Chapter 3 on two-dimensional Delaunay refinement is superseded by the much-improved article above. Tetrahedral Mesh Generation by Delaunay Refinement, Proceedings of the Fourteenth Annual Symposium on Computational Geometry (Minneapolis, Minnesota), pages 86-95, Association for Computing Machinery, June 1998. PostScript (1,504k, 10 pages), PDF (299k, 10 pages). A description of the core three-dimensional mesh generation algorithm used in Pyramid, for those who want a quick overview with less detail. A more thorough treatment appears in Chapter 4 of my dissertation.
Tetrahedral Mesh Generation by Delaunay Refinement, Proceedings of the Fourteenth Annual Symposium on Computational Geometry (Minneapolis, Minnesota), pages 86-95, Association for Computing Machinery, June 1998. PostScript (1,504k, 10 pages), PDF (299k, 10 pages). A description of the core three-dimensional mesh generation algorithm used in Pyramid, for those who want a quick overview with less detail. A more thorough treatment appears in Chapter 4 of my dissertation. Triangle: Engineering a 2D Quality Mesh Generator and Delaunay Triangulator, in Applied Computational Geometry: Towards Geometric Engineering (Ming C. Lin and Dinesh Manocha, editors), volume 1148 of Lecture Notes in Computer Science, pages 203-222, Springer-Verlag (Berlin), May 1996.From the First Workshop on Applied Computational Geometry (Philadelphia, Pennsylvania). Abstract (with BibTeX citation), PostScript (513k, 10 pages), PDF (153k, 10 pages), HTML. A short paper on Triangle, for those who want a quick overview with less detail. All this material is scattered through my dissertation as well.
Triangle: Engineering a 2D Quality Mesh Generator and Delaunay Triangulator, in Applied Computational Geometry: Towards Geometric Engineering (Ming C. Lin and Dinesh Manocha, editors), volume 1148 of Lecture Notes in Computer Science, pages 203-222, Springer-Verlag (Berlin), May 1996.From the First Workshop on Applied Computational Geometry (Philadelphia, Pennsylvania). Abstract (with BibTeX citation), PostScript (513k, 10 pages), PDF (153k, 10 pages), HTML. A short paper on Triangle, for those who want a quick overview with less detail. All this material is scattered through my dissertation as well. François Labelle and Jonathan Richard Shewchuk,Anisotropic Voronoi Diagrams and Guaranteed-Quality Anisotropic Mesh Generation, Proceedings of the Nineteenth Annual Symposium on Computational Geometry (San Diego, California), pages 191-200, Association for Computing Machinery, June 2003. PostScript (910k, 10 pages), PDF (284k, 10 pages). The best triangulations for interpolation and numerical modeling are often anisotropic: long and skinny, oriented in directions dictated by the function being approximated. (See my “What Is a Good Linear Element?” papers below for details.) The ideal orientations and aspect ratios of the elements may vary greatly from one position to another. This paper discusses a Voronoi refinement algorithm for provably good anisotropic mesh generation. The skewed elements are generated with the help of_anisotropic Voronoi diagrams_, wherein each site has its own distorted distance metric. Anisotropic Voronoi diagrams are somewhat badly behaved, and do not always dualize to triangulations. We call it “Voronoi refinement” because the diagrams can be tamed by inserting new sites. After they are carefully refined, they dualize to guaranteed-quality anisotropic triangular meshes.
François Labelle and Jonathan Richard Shewchuk,Anisotropic Voronoi Diagrams and Guaranteed-Quality Anisotropic Mesh Generation, Proceedings of the Nineteenth Annual Symposium on Computational Geometry (San Diego, California), pages 191-200, Association for Computing Machinery, June 2003. PostScript (910k, 10 pages), PDF (284k, 10 pages). The best triangulations for interpolation and numerical modeling are often anisotropic: long and skinny, oriented in directions dictated by the function being approximated. (See my “What Is a Good Linear Element?” papers below for details.) The ideal orientations and aspect ratios of the elements may vary greatly from one position to another. This paper discusses a Voronoi refinement algorithm for provably good anisotropic mesh generation. The skewed elements are generated with the help of_anisotropic Voronoi diagrams_, wherein each site has its own distorted distance metric. Anisotropic Voronoi diagrams are somewhat badly behaved, and do not always dualize to triangulations. We call it “Voronoi refinement” because the diagrams can be tamed by inserting new sites. After they are carefully refined, they dualize to guaranteed-quality anisotropic triangular meshes.- Talk slides: Anisotropic Voronoi Diagrams and Guaranteed-Quality Anisotropic Mesh Generation. PDF (color, 1,301k, 47 pages). Slides from a talk on our paper above. You can evenwatch me give a shorter version of this talk at the Mathematical Sciences Research Institute.
 Mesh Generation for Domains with Small Angles, Proceedings of the Sixteenth Annual Symposium on Computational Geometry (Hong Kong), pages 1-10, Association for Computing Machinery, June 2000. PostScript (663k, 10 pages), PDF (237k, 10 pages). How to adapt Delaunay refinement algorithms to domains that are difficult to mesh because they have small angles. The two-dimensional portion of this paper is superseded by the improved writing in “Delaunay Refinement Algorithms for Triangular Mesh Generation,” above. The three-dimensional portion is still found only here.
Mesh Generation for Domains with Small Angles, Proceedings of the Sixteenth Annual Symposium on Computational Geometry (Hong Kong), pages 1-10, Association for Computing Machinery, June 2000. PostScript (663k, 10 pages), PDF (237k, 10 pages). How to adapt Delaunay refinement algorithms to domains that are difficult to mesh because they have small angles. The two-dimensional portion of this paper is superseded by the improved writing in “Delaunay Refinement Algorithms for Triangular Mesh Generation,” above. The three-dimensional portion is still found only here. Star Splaying: An Algorithm for Repairing Delaunay Triangulations and Convex Hulls, Proceedings of the Twenty-First Annual Symposium on Computational Geometry (Pisa, Italy), pages 237-246, Association for Computing Machinery, June 2005. PostScript (392k, 10 pages), PDF (209k, 10 pages). Star splaying is a general-dimensional algorithm for fixing broken Delaunay triangulations and convex hulls. Its input is a triangulation, an approximate convex hull, or even just a set of vertices and guesses about who their neighbors are. If the input is “nearly Delaunay” or “nearly convex,” star splaying is quite fast, so I call it a “Delaunay repair” algorithm. Star splaying is designed for dynamic mesh generation, to repair the quality of a finite element mesh that has lost the Delaunay property after its vertices have moved in response to simulated physical forces. Star splaying is akin to Lawson's edge flip algorithm for converting a two-dimensional triangulation to a Delaunay triangulation, but it works in any dimensionality.
Star Splaying: An Algorithm for Repairing Delaunay Triangulations and Convex Hulls, Proceedings of the Twenty-First Annual Symposium on Computational Geometry (Pisa, Italy), pages 237-246, Association for Computing Machinery, June 2005. PostScript (392k, 10 pages), PDF (209k, 10 pages). Star splaying is a general-dimensional algorithm for fixing broken Delaunay triangulations and convex hulls. Its input is a triangulation, an approximate convex hull, or even just a set of vertices and guesses about who their neighbors are. If the input is “nearly Delaunay” or “nearly convex,” star splaying is quite fast, so I call it a “Delaunay repair” algorithm. Star splaying is designed for dynamic mesh generation, to repair the quality of a finite element mesh that has lost the Delaunay property after its vertices have moved in response to simulated physical forces. Star splaying is akin to Lawson's edge flip algorithm for converting a two-dimensional triangulation to a Delaunay triangulation, but it works in any dimensionality.- Talk slides: Theoretically Guaranteed Delaunay Mesh Generation—In Practice,September 2005. PDF (color, 2,003k, 106 pages). Slides from a short course I gave at the Thirteenth and Fourteenth International Meshing Roundtables. Not just about my work! This is a survey of what I see as the most central contributions to provably good mesh generation, restricted to algorithms that have been demonstrated to work in practice as well as in theory. It includes full citations for all the featured algorithms, and is designed to be a fast, readable introduction to the area. Topics covered include a short review of Delaunay triangulations and constrained Delaunay triangulations; extensive coverage of Delaunay refinement algorithms for triangular and tetrahedral mesh generation, including methods by Chew, Ruppert, Boivin/Ollivier-Gooch, Miller/Walkington/Pav, Üngör, and me; handling of 2D domains with curved boundaries; handling of 2D and 3D domains with small angles; sliver elimination; and a brief discussion of how to prove that a Delaunay refinement algorithm eliminates bad elements.
Non-Delaunay Mesh Generation
THE GREAT CHALLENGE OF TETRAHEDRAL MESH GENERATIONis to create tetrahedra whose dihedral angles are never too small nor too large. Although Delaunay refinement usually does this well in practice, there's lots of room for improvement—both in obtaining mathematically guaranteed bounds on angles, and in further improving the angles beyond what Delaunay algorithms or guaranteed-quality algorithms can provide. Our isosurface stuffing algorithm comes with theoretical guarantees on dihedral angles. Through mesh improvement procedures, we can make them even better in practice.
 François Labelle and Jonathan Richard Shewchuk,Isosurface Stuffing: Fast Tetrahedral Meshes with Good Dihedral Angles, ACM Transactions on Graphics 26(3):57.1-57.10, August 2007. Special issue on Proceedings of SIGGRAPH 2007. PDF (color, 3,530k, 10 pages). The isosurface stuffing algorithm fills an isosurface with a mesh whose dihedral angles are bounded between 10.7o and 164.8o. We're pretty proud of this, because virtually nobody has been able to prove dihedral angle bounds anywhere close to this, except for very simple geometries. Although the tetrahedra at the isosurface must be uniformly sized, the tetrahedra in the interior can be graded. The algorithm is whip fast, numerically robust, and easy to implement because, like Marching Cubes, it generates tetrahedra from a small set of precomputed stencils. The angle bounds are guaranteed by a computer-assisted proof. If the isosurface is a smooth 2-manifold with bounded curvature, and the tetrahedra are sufficiently small, then the boundary of the mesh is guaranteed to be a geometrically and topologically accurate approximation of the isosurface. Unfortunately, the algorithm rounds off sharp corners and edges. (I think it will be extremely hard for anyone to devise an algorithm that provably obtains dihedral angle bounds of this order _and_conforms perfectly to creases.)
François Labelle and Jonathan Richard Shewchuk,Isosurface Stuffing: Fast Tetrahedral Meshes with Good Dihedral Angles, ACM Transactions on Graphics 26(3):57.1-57.10, August 2007. Special issue on Proceedings of SIGGRAPH 2007. PDF (color, 3,530k, 10 pages). The isosurface stuffing algorithm fills an isosurface with a mesh whose dihedral angles are bounded between 10.7o and 164.8o. We're pretty proud of this, because virtually nobody has been able to prove dihedral angle bounds anywhere close to this, except for very simple geometries. Although the tetrahedra at the isosurface must be uniformly sized, the tetrahedra in the interior can be graded. The algorithm is whip fast, numerically robust, and easy to implement because, like Marching Cubes, it generates tetrahedra from a small set of precomputed stencils. The angle bounds are guaranteed by a computer-assisted proof. If the isosurface is a smooth 2-manifold with bounded curvature, and the tetrahedra are sufficiently small, then the boundary of the mesh is guaranteed to be a geometrically and topologically accurate approximation of the isosurface. Unfortunately, the algorithm rounds off sharp corners and edges. (I think it will be extremely hard for anyone to devise an algorithm that provably obtains dihedral angle bounds of this order _and_conforms perfectly to creases.) Nuttapong Chentanez, Bryan Feldman, François Labelle, James O'Brien, and Jonathan Richard Shewchuk,Liquid Simulation on Lattice-Based Tetrahedral Meshes, 2007 Symposium on Computer Animation (San Diego, California), pages 219-228, August 2007. PDF (color, 4,782k, 10 pages). Here, we use isosurface stuffing (above) as part of an algorithm for simulating liquids with free surfaces. The graded meshes allow us to maintain fine detail on the liquid surface without excessive computational cost in the interior. The rendered surface is represented as a separate surface triangulation, at an even finer detail than the surface of the tetrahedral mesh. We exploit the regularity of the meshes for fast point location, needed for semi-Lagrangian advection of the velocities and the surface itself. We also introduce a thickening strategy to prevent the liquid from breaking up into sheets or droplets so thin that they disappear, falling below the finest resolution of the mesh.
Nuttapong Chentanez, Bryan Feldman, François Labelle, James O'Brien, and Jonathan Richard Shewchuk,Liquid Simulation on Lattice-Based Tetrahedral Meshes, 2007 Symposium on Computer Animation (San Diego, California), pages 219-228, August 2007. PDF (color, 4,782k, 10 pages). Here, we use isosurface stuffing (above) as part of an algorithm for simulating liquids with free surfaces. The graded meshes allow us to maintain fine detail on the liquid surface without excessive computational cost in the interior. The rendered surface is represented as a separate surface triangulation, at an even finer detail than the surface of the tetrahedral mesh. We exploit the regularity of the meshes for fast point location, needed for semi-Lagrangian advection of the velocities and the surface itself. We also introduce a thickening strategy to prevent the liquid from breaking up into sheets or droplets so thin that they disappear, falling below the finest resolution of the mesh. Bryan Matthew Klingner and Jonathan Richard Shewchuk,Aggressive Tetrahedral Mesh Improvement, Proceedings of the 16th International Meshing Roundtable (Seattle, Washington), pages 3-23, October 2007. PDF (color, 26,567k, 18 pages).Mesh clean-up software takes an existing mesh and improves the quality of its elements by way of operations such as smoothing (moving vertices to better locations) and topological transformations (replacing a small set of tetrahedra with better ones). Here, we demonstrate algorithms and software that so aggressively improve tetrahedral meshes that we obtain quality substantially better than that produced by any previous method for tetrahedral mesh generation or mesh improvement. Our main innovation is to augment the best traditional clean-up methods (including some from the paper below) with topological transformations and combinatorial optimization algorithms that insert new vertices. Our software often improves a mesh so that all its dihedral angles are between 30o and 130o.
Bryan Matthew Klingner and Jonathan Richard Shewchuk,Aggressive Tetrahedral Mesh Improvement, Proceedings of the 16th International Meshing Roundtable (Seattle, Washington), pages 3-23, October 2007. PDF (color, 26,567k, 18 pages).Mesh clean-up software takes an existing mesh and improves the quality of its elements by way of operations such as smoothing (moving vertices to better locations) and topological transformations (replacing a small set of tetrahedra with better ones). Here, we demonstrate algorithms and software that so aggressively improve tetrahedral meshes that we obtain quality substantially better than that produced by any previous method for tetrahedral mesh generation or mesh improvement. Our main innovation is to augment the best traditional clean-up methods (including some from the paper below) with topological transformations and combinatorial optimization algorithms that insert new vertices. Our software often improves a mesh so that all its dihedral angles are between 30o and 130o. Two Discrete Optimization Algorithms for the Topological Improvement of Tetrahedral Meshes, unpublished manuscript, 2002. PostScript (295k, 11 pages), PDF (168k, 11 pages). This tutorial studies two local topological transformations for improving tetrahedral meshes: edge removal and_multi-face removal_. Given a selected edge, edge removal deletes all the tetrahedra that contain the edge, and replaces them with other tetrahedra. I work out in detail (and attempt to popularize) an algorithm of Klincsek for finding the optimal set of new tetrahedra. The multi-face removal operation is the inverse of the edge removal operation. I give a new algorithm for finding the optimal multi-face removal operation that involves a selected face of the tetrahedralization. These algorithms are part of our tetrahedral mesh improvement software described in the paper above.
Two Discrete Optimization Algorithms for the Topological Improvement of Tetrahedral Meshes, unpublished manuscript, 2002. PostScript (295k, 11 pages), PDF (168k, 11 pages). This tutorial studies two local topological transformations for improving tetrahedral meshes: edge removal and_multi-face removal_. Given a selected edge, edge removal deletes all the tetrahedra that contain the edge, and replaces them with other tetrahedra. I work out in detail (and attempt to popularize) an algorithm of Klincsek for finding the optimal set of new tetrahedra. The multi-face removal operation is the inverse of the edge removal operation. I give a new algorithm for finding the optimal multi-face removal operation that involves a selected face of the tetrahedralization. These algorithms are part of our tetrahedral mesh improvement software described in the paper above.
Streaming Computation
A STREAMING COMPUTATION MAKES A SMALL NUMBER of sequential passes over a data file (ideally, one pass), and processes the data using a memory buffer whose size is a fraction of the stream length. Streaming allows us to compute Delaunay triangulations of billions of points on an ordinary laptop computer—and amazingly, to attain faster speeds than ordinary in-core triangulators. We also have streaming implementations of several standard GIS computations, such as converting Triangulated Irregular Networks (TINs, which are unstructured triangulations used to interpolate elevation fields) into Digital Elevation Maps (DEMs), and computing isolines. A major benefit of streaming is quick feedback. For example, a user can pipe the triangulator's output to our streaming isocontour extraction module, whose output is piped to a visualization module. Isocontours begin to appear within minutes or seconds, because streaming modules produce output while still consuming input. If they look wrong, the user can abort the pipeline and restart all the streaming components with different parameters. With other methods, users must wait hours for the computations to finish before glimpsing the results.
 Martin Isenburg, Yuanxin Liu, Jonathan Shewchuk, and Jack Snoeyink,Streaming Computation of Delaunay Triangulations, ACM Transactions on Graphics 25(3):1049-1056, July 2006. Special issue on Proceedings of SIGGRAPH 2006. PDF (color, 9,175k, 8 pages). We compute a billion-triangle terrain representation for the Neuse River system from 11.2 GB of LIDAR data in 48 minutes using only 70 MB of memory on a laptop with two hard drives. This is a factor of twelve faster than the previous fastest out-of-core Delaunay triangulation software. We also construct a nine-billion-triangle, 152 GB triangulation in under seven hours using 166 MB of main memory. The main new idea in our streaming Delaunay triangulators is_spatial finalization_. We partition space into regions, and include _finalization tags_in the stream that indicate when no more points in the stream will fall in specified regions. Our triangulators certify triangles or tetrahedra as Delaunay when the finalization tags show it is safe to do so. This make it possible to write them out early, freeing up memory to read more from the input stream. Because only the unfinalized parts of a triangulation are resident in memory, the memory footprint remains small.
Martin Isenburg, Yuanxin Liu, Jonathan Shewchuk, and Jack Snoeyink,Streaming Computation of Delaunay Triangulations, ACM Transactions on Graphics 25(3):1049-1056, July 2006. Special issue on Proceedings of SIGGRAPH 2006. PDF (color, 9,175k, 8 pages). We compute a billion-triangle terrain representation for the Neuse River system from 11.2 GB of LIDAR data in 48 minutes using only 70 MB of memory on a laptop with two hard drives. This is a factor of twelve faster than the previous fastest out-of-core Delaunay triangulation software. We also construct a nine-billion-triangle, 152 GB triangulation in under seven hours using 166 MB of main memory. The main new idea in our streaming Delaunay triangulators is_spatial finalization_. We partition space into regions, and include _finalization tags_in the stream that indicate when no more points in the stream will fall in specified regions. Our triangulators certify triangles or tetrahedra as Delaunay when the finalization tags show it is safe to do so. This make it possible to write them out early, freeing up memory to read more from the input stream. Because only the unfinalized parts of a triangulation are resident in memory, the memory footprint remains small. Videoplus leaflet:Martin Isenburg, Yuanxin Liu, Jonathan Shewchuk, and Jack Snoeyink,Illustrating the Streaming Construction of 2D Delaunay Triangulations, Proceedings of the Fifteenth Video Review of Computational Geometry(video) andProceedings of the Twenty-Second Annual Symposium on Computational Geometry (Sedona, Arizona), pages 481-482, Association for Computing Machinery, June 2006(leaflet). PDF (color, 766k, 2 pages). Thevideo(your choice of QT/mpeg4 or DivX) demonstrates the implementation described in our SIGGRAPH paper (above), and the leaflet describes the video.
Videoplus leaflet:Martin Isenburg, Yuanxin Liu, Jonathan Shewchuk, and Jack Snoeyink,Illustrating the Streaming Construction of 2D Delaunay Triangulations, Proceedings of the Fifteenth Video Review of Computational Geometry(video) andProceedings of the Twenty-Second Annual Symposium on Computational Geometry (Sedona, Arizona), pages 481-482, Association for Computing Machinery, June 2006(leaflet). PDF (color, 766k, 2 pages). Thevideo(your choice of QT/mpeg4 or DivX) demonstrates the implementation described in our SIGGRAPH paper (above), and the leaflet describes the video.- Talk slides: Streaming Construction of Delaunay Triangulations. PDF (color, 2,058k, 96 pages).
 Martin Isenburg, Yuanxin Liu, Jonathan Shewchuk, Jack Snoeyink, and Tim Thirion,Generating Raster DEM from Mass Points via TIN Streaming, Proceedings of the Fourth International Conference on Geographic Information Science (GIScience 2006, Münster, Germany), September 2006. PostScript (color, 16,554k, 13 pages). PDF (color, 490k, 13 pages).
Martin Isenburg, Yuanxin Liu, Jonathan Shewchuk, Jack Snoeyink, and Tim Thirion,Generating Raster DEM from Mass Points via TIN Streaming, Proceedings of the Fourth International Conference on Geographic Information Science (GIScience 2006, Münster, Germany), September 2006. PostScript (color, 16,554k, 13 pages). PDF (color, 490k, 13 pages). Martin Isenburg, Peter Lindstrom, Stefan Gumhold, and Jonathan Shewchuk,Streaming Compression of Tetrahedral Volume Meshes, Proceedings: Graphics Interface 2006 (Quebec City, Quebec, Canada), pages 115-121, June 2006. PDF (color, 3,821k, 7 pages).
Martin Isenburg, Peter Lindstrom, Stefan Gumhold, and Jonathan Shewchuk,Streaming Compression of Tetrahedral Volume Meshes, Proceedings: Graphics Interface 2006 (Quebec City, Quebec, Canada), pages 115-121, June 2006. PDF (color, 3,821k, 7 pages).
Finite Element Quality
IT IS NOT EASY TO FORMULATE the problem that a mesh generator is to solve. The natural first question is how to characterize good and bad triangles and tetrahedra based on their sizes and shapes. The answer to that question depends on the application. The universal concern is that the errors introduced by interpolation be as small as possible. In the finite element method, another concern (with different implications) is that the condition numbers of the stiffness matrices be small. Forty-odd years after the invention of the finite element method, our understanding of the relationship between mesh geometry, numerical accuracy, and stiffness matrix conditioning remains incomplete, especially in anisotropic cases. The following papers examine these issues for linear elements, and present error bounds and quality measures to help guide numerical analysts, researchers in triangulation and mesh generation, and application writers in graphics and geographic information systems.
 What Is a Good Linear Finite Element? Interpolation, Conditioning, Anisotropy, and Quality Measures, unpublished preprint, 2002. COMMENTS NEEDED! Help me improve this manuscript. If you read this, please send feedback. PostScript (5,336k, 66 pages), PDF (1,190k, 66 pages). Why are elements with tiny angles harmless for interpolation, but deadly for stiffness matrix conditioning? Why are long, thin elements with angles near 180o terrible in isotropic cases but perfectly acceptable, if they're aligned properly, for anisotropic PDEs whose solutions have anisotropic curvature? Why do elements that are too long and thin sometimes offer unexpectedly accurate PDE solutions? Why can interpolation error, discretization error, and stiffness matrix conditioning sometimes have a three-way disagreement about the aspect ratio and alignment of the ideal element? Why do scale-invariant element quality measures often lead to incorrect conclusions about how to improve a finite element mesh? Why is the popular inradius-to-circumradius ratio such an ineffective quality measure for optimization-based mesh smoothing? All is revealed here.
What Is a Good Linear Finite Element? Interpolation, Conditioning, Anisotropy, and Quality Measures, unpublished preprint, 2002. COMMENTS NEEDED! Help me improve this manuscript. If you read this, please send feedback. PostScript (5,336k, 66 pages), PDF (1,190k, 66 pages). Why are elements with tiny angles harmless for interpolation, but deadly for stiffness matrix conditioning? Why are long, thin elements with angles near 180o terrible in isotropic cases but perfectly acceptable, if they're aligned properly, for anisotropic PDEs whose solutions have anisotropic curvature? Why do elements that are too long and thin sometimes offer unexpectedly accurate PDE solutions? Why can interpolation error, discretization error, and stiffness matrix conditioning sometimes have a three-way disagreement about the aspect ratio and alignment of the ideal element? Why do scale-invariant element quality measures often lead to incorrect conclusions about how to improve a finite element mesh? Why is the popular inradius-to-circumradius ratio such an ineffective quality measure for optimization-based mesh smoothing? All is revealed here. What Is a Good Linear Element? Interpolation, Conditioning, and Quality Measures, Eleventh International Meshing Roundtable (Ithaca, New York), pages 115-126, Sandia National Laboratories, September 2002. PostScript (1,083k, 12 pages), PDF (250k, 12 pages). A greatly abbreviated version (omitting the anisotropic cases, the derivations, and the discussions of discretization error and time-dependent problems) of the manuscript above.
What Is a Good Linear Element? Interpolation, Conditioning, and Quality Measures, Eleventh International Meshing Roundtable (Ithaca, New York), pages 115-126, Sandia National Laboratories, September 2002. PostScript (1,083k, 12 pages), PDF (250k, 12 pages). A greatly abbreviated version (omitting the anisotropic cases, the derivations, and the discussions of discretization error and time-dependent problems) of the manuscript above.- Talk slides: What Is a Good Linear Finite Element? Interpolation, Conditioning, Anisotropy, and Quality Measures. PDF (color, 316k, 32 pages).An overview of the basic bounds on interpolation errors and maximum eigenvalues of element stiffness matrices, as well as the quality measures associated with them. Includes a brief discussion of how anisotropy affects these bounds and the shape of the ideal element.
Constrained Delaunay Triangulations
THE CONSTRAINED DELAUNAY TRIANGULATION (CDT) is a fundamental two-dimensional geometric structure with applications in interpolation, rendering, and mesh generation. Unfortunately, it has not hitherto been generalized to higher dimensions, and can never be fully generalized because not every polyhedron has a constrained tetrahedralization (allowing no additional vertices). Here, however, I prove that there is an easily tested condition that guarantees that a polyhedron (or piecewise linear domain) in three or more dimensions does have a constrained Delaunay triangulation. (A domain that satisfies the condition is said to be ridge-protected.)
Suppose you want to tetrahedralize a three-dimensional domain. The result implies that if you insert enough extra vertices on the boundary of a facet to recover its edges in a Delaunay tetrahedralization (in other words, if you make it be ridge-protected) then you can recover the facet's interior for free—that is, you can force the triangular faces of the tetrahedralization to conform to the facet without inserting yet more vertices. This method of facet recovery is immediately useful for mesh generation or the interpolation of discontinuous functions. (The result also fills a theoretical hole in my dissertation by showing that it is safe to delete a vertex from a constrained Delaunay tetrahedralization in the circumstances where my “diametral lens” algorithm does so.)
I provide two algorithms for constructing constrained Delaunay triangulations that are fast enough to be useful in practice. One is based on bistellar flips (which swap a few tetrahedra for a few others), and one is a sweep algorithm. The flip algorithm is easier to implement, and is probably usually faster in practice. However, the sweep algorithm works on almost every input that has a CDT, whereas the flip algorithm works only on ridge-protected inputs. The question of which algorithm is asymptotically faster is tricky—the answer depends on the size of the output, and is different for a worst-case input than for a random input; see the flip algorithm paper for details. See the “Strange Complexity” paper to find out why the sweep algorithm doesn't work on every input that has a CDT.
- Talk slides: Constrained Delaunay Tetrahedralizations, Bistellar Flips, and Provably Good Boundary Recovery. PDF (color, 233k, 49 pages).Slides from a talk that covers the CDT existence theorem, the vertex insertion algorithm for provably good boundary recovery, and the flip algorithm for inserting a facet into a CDT. You can evenwatch me give this talk at the Mathematical Sciences Research Institute.
 Constrained Delaunay Tetrahedralizations and Provably Good Boundary Recovery, Eleventh International Meshing Roundtable (Ithaca, New York), pages 193-204, Sandia National Laboratories, September 2002. PostScript (410k, 12 pages), PDF (187k, 12 pages). A basic guide: why constrained Delaunay tetrahedralizations are good for boundary recovery, and how to use them effectively. Includes an algorithm for inserting vertices to recover the segments of an input domain (e.g. a polyhedron), and high-level descriptions of two algorithms for constructing the constrained Delaunay tetrahedralization of the augmented domain. (Unfortunately, this paper predates the flip algorithm, which I think is usually a better choice in practice.) “Provably good boundary recovery” means that no edge of the final tetrahedralization is shorter than one quarter of the local feature size, so any subsequent Delaunay refinement will not be forced to create unnecessarily small elements. (An exception is any edge that spans two segments of the input domain separated by a small angle; a weaker bound applies to such an edge.) Aimed at programmers and practitioners. This paper discusses the three-dimensional case only, unlike most of the papers below.
Constrained Delaunay Tetrahedralizations and Provably Good Boundary Recovery, Eleventh International Meshing Roundtable (Ithaca, New York), pages 193-204, Sandia National Laboratories, September 2002. PostScript (410k, 12 pages), PDF (187k, 12 pages). A basic guide: why constrained Delaunay tetrahedralizations are good for boundary recovery, and how to use them effectively. Includes an algorithm for inserting vertices to recover the segments of an input domain (e.g. a polyhedron), and high-level descriptions of two algorithms for constructing the constrained Delaunay tetrahedralization of the augmented domain. (Unfortunately, this paper predates the flip algorithm, which I think is usually a better choice in practice.) “Provably good boundary recovery” means that no edge of the final tetrahedralization is shorter than one quarter of the local feature size, so any subsequent Delaunay refinement will not be forced to create unnecessarily small elements. (An exception is any edge that spans two segments of the input domain separated by a small angle; a weaker bound applies to such an edge.) Aimed at programmers and practitioners. This paper discusses the three-dimensional case only, unlike most of the papers below. General-Dimensional Constrained Delaunay and Constrained Regular Triangulations, I: Combinatorial Properties. Discrete & Computational Geometry 39(1-3):580-637, March 2008. PostScript (865k, 54 pages), PDF (447k, 54 pages). This manuscript lays down the combinatorial foundations of CDTs and weighted CDTs. It begins by proving that many properties of Delaunay triangulations (of any dimension) generalize to constrained Delaunay triangulations—in particular, the Delaunay Lemma, which states that a triangulation is a weighted CDT if and only if every (_d_-1)-dimensional face is either “locally regular” (locally convex on the lifting map) or a constraining face. Next, the manuscript shows that (weighted) CDTs have several optimality properties when used for piecewise linear interpolation. It culminates with the proof that if an input is weakly ridge-protected (a less restrictive condition than ridge-protected), it has a CDT. This proof also applies to weighted CDTs. This paper is the ideal starting point for researchers who want to work with CDTs of dimension higher than two, and it is the foundation of the correctness proofs of my CDT construction algorithms. For those who don't want to read the proofs, the introduction summarizes the results and how to use them. Aimed at computational geometers. Discusses the general-dimensional case.
General-Dimensional Constrained Delaunay and Constrained Regular Triangulations, I: Combinatorial Properties. Discrete & Computational Geometry 39(1-3):580-637, March 2008. PostScript (865k, 54 pages), PDF (447k, 54 pages). This manuscript lays down the combinatorial foundations of CDTs and weighted CDTs. It begins by proving that many properties of Delaunay triangulations (of any dimension) generalize to constrained Delaunay triangulations—in particular, the Delaunay Lemma, which states that a triangulation is a weighted CDT if and only if every (_d_-1)-dimensional face is either “locally regular” (locally convex on the lifting map) or a constraining face. Next, the manuscript shows that (weighted) CDTs have several optimality properties when used for piecewise linear interpolation. It culminates with the proof that if an input is weakly ridge-protected (a less restrictive condition than ridge-protected), it has a CDT. This proof also applies to weighted CDTs. This paper is the ideal starting point for researchers who want to work with CDTs of dimension higher than two, and it is the foundation of the correctness proofs of my CDT construction algorithms. For those who don't want to read the proofs, the introduction summarizes the results and how to use them. Aimed at computational geometers. Discusses the general-dimensional case. A Condition Guaranteeing the Existence of Higher-Dimensional Constrained Delaunay Triangulations, Proceedings of the Fourteenth Annual Symposium on Computational Geometry (Minneapolis, Minnesota), pages 76-85, Association for Computing Machinery, June 1998. PostScript (328k, 10 pages), PDF (181k, 10 pages). An early version of the CDT existence proof, which is the most difficult proof I've ever done. This version of the proof is shorter than the more general and rigorous proof in the paper above, but it also has some unnecessary complications that I excised from the later version. The proof is tough reading, but you don't need to understand it to use the result. Also includes a discussion of a slow-but-simple gift-wrapping algorithm for constructing a constrained Delaunay triangulation. This paper does not discuss weighted CDTs (constrained regular triangulations); see the paper above for that. Aimed at computational geometers. Discusses the general-dimensional case.
A Condition Guaranteeing the Existence of Higher-Dimensional Constrained Delaunay Triangulations, Proceedings of the Fourteenth Annual Symposium on Computational Geometry (Minneapolis, Minnesota), pages 76-85, Association for Computing Machinery, June 1998. PostScript (328k, 10 pages), PDF (181k, 10 pages). An early version of the CDT existence proof, which is the most difficult proof I've ever done. This version of the proof is shorter than the more general and rigorous proof in the paper above, but it also has some unnecessary complications that I excised from the later version. The proof is tough reading, but you don't need to understand it to use the result. Also includes a discussion of a slow-but-simple gift-wrapping algorithm for constructing a constrained Delaunay triangulation. This paper does not discuss weighted CDTs (constrained regular triangulations); see the paper above for that. Aimed at computational geometers. Discusses the general-dimensional case. Updating and Constructing Constrained Delaunay and Constrained Regular Triangulations by Flips, Proceedings of the Nineteenth Annual Symposium on Computational Geometry (San Diego, California), pages 181-190, Association for Computing Machinery, June 2003. PostScript (545k, 14 pages including a four-page appendix not in the published version), PDF (244k, 14 pages). If you want to incrementally update a constrained Delaunay triangulation, you need four operations: inserting and deleting a facet, and inserting and deleting a vertex. (To “insert a facet” is to force the faces of the CDT to respect the facet; to “delete a facet” is to relax the facet constraint so the CDT can act a little more Delaunay and a little less constrained.) This paper gives algorithms for the first two, based on simple bistellar flips. A sweep algorithm for deleting a vertex appears in the paper below (and it is trivially converted to a flip algorithm). Finally, Barry Joe's flip algorithm for inserting a vertex into a Delaunay triangulation is easily modified to work in a CDT. These operations work in any dimensionality, and they can all be applied to the more general class of_constrained regular triangulations_ (which include CDTs).
Updating and Constructing Constrained Delaunay and Constrained Regular Triangulations by Flips, Proceedings of the Nineteenth Annual Symposium on Computational Geometry (San Diego, California), pages 181-190, Association for Computing Machinery, June 2003. PostScript (545k, 14 pages including a four-page appendix not in the published version), PDF (244k, 14 pages). If you want to incrementally update a constrained Delaunay triangulation, you need four operations: inserting and deleting a facet, and inserting and deleting a vertex. (To “insert a facet” is to force the faces of the CDT to respect the facet; to “delete a facet” is to relax the facet constraint so the CDT can act a little more Delaunay and a little less constrained.) This paper gives algorithms for the first two, based on simple bistellar flips. A sweep algorithm for deleting a vertex appears in the paper below (and it is trivially converted to a flip algorithm). Finally, Barry Joe's flip algorithm for inserting a vertex into a Delaunay triangulation is easily modified to work in a CDT. These operations work in any dimensionality, and they can all be applied to the more general class of_constrained regular triangulations_ (which include CDTs).
By starting with a Delaunay (or regular) triangulation and incrementally inserting facets one by one, you can construct the constrained Delaunay (or constrained regular) triangulation of a ridge-protected input in O(nv + 1 log nv) time, where nv is the number of input vertices and d is the dimensionality. In odd dimensions (including three dimensions, which is what I care about most) this is within a factor of log nv of worst-case optimal. The algorithm is likely to take only O(nv log nv) time in many practical cases. Aimed at both programmers and computational geometers. Discusses the general-dimensional case, but most useful in three dimensions.
+ 1 log nv) time, where nv is the number of input vertices and d is the dimensionality. In odd dimensions (including three dimensions, which is what I care about most) this is within a factor of log nv of worst-case optimal. The algorithm is likely to take only O(nv log nv) time in many practical cases. Aimed at both programmers and computational geometers. Discusses the general-dimensional case, but most useful in three dimensions. Sweep Algorithms for Constructing Higher-Dimensional Constrained Delaunay Triangulations, Proceedings of the Sixteenth Annual Symposium on Computational Geometry (Hong Kong), pages 350-359, Association for Computing Machinery, June 2000. PostScript (352k, 10 pages), PDF (195k, 10 pages). Gives an O(nvns)-time sweep algorithm for constructing a constrained Delaunay triangulation, where nv is the number of input vertices, and ns is the number of simplices in the triangulation. (The algorithm is likely to be faster in most practical cases.) The running time improves to O(ns log nv) for star-shaped polytopes, yielding an efficient way to delete a vertex from a CDT.
Sweep Algorithms for Constructing Higher-Dimensional Constrained Delaunay Triangulations, Proceedings of the Sixteenth Annual Symposium on Computational Geometry (Hong Kong), pages 350-359, Association for Computing Machinery, June 2000. PostScript (352k, 10 pages), PDF (195k, 10 pages). Gives an O(nvns)-time sweep algorithm for constructing a constrained Delaunay triangulation, where nv is the number of input vertices, and ns is the number of simplices in the triangulation. (The algorithm is likely to be faster in most practical cases.) The running time improves to O(ns log nv) for star-shaped polytopes, yielding an efficient way to delete a vertex from a CDT. Nicolas Grislain and Jonathan Richard Shewchuk,The Strange Complexity of Constrained Delaunay Triangulation, Proceedings of the Fifteenth Canadian Conference on Computational Geometry (Halifax, Nova Scotia), pages 89-93, August 2003. PostScript (195k, 4 pages), PDF (73k, 4 pages). The problem of constructing a constrained Delaunay tetrahedralization has the unusual status (for a small-dimensional problem) of being NP-hard only for degenerate inputs, namely those with subsets of five or more cospherical vertices. This paper proves one half of that statement: it is NP-hard to decide whether a polyhedron has a constrained Delaunay tetrahedralization. The paper on sweep algorithms (above) contains the proof of the other half: for a polyhedron (or more generally, a piecewise linear complex) with no five vertices lying on a common sphere, a polynomial-time algorithm constructs the CDT (if it exists) and thereby solves the decision problem. Freaky, eh?
Nicolas Grislain and Jonathan Richard Shewchuk,The Strange Complexity of Constrained Delaunay Triangulation, Proceedings of the Fifteenth Canadian Conference on Computational Geometry (Halifax, Nova Scotia), pages 89-93, August 2003. PostScript (195k, 4 pages), PDF (73k, 4 pages). The problem of constructing a constrained Delaunay tetrahedralization has the unusual status (for a small-dimensional problem) of being NP-hard only for degenerate inputs, namely those with subsets of five or more cospherical vertices. This paper proves one half of that statement: it is NP-hard to decide whether a polyhedron has a constrained Delaunay tetrahedralization. The paper on sweep algorithms (above) contains the proof of the other half: for a polyhedron (or more generally, a piecewise linear complex) with no five vertices lying on a common sphere, a polynomial-time algorithm constructs the CDT (if it exists) and thereby solves the decision problem. Freaky, eh? Stabbing Delaunay Tetrahedralizations, Discrete & Computational Geometry 32(3):339-343, October 2004. PostScript (143k, 4 pages), PDF (70k, 4 pages), HTML. This note answers (pessimistically) the formerly open question of how many tetrahedra in an _n_-point Delaunay tetrahedralization can be stabbed by a straight line. The answer: for a worst-case tetrahedralization, a line can intersect the interiors of
Stabbing Delaunay Tetrahedralizations, Discrete & Computational Geometry 32(3):339-343, October 2004. PostScript (143k, 4 pages), PDF (70k, 4 pages), HTML. This note answers (pessimistically) the formerly open question of how many tetrahedra in an _n_-point Delaunay tetrahedralization can be stabbed by a straight line. The answer: for a worst-case tetrahedralization, a line can intersect the interiors of tetrahedra. In d dimensions, a line can stab the interiors of
tetrahedra. In d dimensions, a line can stab the interiors of Delaunay _d_-simplices. The result explains why my sweep algorithm for constructing CDTs has a worst-case running time of O(nvns) and not O(_nv_2 +ns log nv). The difficulty of finding the worst-case example explains why the sweep algorithm is unlikely to take longer than O(_nv_2 +ns log nv) time on any real-world input.
Delaunay _d_-simplices. The result explains why my sweep algorithm for constructing CDTs has a worst-case running time of O(nvns) and not O(_nv_2 +ns log nv). The difficulty of finding the worst-case example explains why the sweep algorithm is unlikely to take longer than O(_nv_2 +ns log nv) time on any real-world input.
Surface Reconstruction
WE WISH TO RECONSTRUCT CLOSED SURFACESfrom simple geometric inputs like sets of points, sampled from the surface of a three-dimensional object using a laser range finder, or sets of polygons, which are often used as crude geometric models for rendering. However, the input data are rarely well-behaved. Laser range finders are imperfect physical devices that introduce random errors (noise) into the point coordinates, and often introduce points that don't lie anywhere near the surface (outliers) as well. Moreover, range finders can't usually scan an entire object's surface—portions of the surface are left undersampled or unsampled. Polygon inputs used for rendering are rarely topologically consistent—the polygons often have spurious intersections or leave small gaps in what should be a closed surface. For either type of input, we wish to generate a watertight surface that approximates the surface suggested by the data.
 Ravikrishna Kolluri, Jonathan Richard Shewchuk, and James F. O'Brien,Spectral Surface Reconstruction from Noisy Point Clouds, Symposium on Geometry Processing 2004 (Nice, France), pages 11-21, Eurographics Association, July 2004. PDF (color, 7,648k, 11 pages). Researchers have put forth several provably good Delaunay-based algorithms for surface reconstruction from unorganized point sets. However, in the presence of undersampling, noise, and outliers, they are neither “provably good” nor robust in practice. Our Eigencrust algorithm uses a spectral graph partitioner to make robust decisions about which Delaunay tetrahedra are inside the surface and which are outside. In practice, the Eigencrust algorithm handles undersampling, noise, and outliers quite well, while giving essentially the same results as the provably good Tight Cocone or Powercrust algorithms on “clean” point sets. (There is no theory in this paper, though.)
Ravikrishna Kolluri, Jonathan Richard Shewchuk, and James F. O'Brien,Spectral Surface Reconstruction from Noisy Point Clouds, Symposium on Geometry Processing 2004 (Nice, France), pages 11-21, Eurographics Association, July 2004. PDF (color, 7,648k, 11 pages). Researchers have put forth several provably good Delaunay-based algorithms for surface reconstruction from unorganized point sets. However, in the presence of undersampling, noise, and outliers, they are neither “provably good” nor robust in practice. Our Eigencrust algorithm uses a spectral graph partitioner to make robust decisions about which Delaunay tetrahedra are inside the surface and which are outside. In practice, the Eigencrust algorithm handles undersampling, noise, and outliers quite well, while giving essentially the same results as the provably good Tight Cocone or Powercrust algorithms on “clean” point sets. (There is no theory in this paper, though.)- Talk slides: Spectral Surface Reconstruction from Noisy Point Clouds. PDF (color, 1,758k, 53 pages). Slides from a talk on our paper above.
 Chen Shen, James F. O'Brien, and Jonathan R. Shewchuk,Interpolating and Approximating Implicit Surfaces from Polygon Soup, ACM Transactions on Graphics 23(3):896-904, August 2004. Special issue on Proceedings of SIGGRAPH 2004. PDF (color, 17,269k, 9 pages). The Moving Least Squares (MLS) method is a popular way to define an implicit surface that interpolates or approximates a set of points in three-dimensional space. But graphics programmers have made millions of polygonalized surface models; what if we want to interpolate whole polygons? Approximating a polygon as a bunch of points gives poor results. Instead, we show how to force an MLS function to have a specified value over each input polygon, by integrating constraints over triangles. Better yet, we show how to force the MLS function to have a specified gradient over each polygon as well, so that we can robustly specify which parts of space should be inside or outside the implicit surface—without creating undue oscillations in the MLS function. The trick is to define a different function on each input polygon, and use MLS to interpolate between _functions_—not just to interpolate between values (as the usual formulations of MLS for implicit surfaces do). This trick gives us profound control of an MLS function. Although our examples are all surfaces embedded in three-dimensional space, the techniques generalize to any dimensionality.
Chen Shen, James F. O'Brien, and Jonathan R. Shewchuk,Interpolating and Approximating Implicit Surfaces from Polygon Soup, ACM Transactions on Graphics 23(3):896-904, August 2004. Special issue on Proceedings of SIGGRAPH 2004. PDF (color, 17,269k, 9 pages). The Moving Least Squares (MLS) method is a popular way to define an implicit surface that interpolates or approximates a set of points in three-dimensional space. But graphics programmers have made millions of polygonalized surface models; what if we want to interpolate whole polygons? Approximating a polygon as a bunch of points gives poor results. Instead, we show how to force an MLS function to have a specified value over each input polygon, by integrating constraints over triangles. Better yet, we show how to force the MLS function to have a specified gradient over each polygon as well, so that we can robustly specify which parts of space should be inside or outside the implicit surface—without creating undue oscillations in the MLS function. The trick is to define a different function on each input polygon, and use MLS to interpolate between _functions_—not just to interpolate between values (as the usual formulations of MLS for implicit surfaces do). This trick gives us profound control of an MLS function. Although our examples are all surfaces embedded in three-dimensional space, the techniques generalize to any dimensionality.
Geometric Robustness
 GEOMETRIC PROGRAMS ARE SURPRISINGLY SUSCEPTIBLEto failure because of floating-point roundoff error. Robustness problems can be solved by using exact arithmetic, at the cost of reducing program speed by a factor of ten or more. Here, I describe a strategy for computing correct answers quickly when the inputs are floating-point values. (Much other research has dealt with the problem for integer inputs, which are less convenient for users but more tractable for robustness researchers.)
GEOMETRIC PROGRAMS ARE SURPRISINGLY SUSCEPTIBLEto failure because of floating-point roundoff error. Robustness problems can be solved by using exact arithmetic, at the cost of reducing program speed by a factor of ten or more. Here, I describe a strategy for computing correct answers quickly when the inputs are floating-point values. (Much other research has dealt with the problem for integer inputs, which are less convenient for users but more tractable for robustness researchers.)
To make robust geometric tests fast, I propose two new techniques (which can also be applied to other problems of numerical accuracy). First, I develop and prove the correctness of software-level algorithms for arbitrary precision floating-point arithmetic. These algorithms are refinements (especially with regard to speed) of algorithms suggested by Douglas Priest, and are roughly five times faster than the best available competing method when values of small or intermediate precision (hundreds or thousands of bits) are used. Second, I show how simple expressions (whose only operations are addition, subtraction, and multiplication) can be computed adaptively, trading off accuracy and speed as necessary to satisfy an error bound as quickly as possible. (This technique is probably applicable to any exact arithmetic scheme.) I apply these ideas to build fast, correct_orientation_ and incircle tests in two and three dimensions, and to make robust the implementations of two- and three-dimensional Delaunay triangulation in Triangle and Pyramid. Detailed measurements show that in most circumstances, these programs run nearly as quickly when using my adaptive predicates as they do using nonrobust predicates.
See my Robust Predicates page for more information about this research, or to obtain C source code for exact floating-point addition and multiplication and the robust geometric predicates.
 Adaptive Precision Floating-Point Arithmetic and Fast Robust Geometric Predicates, Discrete & Computational Geometry 18(3):305-363, October 1997. PostScript (775k, 55 pages), PDF (556k, 55 pages). Also appears as Chapter 6 of my dissertation.
Adaptive Precision Floating-Point Arithmetic and Fast Robust Geometric Predicates, Discrete & Computational Geometry 18(3):305-363, October 1997. PostScript (775k, 55 pages), PDF (556k, 55 pages). Also appears as Chapter 6 of my dissertation. Robust Adaptive Floating-Point Geometric Predicates, Proceedings of the Twelfth Annual Symposium on Computational Geometry (Philadelphia, Pennsylvania), pages 141-150, Association for Computing Machinery, May 1996. Abstract (with BibTeX citation), PostScript (310k, 10 pages), PDF (174k, 10 pages). A very abbreviated summary of the ideas from the full-length paper above.
Robust Adaptive Floating-Point Geometric Predicates, Proceedings of the Twelfth Annual Symposium on Computational Geometry (Philadelphia, Pennsylvania), pages 141-150, Association for Computing Machinery, May 1996. Abstract (with BibTeX citation), PostScript (310k, 10 pages), PDF (174k, 10 pages). A very abbreviated summary of the ideas from the full-length paper above.
The Quake Project
 PAPERS ABOUT THE QUAKE PROJECT, a multidisciplinary Grand Challenge Application Group studying ground motion in large basins during strong earthquakes, with the goal of characterizing the seismic response of the Los Angeles basin. The Quake Project is a joint effort between the departments of Computer Science and Civil and Environmental Engineering at Carnegie Mellon, the Southern California Earthquake Center, and the National University of Mexico. We've created some of the largest unstructured finite element simulations ever carried out; in particular, the papers below describe a simulation of ground motion during an aftershock of the 1994 Northridge Earthquake.
PAPERS ABOUT THE QUAKE PROJECT, a multidisciplinary Grand Challenge Application Group studying ground motion in large basins during strong earthquakes, with the goal of characterizing the seismic response of the Los Angeles basin. The Quake Project is a joint effort between the departments of Computer Science and Civil and Environmental Engineering at Carnegie Mellon, the Southern California Earthquake Center, and the National University of Mexico. We've created some of the largest unstructured finite element simulations ever carried out; in particular, the papers below describe a simulation of ground motion during an aftershock of the 1994 Northridge Earthquake.
 Hesheng Bao, Jacobo Bielak, Omar Ghattas, Loukas F. Kallivokas, David R. O'Hallaron, Jonathan R. Shewchuk, and Jifeng Xu,Large-scale Simulation of Elastic Wave Propagation in Heterogeneous Media on Parallel Computers, Computer Methods in Applied Mechanics and Engineering 152(1-2):85-102, 22 January 1998. Abstract (with BibTex citation), Compressed PostScript (color, 4,262k, 34 pages)(Expands to 41,267k when uncompressed), PDF (color, 6,681k, 34 pages), HTML. The PostScript version uncompresses into six PostScript files. The first and last of these files are black-and-white. The middle four are huge and in full color.
Hesheng Bao, Jacobo Bielak, Omar Ghattas, Loukas F. Kallivokas, David R. O'Hallaron, Jonathan R. Shewchuk, and Jifeng Xu,Large-scale Simulation of Elastic Wave Propagation in Heterogeneous Media on Parallel Computers, Computer Methods in Applied Mechanics and Engineering 152(1-2):85-102, 22 January 1998. Abstract (with BibTex citation), Compressed PostScript (color, 4,262k, 34 pages)(Expands to 41,267k when uncompressed), PDF (color, 6,681k, 34 pages), HTML. The PostScript version uncompresses into six PostScript files. The first and last of these files are black-and-white. The middle four are huge and in full color. Hesheng Bao, Jacobo Bielak, Omar Ghattas, David R. O'Hallaron, Loukas F. Kallivokas, Jonathan R. Shewchuk, and Jifeng Xu,Earthquake Ground Motion Modeling on Parallel Computers, Supercomputing '96 (Pittsburgh, Pennsylvania), November 1996. Abstract (with BibTeX citation), PostScript (color, 9,370k, 19 pages), PDF (color, 2,436k, 19 pages), HTML. An abbreviated version of the full-length paper above.
Hesheng Bao, Jacobo Bielak, Omar Ghattas, David R. O'Hallaron, Loukas F. Kallivokas, Jonathan R. Shewchuk, and Jifeng Xu,Earthquake Ground Motion Modeling on Parallel Computers, Supercomputing '96 (Pittsburgh, Pennsylvania), November 1996. Abstract (with BibTeX citation), PostScript (color, 9,370k, 19 pages), PDF (color, 2,436k, 19 pages), HTML. An abbreviated version of the full-length paper above.
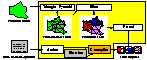 OUR SECRET TO PRODUCING such huge unstructured simulations? With the collaboration of David O'Hallaron, I've writtenArchimedes, a chain of tools for automating the construction of general-purpose finite element simulations on parallel computers. In addition to the mesh generators Triangle and Pyramid discussed above, Archimedes includes Slice, a mesh partitioner based on geometric recursive bisection;Parcel, which performs the surprisingly jumbled task of computing communication schedules and reordering partitioned mesh data into a format a parallel simulation can use; and Author, which generates parallel C code from high-level machine-independent programs (which are currently written by the civil engineers in our group). Archimedes has made it possible for the Quake Project to weather four consecutive changes in parallel architecture without missing a beat. The most recent information about Archimedes is contained in the Quake papers listed above. See also theArchimedes page.
OUR SECRET TO PRODUCING such huge unstructured simulations? With the collaboration of David O'Hallaron, I've writtenArchimedes, a chain of tools for automating the construction of general-purpose finite element simulations on parallel computers. In addition to the mesh generators Triangle and Pyramid discussed above, Archimedes includes Slice, a mesh partitioner based on geometric recursive bisection;Parcel, which performs the surprisingly jumbled task of computing communication schedules and reordering partitioned mesh data into a format a parallel simulation can use; and Author, which generates parallel C code from high-level machine-independent programs (which are currently written by the civil engineers in our group). Archimedes has made it possible for the Quake Project to weather four consecutive changes in parallel architecture without missing a beat. The most recent information about Archimedes is contained in the Quake papers listed above. See also theArchimedes page.  Anja Feldmann, Omar Ghattas, John R. Gilbert, Gary L. Miller, David R. O'Hallaron, Eric J. Schwabe, Jonathan R. Shewchuk, and Shang-Hua Teng,Automated Parallel Solution of Unstructured PDE Problems, unpublished manuscript, June 1996. PostScript (b/w, 1,708k, 19 pages), PostScript (color, 1,845k, 19 pages). Because color printing is expensive, you may want to print a complete black and white copy; then use a color printer to print the following file (which contains only the five color pages) and replace the corresponding black and white pages. PostScript (color pages only, 818k, 5 pages). A simple overview aimed at people who have little familiarity with finite element methods or parallel scientific computing.
Anja Feldmann, Omar Ghattas, John R. Gilbert, Gary L. Miller, David R. O'Hallaron, Eric J. Schwabe, Jonathan R. Shewchuk, and Shang-Hua Teng,Automated Parallel Solution of Unstructured PDE Problems, unpublished manuscript, June 1996. PostScript (b/w, 1,708k, 19 pages), PostScript (color, 1,845k, 19 pages). Because color printing is expensive, you may want to print a complete black and white copy; then use a color printer to print the following file (which contains only the five color pages) and replace the corresponding black and white pages. PostScript (color pages only, 818k, 5 pages). A simple overview aimed at people who have little familiarity with finite element methods or parallel scientific computing. Jonathan Richard Shewchuk and Omar Ghattas,A Compiler for Parallel Finite Element Methods with Domain-Decomposed Unstructured Meshes, Proceedings of the Seventh International Conference on Domain Decomposition Methods in Scientific and Engineering Computing (Pennsylvania State University), Contemporary Mathematics 180 (David E. Keyes and Jinchao Xu, editors), pages 445-450, American Mathematical Society, October 1993. Abstract, PostScript (color, 1,203k, 6 pages), PDF (color, 319k, 6 pages). A short discussion of our unusual way of storing distributed stiffness matrices and how it makes some domain decomposition algorithms easy to parallelize.
Jonathan Richard Shewchuk and Omar Ghattas,A Compiler for Parallel Finite Element Methods with Domain-Decomposed Unstructured Meshes, Proceedings of the Seventh International Conference on Domain Decomposition Methods in Scientific and Engineering Computing (Pennsylvania State University), Contemporary Mathematics 180 (David E. Keyes and Jinchao Xu, editors), pages 445-450, American Mathematical Society, October 1993. Abstract, PostScript (color, 1,203k, 6 pages), PDF (color, 319k, 6 pages). A short discussion of our unusual way of storing distributed stiffness matrices and how it makes some domain decomposition algorithms easy to parallelize. Eric J. Schwabe, Guy E. Blelloch, Anja Feldmann, Omar Ghattas, John R. Gilbert, Gary L. Miller, David R. O'Hallaron, Jonathan R. Shewchuk, and Shang-Hua Teng,A Separator-Based Framework for Automated Partitioning and Mapping of Parallel Algorithms for Numerical Solution of PDEs, Proceedings of the 1992 DAGS/PC Symposium, Dartmouth Institute for Advanced Graduate Studies, pages 48-62, June 1992. Abstract, PostScript (2,247k, 15 pages), PDF (464k, 15 pages). This paper is made obsolete by the manuscript “Automated Parallel Solution of Unstructured PDE Problems” above.
SEVERAL PAPERS ON THE COMPUTATION AND COMMUNICATION DEMANDSof the Quake Project's parallel finite element simulations, and what requirements such unstructured simulations will place on future parallel machines and networks. For designers of computer architecture who want to better understand unstructured applications.
Eric J. Schwabe, Guy E. Blelloch, Anja Feldmann, Omar Ghattas, John R. Gilbert, Gary L. Miller, David R. O'Hallaron, Jonathan R. Shewchuk, and Shang-Hua Teng,A Separator-Based Framework for Automated Partitioning and Mapping of Parallel Algorithms for Numerical Solution of PDEs, Proceedings of the 1992 DAGS/PC Symposium, Dartmouth Institute for Advanced Graduate Studies, pages 48-62, June 1992. Abstract, PostScript (2,247k, 15 pages), PDF (464k, 15 pages). This paper is made obsolete by the manuscript “Automated Parallel Solution of Unstructured PDE Problems” above.
SEVERAL PAPERS ON THE COMPUTATION AND COMMUNICATION DEMANDSof the Quake Project's parallel finite element simulations, and what requirements such unstructured simulations will place on future parallel machines and networks. For designers of computer architecture who want to better understand unstructured applications.  David O'Hallaron, Jonathan Richard Shewchuk, and Thomas Gross,Architectural Implications of a Family of Irregular Applications, Fourth International Symposium on High Performance Computer Architecture (Las Vegas, Nevada), February 1998. Abstract (with BibTex citation), PostScript (1,289k, 20 pages), PDF (218k, 20 pages).
David O'Hallaron, Jonathan Richard Shewchuk, and Thomas Gross,Architectural Implications of a Family of Irregular Applications, Fourth International Symposium on High Performance Computer Architecture (Las Vegas, Nevada), February 1998. Abstract (with BibTex citation), PostScript (1,289k, 20 pages), PDF (218k, 20 pages). David R. O'Hallaron and Jonathan Richard Shewchuk,Properties of a Family of Parallel Finite Element Simulations, Technical Report CMU-CS-96-141, School of Computer Science, Carnegie Mellon University, Pittsburgh, Pennsylvania, December 1996. Abstract (with BibTex citation), PostScript (987k, 22 pages).
David R. O'Hallaron and Jonathan Richard Shewchuk,Properties of a Family of Parallel Finite Element Simulations, Technical Report CMU-CS-96-141, School of Computer Science, Carnegie Mellon University, Pittsburgh, Pennsylvania, December 1996. Abstract (with BibTex citation), PostScript (987k, 22 pages).
Route Planning
HERE, WE APPLY CONFORMING DELAUNAY TRIANGULATIONSto the AI problem of route planning on real-world maps. The problem is inherently “dirty,” with difficulties ranging from one-way streets to incorrect maps, so it is not straightforward even to formally specify a problem to solve. Our approach is to use an analogical planner, which uses past experiences to help choose the best result for future travel. However, this case-based reasoning approach to planning requires a similarity metric to decide which previous cases are most appropriate for the current problem. Our similarity metric begins by describing a geometric problem that roughly approximates the problem of discovering good previous cases, then we solve the geometric problem with a combination of geometric theory and heuristics. The solution of the geometric problem is then cast into an approximate solution to the planning problem, and the rough edges are smoothed by brute-force symbolic planning. This procedure proves to be faster than brute-force symbolic planning from scratch.
 Karen Zita Haigh, Jonathan Richard Shewchuk, and Manuela M. Veloso,Exploiting Domain Geometry in Analogical Route Planning, Journal of Experimental and Theoretical Artificial Intelligence9(4):509-541, October 1997. Abstract, PostScript (1,419k, 30 pages), PDF (688k, 30 pages).
Karen Zita Haigh, Jonathan Richard Shewchuk, and Manuela M. Veloso,Exploiting Domain Geometry in Analogical Route Planning, Journal of Experimental and Theoretical Artificial Intelligence9(4):509-541, October 1997. Abstract, PostScript (1,419k, 30 pages), PDF (688k, 30 pages). Karen Zita Haigh, Jonathan Richard Shewchuk, and Manuela M. Veloso,Route Planning and Learning from Execution, Working notes from the AAAI Fall Symposium “Planning and Learning: On to Real Applications” (New Orleans, Louisiana), pages 58-64, AAAI Press, November 1994. Abstract, PostScript (163k, 7 pages), PDF (106k, 7 pages). Superseded by the first paper in this list.
Karen Zita Haigh, Jonathan Richard Shewchuk, and Manuela M. Veloso,Route Planning and Learning from Execution, Working notes from the AAAI Fall Symposium “Planning and Learning: On to Real Applications” (New Orleans, Louisiana), pages 58-64, AAAI Press, November 1994. Abstract, PostScript (163k, 7 pages), PDF (106k, 7 pages). Superseded by the first paper in this list. Karen Zita Haigh and Jonathan Richard Shewchuk,Geometric Similarity Metrics for Case-Based Reasoning, Case-Based Reasoning: Working Notes from the AAAI-94 Workshop (Seattle, Washington), pages 182-187, AAAI Press, August 1994. Abstract, PostScript (156k, 6 pages). Superseded by the first paper in this list.
Karen Zita Haigh and Jonathan Richard Shewchuk,Geometric Similarity Metrics for Case-Based Reasoning, Case-Based Reasoning: Working Notes from the AAAI-94 Workshop (Seattle, Washington), pages 182-187, AAAI Press, August 1994. Abstract, PostScript (156k, 6 pages). Superseded by the first paper in this list.

