Adaptable boronate ester hydrogels with tunable viscoelastic spectra to probe timescale dependent mechanotransduction (original) (raw)
. Author manuscript; available in PMC: 2020 Dec 1.
Abstract
Cells are capable of sensing the differences in elastic and viscous properties (i.e., the ‘viscoelasticity’) of their tissue microenvironment and responding accordingly by changing their transcriptional activity and modifying their behaviors. When designing viscoelastic materials to mimic the mechanical properties of native tissue niches, it is important to consider the timescales over which cells probe their microenvironment, as the response of a viscoelastic material to an imposed stress or strain is timescale dependent. Although the timescale of cellular mechano-sensing is currently unknown, hydrogel substrates with tunable viscoelastic spectra can allow one to probe the cellular response to timescale dependent mechanical properties. Here, we report on a cytocompatible and viscoelastic hydrogel culture system with reversible boronate ester cross-links, formed from pendant boronic acid and vicinal diol moieties, where the equilibrium kinetics of esterification were leveraged to tune the viscoelastic spectrum. We found that viscoelasticity increased as a function of the boronic acid and vicinal diol concentration, and also increased with decreasing cross-linker concentration, where the maximal loss tangent achieved with this system was 0.55 at 0.1 rad s−1. Additionally, we found that the _cis_-vicinal diols configuration altered the viscoelastic spectra, where a tan δ peak occurred at ~1 rad s−1 for hydrogels functionalized with boronic acid, while an additional peak formed at ≥10 rad s−1 for hydrogels functionalized with both boronic acid and _cis-vic_-diols. In experiments with NIH-3T3 fibroblasts cultured on these hydrogels, the projected cell area and nuclear area, focal adhesion tension, and subcellular localization of YAP/TAZ were all found to be lower for cells cultured on the viscoelastic hydrogels compared to elastic hydrogels with a similar storage modulus. Despite these differences, there was not a statistically significant relationship between the frequency dependent viscoelastic material properties characterized in this study and cellular morphologies, focal adhesion tension, or the subcellular localization of YAP. While these results demonstrate that mechanotransduction pathways are affected by viscoelasticity, they also suggest that these mechanotransduction pathways are not particularly sensitive to the frequency dependent viscoelastic material properties from 0.1 to 10 rad s−1.
Keywords: hydrogel, viscoelastic, mechanotransduction, boronate esterification
1. Introduction
Focal adhesions (FAs) are a complex of transmembrane proteins that allow for cellular attachment to adhesion motifs in the extracellular domain, and consequently, connect the cellular microenvironment directly to the actin cytoskeleton. FAs allow biophysical signals from the microenvironment to be translocated across the cell membrane where they regulate chemical signaling cascades, in a process called mechanotransduction. The transduction of biophysical signals into chemical signals, together with direct modification of nuclear processes via the LINC complex, help to maintain normal physiological function at the transcriptional level [1–3]. Elucidating the biophysical signals that are the most pertinent in controlling cellular transcription will provide important design rules for therapeutic biomaterials that actively drive functional tissue generation.
Synthetic hydrogels have found use as substrates for studying cellular mechanotransduction, and the resulting cellular fate decisions, because the elastic modulus is easily controlled by tailoring the cross-linking density. As an example, Engler et al. cultured human mesenchymal stem cells (hMSCs) on synthetic hydrogels with elastic moduli similar to those of native tissues and found that hMSC lineage specification corresponded to the elastic modulus of the tissue from which the lineage was derived [4]. However, most tissues are viscoelastic in nature, that is, their relationship between stress and strain are time or frequency dependent, and accordingly, there has been a recent interest in using hydrogels to mimic the viscoelastic properties of the cellular microenvironment in order to better understand how these additional biophysical signals play a role in mechanotransduction [5,6]. Viscoelasticity is a physical property that defines the time dependence of the elastic and viscous characteristics of materials. Viscoelastic materials undergo two important transitions in state depending on whether a constant stress is applied (viscoelastic creep), or a constant strain (viscoelastic relaxation). Viscoelastic creep is a process in which material deformation (i.e., strain ε ) under an applied (constant) stress (σ ) is hindered due to viscous retardation, where the transient creep compliance (J = ε / σ ) is intrinsically linked to the frequency spectra of the dynamic storage (G’ ) and loss (G” ) moduli, which characterize the amount of energy that is stored or dissipated by the material, respectively [7,8]. For cross-linked polymers, the rate of change of J at a given retardation time (τ ) is proportional to the magnitude of the dynamic loss tangent (tan δ), which is defined as the ratio of G” to G’, at an angular frequency of ω = τ −1 [ 8 ]. Accordingly, the timescales at which J changes most rapidly is inversely proportional to the ω at which tan δ is maximized [7,8]. Consequently, both the timescales (e.g., frequencies) and the magnitudes of viscoelastic processes are likely to be an important component of the biophysical signals that direct tissue-specific cell function through mechanotransduction [5,9–11].
Indeed, Cameron et al. found that in the absence of induction factors, hMSCs cultured on highly creeping hydrogels with a large G” spontaneously expressed markers of the smooth muscle cell (SMC) lineage, and in the presence of induction factors, hMSCs cultured on high-creep hydrogels exhibited greater multipotent differentiation potential compared to hMSCs on low-creep hydrogels with an equivalent G’ but smaller G” [12]. They further demonstrated that on high-creep hydrogels, hMSCs compensate for decreased passive cytoskeletal tension by spreading via activation of Rac1, which is a mechanotransductive Rho-GTPase that also plays a role in SMC differentiation [13]. Similarly, it has been shown that hMSCs encapsulated in hydrogels characterized by short-timescale viscoplastic processes (i.e., irreversible deformation) were predominantly committed to the osteogenic lineage; conversely, hMSCs encapsulated in hydrogels characterized by long-timescale viscoplastic processes were predominantly committed to the adipogenic lineage [5]. Despite a newfound interest in viscoelastic biophysical signaling, to our knowledge, there have not been any studies that investigate the relevant frequencies at which cells probe and sense viscoelastic properties.
The idea that cells may sense mechanical properties at specific frequencies is supported by observations that mouse embryonic fibroblasts periodically “tug” on polyacrylamide hydrogel substrates with their FAs at a period of approximately 10 s (0.63 rad s−1) [14]. However, the positional sampling frequency of periodic “tugging” in this study was quite low due to experimental limitations, and thus, cells may sample substrate mechanics at much shorter timescales. In fact, the motor-clutch model of cellular traction developed by Chan and Odde predicts that FAs characterized by a short ensemble clutch binding time (e.g., a small number of clutches or a rapid clutch binding rate) coupled to stiff substrata, will exhibit “load-and-fail” behavior — a regime characterized by maximal traction force transmission and sensitivity to substrate mechanics — at cycle times significantly shorter than 10 s [15–17]. Interestingly, Charrier et al. discovered that NIH-3T3 morphology, stress fiber formation, and paxillin patch formation were more sensitive to substrate viscoelasticity when adhered to a hydrogel functionalized with collagen I compared to fibronectin, which they attributed to differential frequencies of probing by the corresponding integrin receptors [10].
Here, we describe the development and characterization of biocompatible hydrogels with tunable viscoelastic spectra to ascertain the physiologically relevant timescales at which cells sense biophysical signals. The esterification of boronic acid and vicinal diols was exploited to enable viscoelasticity via covalent adaptable hydrogel cross-links. Boronate esterification was employed as an adaptable cross-linking chemistry because the reaction is cytocompatible and affords controllable kinetics [18–24]. A complex series of equilibria govern boronate ester stability and has only been elucidated recently, despite a long history. Specifically, the boronate ester equilibrium is affected by steric interactions produced in the adduct relative to the free state, as well as the pH, diol pKa, and boronic acid pKa [21,25,26]. In contrast to other viscoelastic hydrogels based upon boronate esterification [19], the boronate esters in this system are formed with simple monosaccharides such that the esterification equilibrium — and consequently, the network adaptability — is predominantly affected by ring strain in the boronate ester resulting from vicinal hydroxyl configuration in the saccharide [21,25]. For monosaccharides in the pyranose form, equatorial-axial vicinal diol configurations (i.e., _cis_-_vic-_diol) exhibit the greatest propensity for boronate ester formation (10 – 20 M−1), followed by cis/_trans_-4,6-diols (3 – 6 M−1), and equatorial-equatorial vicinal diols (i.e., _trans_-_vic_-diol, 0 – 1 M−1) [21,25,27]. Hydrogel viscoelasticity was first characterized as a function of boronic acid concentration and _vic_-diol configuration. Next, we examined the effect of _vic_-diol and cross-link concentration on the time-variant network, properties. Finally, experiments were conducted to determine the angular frequency that has the greatest effect on cellular morphology, focal adhesion tension, and transcriptional co-activator sub-cellular localization. The results presented confirm that the hydrogel system described herein provides a unique cellular substrate with tunable viscoelastic spectra that affords frequency-specific targeting of mechanotransduction pathways.
2. Experimental section
2.1. Materials
Poly(ethylene glycol)-diacrylate (2kDa, PEG-diAc) was purchased from JenKem Technology USA. The cell adhesion peptide (RGD-SH) was purchased from the American Peptide Company. Methyl-α-D-glucopyranoside, methyl-α-D-mannopyranoside, vinyl acrylate, 4-methoxyphenol (MEHQ), anhydrous acetonitrile, methanol, Candida Antarctica lipase B acrylic resin (Novozym 435, 5,000 U g−1), 4′,6-diamidino-2-phenylindole (DAPI) and 3-(acrylamido)phenylboronic acid (PBA-Ac) were purchased from Sigma. Poly(ethylene glycol) methyl ether acrylate (Mn ~480 Da, PEG-MeOAc) was purchased from Sigma and treated with inhibitor removers prior to use. Anti-YAP (63.7, mouse) and anti-vinculin (7F9, mouse) primary antibodies were purchased from Santa Cruz Biotech. Rhodamine-phalloidin and AlexaFluor488 donkey-anti-mouse secondary antibodies were purchased from Invitrogen. Lipofectamine 3000 kit was purchased from ThermoFisher. The Vinculin Tension Sensor (VinTS, Addgene plasmid # 26019), Tailless Vinculin Tension Sensor (VinTL, Addgene plasmid # 26020), and Vinculin-venus (Addgene plasmid # 27300) plasmids were donated by Martin Schwartz. The mTFP1-Lifeact-7 plasmid was a gift from Robert Campbell & Michael Davidson (Addgene plasmid # 54749). Lithium phenyl-2,4,6-trimethylbenzoylphosphinate (LAP) was synthesized according to a previously described route [28]. With the exception of PBA-Ac, which was dissolved in DMSO, all reagents were dissolved in ddH2O and adjusted to pH ~7. 1H-NMR spectra were collected on a 400 MHz Bruker NMR spectrometer.
2.2. Synthesis of 6-O-acryloyl methyl-α-D-mannopyranoside (Man-Ac)
The compound was prepared according to a previously published method [29]. Briefly, A flame dried 50mL round bottom flask was charged with anhydrous acetonitrile (30 mL), methyl-α-D-mannopyranoside (6 g, 31 mmol), Novozym 435 (3 g, 15,000 U), vinyl acrylate (3 mL, 28 mmol), and MEHQ (20 mg, 0.15 mmol). The reaction mixture was stirred for three days in the dark at 50 °C, after which the flask was charged with Novazym 435 (1 g, 5,000 U) and reacted an additional five days at 50 °C. The crude reaction mixture was separated with vacuum filtration over a glass frit with paper, the Novozym cake was washed with methanol (300 mL), and the resulting solution was adsorbed onto silica gel with rotary evaporation. The adsorbed crude product was transferred to an equilibrated silica column (Biotage, SNAP 100g) and purified on an Isolera One (Biotage) automated chromatography instrument with a mobile phase consisting of 6:3:1 ethyl acetate:hexanes:ethanol. The fractions collected after five column volumes was combined and concentrated with rotary evaporation to yield a yellow sap (0.74 g, 9.6%). TLC in 6:3:1 ethyl acetate:hexanes:ethanol revealed a single spot with Rf = 0.4, which was visualized with UV and PMA stain. 1H NMR (400 MHz, Deuterium Oxide) δ 6.42 (dd, J = 17.4, 1.1 Hz, 1H), 6.19 (dd, J = 17.4, 10.5 Hz, 1H), 5.95 (dd, J = 10.5, 1.1 Hz, 1H), 4.70 (d, J = 1.7 Hz, 1H), 4.48 (dd, J = 12.2, 2.2 Hz, 1H), 4.32 (dd, J = 12.2, 5.9 Hz, 1H), 3.90 (dd, J = 3.2, 1.7 Hz, 1H), 3.80 (ddd, J = 8.3, 5.9, 2.2 Hz, 1H), 3.76 – 3.64 (m, 2H), 3.34 (s, 3H). ESI+: expected [M+Na]+ = 271.08 g mol−1, found 271.08 g mol−1.
2.3. Synthesis of 6-O-acryloyl methyl-α-D-glucopyranoside (Glu-Ac)
The compound was prepared according to a previously published method [29]. A flame dried 50mL round bottom flask was charged with anhydrous acetonitrile (20 mL), methyl-α-D-glucopyranoside (4 g, 21 mmol), Novozym 435 (2 g, 10,000 U), vinyl acrylate (2 mL, 18.5 mmol), and MEHQ (13 mg, 0.1 mmol). The reaction mixture was stirred for three days in the dark at 50 °C, after which the flask was charged with additional Novazym 435 (0.7 g, 3,500 U) and reacted four more days at 50 °C. The crude reaction mixture was separated with vacuum filtration over a glass frit with paper, the Novozym cake was washed with methanol (300 mL), and the resulting solution was adsorbed onto silica gel with rotary evaporation. The adsorbed crude product was transferred to an equilibrated silica column (Biotage, SNAP 100g) and purified on an Isolera One (Biotage) automated chromatography instrument with a mobile phase consisting of 6:3:1 ethyl acetate:hexanes:ethanol. The fractions collected after five column volumes was combined and concentrated with rotary evaporation to yield a sweet-smelling yellow sap (1.7 g, 33%). TLC in 6:3:1 ethyl acetate:hexanes:ethanol revealed a single spot with Rf = 0.35, which was visualized with UV and PMA stain. 1H NMR (400 MHz, Deuterium Oxide) δ 6.41 (dt, J = 17.3, 0.9 Hz, 1H), 6.17 (ddd, J = 17.3, 10.5, 0.7 Hz, 1H), 5.95 (dt, J = 10.4, 0.8 Hz, 1H), 4.74 (d, J = 3.8 Hz, 1H), 4.45 (dd, J = 12.2, 2.2 Hz, 1H), 4.33 (dd, J = 12.3, 5.3 Hz, 1H), 3.84 (ddt, J = 10.6, 5.6, 2.9 Hz, 1H), 3.61 (d, J = 9.2 Hz, 1H), 3.52 (ddd, J = 9.8, 3.8, 0.7 Hz, 1H), 3.45 – 3.33 (m, 4H). ESI+: expected [M+Na]+ = 271.08 g mol−1, found 271.08 g mol−1.
2.4. Rheology
Rheology was performed on a TA Instruments DHR-3 strain-controlled rheometer equipped with an 8 mm parallel plate and a quartz plate accessory for controlled illumination. The hydrogel pre-polymer solution was prepared in PBS by mixing the cross-linker and monomer solutions to reach a total acrylate concentration of 700 mM, and a final concentration of 1 mM RGD-SH and 2 mM LAP. The pH of the resulting pre-polymer solutions was confirmed to be approximately 7. The pre-polymer solution was placed on the rheometer, the gap was lowered to 300 μm, and photo-polymerization was monitored in situ, at an oscillatory shear strain of 10% and a frequency of 1 rad s−1, during light exposure (Exfos Omnicure, 365 nm, 10 mW cm−2). Once the polymerization had completed, mineral oil was added to the gap to prevent dehydration, the axial force was zeroed and held at 1 N for all subsequent procedures. Frequency-sweep characterization of the G’ and G” was performed from 0.1 to 10 rad s−1 at an oscillatory shear strain of 10%, which was determined to be within the linear viscoelastic range.
For creep characterization, the strain was measured as samples were subjected to 1 kPa shear stress (rise time = 0.02 s) for 10 min, after which the stress was released, and the creep recovery strain was measured for an additional 10 min. A program was created in MATLAB to identify and eliminate data points in the creep-ringing regime, which is a resonance phenomenon characterized by rapidly oscillating J. Briefly, the moving variance was calculated from the previous 10 time points and those with a large moving variance (e.g., large amplitude oscillations) were eliminated. In order to calculate the fraction of total creep, viscoelastic model parameters were obtained by fitting the average creep compliance J(t) — at times after creep-ringing had subsided — to Equation 1, which describes creep in a five-element generalized Kelvin-Voigt model.
| J(t)=ϵ(t)σ=1E0+1E1*(1−e−tτc1)+1E2*(1−e−tτc2) | (Eq 1) |
|---|
For Equation 1, σ represents applied shear stress, ε represents shear strain, E i represents the elastic modulus of the i th spring element, η i represents the viscosity of the i th dashpot element and τ ci represents the retardation time constant of the i th Voigt element (i.e., η i /E i). The best-fit parameters obtained from Equation 1 were used to extrapolate the glassy creep compliance (J g ), which is equivalent to the instantaneous creep compliance resulting from a sudden imposition of stress. The Kelvin-Voigt creep model was used to compute the fraction of total creep for the experimental data according to Equation 2, where the subscript, e, represents equilibrium values, and the subscript, g, represents glassy values.
| ΔJ(t)Je−Jg=ϵ(t)−ϵ0ϵe−ϵg | (Eq 2) |
|---|
The approximate retardation spectra were calculated from J(t) with the method of Ferry and Williams, which provide Equations 3 – 5 [30].
| L(τ)=M(m)J(t)dlogJ(t)dlogt|t=τ | (Eq 3) |
|---|
Briefly, a series of 12 evenly spaced points were chosen from the doubly logarithmic plot of J(t) against t (4 points per decade). The value of the correction factor, M(m), was initially assigned to unity and the tentative retardation spectrum, L(τ), was calculated from Equation 3. The slope, m, of the doubly logarithmic plot of the tentative L(τ) was determined from Equation 5 and was used to calculate M(m) from Equation 4. Finally, the approximate L(τ) was acquired upon multiplication of the tentative L(τ) with M(m).
The approximate retardation spectra were calculated from the complex compliance (J* ) with the method of Ferry and Williams. The method follows an identical procedure as outlined above, but with Equations 6 – 8, and Equation 5 [30].
| L(τ)=−A(m)J′(ω)dlogJ′(ω)dlogω|(1ω)=τ | (Eq 6) |
|---|
| J′(ω)=G′(ω)G′(ω)2+G″(ω)2 | (Eq 7) |
|---|
2.5. Cell culture and imaging
2.5.1. Gel fabrication
The hydrogel pre-polymer solution was prepared sterilely by mixing the cross-linker and monomer solutions to reach a total acrylate concentration of 700 mM, and a final concentration of 1 mM RGD-SH and 2 mM LAP. The precursor solution was dropped onto a high-density polyethylene surface, then an acrylate functionalized glass coverslip was placed on top of the precursor solution. The gels were irradiated with 365 nm light at 4 mW cm−2 for 5 minutes, soaked in PBS for an additional 10 minutes, then carefully removed with a razor. The gels were washed two times and swollen overnight in sterile PBS.
2.5.2. Cell culture
NIH-3T3 fibroblasts (ATCC, CRL 1658) were thawed and passaged at 70–80% confluence at 37 °C and 5% CO2, with culture medium changes every 2–3 days (Gibco high glucose DMEM supplemented with 10% FBS, 50 U/mL of penicillin and streptomycin).
2.5.3. Immunofluorescent imaging and analysis
Cells were seeded at 3×103 cells cm−2 in fresh culture medium and on day four, the samples were fixed by replacing half of the culture medium with 4% ice-cold paraformaldehyde, and incubating at room temperature for 30 min. The fixative was then removed, and the samples were washed with PBS. The samples were immersed in PBST (0.1% Triton X-100 in PBS) for 1 hr to permeabilize cell membranes, followed by incubation in 5% bovine serum albumin (BSA) for 1 hr to block non-specific protein interactions. The blocked samples were then incubated in 5% BSA with anti-YAP (1:200, mouse) or anti-vinculin (1:200, mouse) primary antibody overnight at 4 °C. The samples were washed three times with PBST and then incubated in 1% BSA with rhodamine-phalloidin (1:300), DAPI (1 μg mL−1), and AlexaFluor488 donkey-anti-mouse for 1 hr. Finally, the samples were washed three times with PBS for 10 min prior to imaging on a laser scanning confocal microscope (Zeiss LSM710). Vinculin stained focal adhesions (FAs) of individual cells were imaged with a 63× objective (W Plan-Apochromat 1.0) and YAP localization was imaged with a 10× objective (Fluar 0.5). Image analysis was performed in FIJI [31]. For each image, the YAP nuclear:cytosolic ratio was determined by dividing the average YAP fluorescence intensity in the nuclei by the average YAP fluorescence intensity in the cytoplasm. Specifically, a binary mask of the nuclei was created from the DAPI channel and a mask of the cytoplasm was created from the YAP channel by subtracting the nuclei mask. Additionally, the average projected nuclear area was obtained from the nuclei mask, and the average cell area was calculated by dividing the combined area of the cytoplasmic and nuclear masks by the number of nuclei. The FAs of individual cells were identified by thresholding the vinculin image and manually selecting regions of interest (ROIs) containing the FAs. Vinculin particles in the ROIs that were between 0.3 μm2 and 5 μm2 in size were measured and the average FA area was recorded for each cell.
2.5.4. FRET imaging and analysis
NIH-3T3 fibroblasts at passage two were transfected with 4 ng DNA using the Lipofectamine 3000 kit according to the manufacturer’s instructions. Transfected NIH-3T3s were allowed to recover overnight, then seeded directly onto the gels at a density of 4×105 cells cm−2 in fresh imaging medium (High glucose FluoroBrite DMEM supplemented with 10% FBS, Gibco). The cells were grown on the hydrogels for 24 h, then washed gently with PBS and transferred to a glass bottom 24-well plate (Cellvis), upside-down, with fresh imaging medium. A rubber gasket was placed on top of the gel to preclude movement. The live cells were all imaged on the same day with an inverted spinning disk confocal microscope (Nikon Eclipse Ti-E) equipped with a 60× ELWD objective and an environmental chamber maintained at 37 °C and 5% CO2. Cells in clusters and cells that exhibited extremely high fluorescence were excluded from image acquisition. Images of the donor signal (mTFP1), the acceptor signal (vinculin-venus), and the FRET signal were acquired for each cell with 2×2 binning. Images of the donor and acceptor signal were obtained with CFP and YFP filter sets, respectively, and images of the FRET signal were collected with a 445 nm excitation laser and a YFP emission filter.
FAs in Vinculin-venus transfected cells and actin filaments in LifeAct-mTFP1 transfected cells, on glucose functionalized gels, were manually selected and used to determine the donor and acceptor bleed-through coefficients. The PixFRET plugin for FIJI [32] was then used to obtain images of the corrected FRET index by subtracting the background signal in addition to the bleed-through signal from the donor and acceptor channels. Specifically, a gaussian blur filter (σ = 0.1) was applied to each channel, pixels less than 1.5-fold the background were excluded, the background and bleed-through were subtracted from the FRET signal, and finally the corrected FRET index of each pixel was normalized to the donor intensity. FAs identified in the acceptor channel were used to create a binary mask by manual thresholding, which was subsequently applied to the normalized FRET index image. The average normalized FRET index for FA pixels was recorded for each cell.
2.6. Statistical Analysis
All statistical comparisons and nonlinear regressions were performed in Prism 6 (GraphPad Software, Inc). For all rheological experiments, three technical replicates were performed, and data is presented as the mean ± the standard deviation. For clarity, error bars smaller than the symbols were omitted. For all cellular experiments, three biological replicates were performed, the data was pooled together (n ≥ 9), and then the ROUT method was used to identify and exclude significant outliers. The remaining data were presented as the mean ± the 95% confidence interval. For the analysis of cellular area, projected nuclear area, and YAP subcellular localization, a minimum of three images containing at least 100 cells per image was captured for each biological replicate, and the average value for each image was recorded. For analysis of FA size and FRET ratio, a minimum of 6 cells were imaged for each biological replicate and the average value for each cell was recorded. Statistical comparisons between gel conditions were conducted with a one way ANOVA and Holm-Sidak post-hoc analysis.
3. Results and discussion
3.1. Synthesis of boronate ester hydrogels
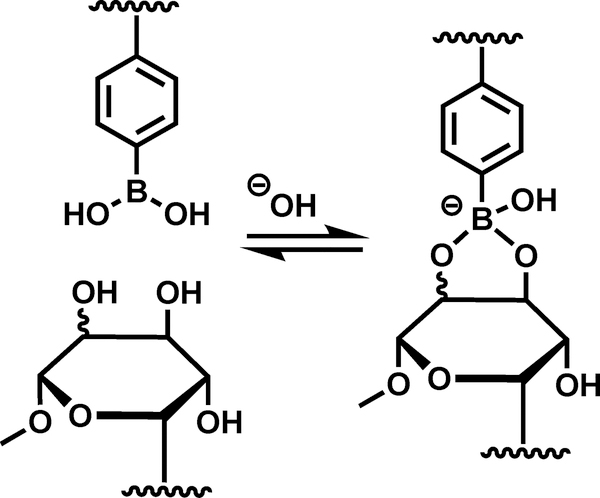
In this work, a poly(ethylene glycol) (PEG) based hydrogel was designed with stable PEG-diacrylate cross-links and adaptable boronate ester cross-links to enable tunable viscoelasticity. We reasoned that a reversible addition reaction, occurring between pendant reaction partners tethered to a polymer network, could impart viscoelastic characteristics via reversible crosslinking (Scheme 1). Two pyranose monosaccharide monomers, each with a unique diol configuration, were synthesized according to a modified protocol and incorporated into hydrogel scaffolds as pendant functionalities in order to control the adaptable cross-linking kinetics via hydroxyl configuration [29]. The first saccharide, 6-O-acryloyl methyl-α-D-glucopyranoside (Glu-Ac), contains two _trans_-_vic_-diol pairs whereas the second saccharide, 6-O-acryloyl methyl-α-D-mannopyranoside (Man-Ac), contains one _cis_-_vic_-diol pair and one _trans_-_vic_-diol pair. Importantly, the 4,6-diols of pendant saccharides are unable to participate in boronate complexation due to acrylate functionalization of the C6 hydroxyl, and additionally, methoxy functionalization of the C1 hydroxyl severely hinders pyranose-furanose interconversion and anomerization under physiological conditions in these saccharides [27]. The inability of Glu-Ac and Man-Ac to undergo pyranose-furanose interconversion is especially important considering that _cis_-_vic_-diol pairs in furanose isomers form much more stable boronate esters, which causes a shift in the pyranose-furanose equilibria for unsubstituted monosaccharides in the presence of bor(on)ic acids [21]. Together, the covalent modifications on C1 and C6 hydroxyls allowed us to carefully control hydrogel viscoelasticity via hydroxyl configuration, without concern of confounding effects arising from anomerization and 1,3-diol formation.
Scheme 1:
Covalent adaptability of the hydrogels is achieved through reversible boronate esterification of pendant PBA and _vic_-diols. Wavy line represent the hydrogel backbone.
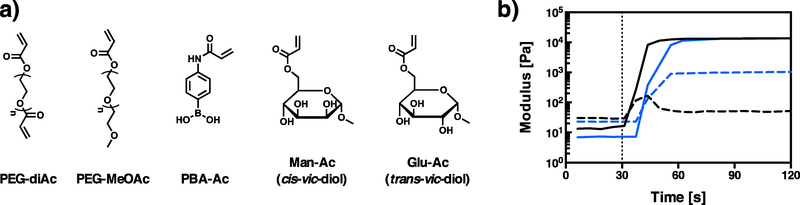
Hydrogels were fabricated in situ on a rheometer at physiologic pH via photo-polymerization of PEG-diacrylate (2,000 g mol−1, PEG-diAc) at 1 wt% or 0.1 wt%, PEG methyl ether acrylate (Mn 480 g mol−1, PEG-MeOAc), and the cell adhesion peptide RGD (1 mM), with lithium phenyl-2,4,6-trimethylbenzoylphosphinate (LAP, 2 mM) and 365 nm light at 10 mW cm−2 (Figure 1b); 3-(acrylamido)phenylboronic acid (PBA-Ac), and Glu-Ac or Man-Ac, were incorporated into the hydrogels at the concentrations recorded in Table S1. For consistency, the kinetic chain length of the polymers was kept approximately constant by maintaining a total acrylate concentration of 700 mM and the same initiation rate. Oscillatory rheological measurements of elastic hydrogels (Ela, Table S1) were taken during photo-polymerization and showed that network formation was complete within 15 s of exposure, reaching a plateau in the shear storage modulus (G’ ) of 13.8 kPa and shear loss modulus (G” ) of 0.04 kPa. In contrast, the polymerization of Man-Ac functionalized hydrogels (Man-100, Table S1) was retarded, reaching completion in 30 s and plateauing at a G’ of 13.8 kPa and G” of 1.1 kPa (Figure 1b). The significantly larger G” of the Man-100 gels compared to the Ela gels was ascribed to the covalent adaptability of the viscoelastic network derived from boronate ester formation.
Figure 1:
Design of adaptable hydrogels via reversible boronate esterification. (a) Chemical structures of monomers used to synthesize hydrogels via photo-polymerization. (b) Network formation occurs rapidly upon illumination (10 mW cm−2, 365 nm, 90 s), as indicated by the crossover of G’ (solid) and G” (dashed), for elastic hydrogels (black, Ela) and boronate ester functionalized hydrogels (blue, Man-100). Dotted line indicates the onset of illumination at 30 s.
2.2. Rheological characterization
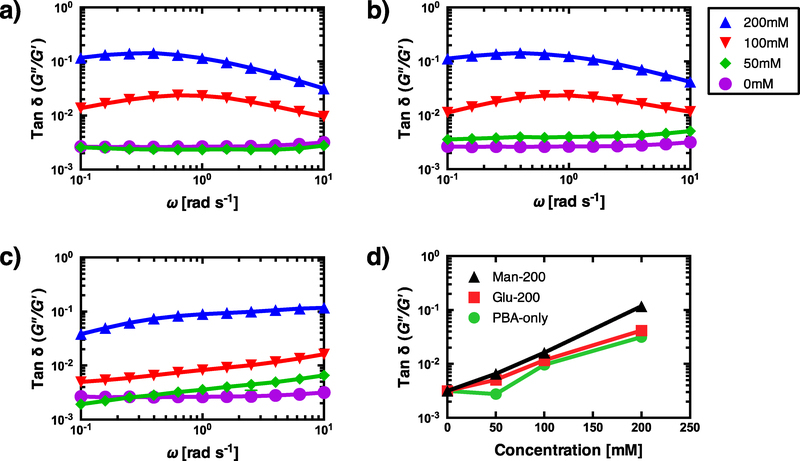
First, we examined the effect of PBA-Ac concentration on the viscoelastic properties of the hydrogels. Hydrogel viscoelastic properties were assessed as a function of PBA-Ac concentration (50 – 200 mM) by measuring the complex modulus (G’ & G” ) and tan δ at frequencies from 0.1 – 10 rad s−1. It was determined that the gels lacking PBA and saccharide moieties (Ela) were primarily elastic because the tan δ was independent of the frequency (Figure 2a). Surprisingly, we found that gels functionalized with >100 mM PBA-Ac were viscoelastic, as indicated by the frequency dependent tan δ spectra for PBA-100 and PBA-200 gels. The maximal tan δ (tan δMAX) increased significantly with PBA concentration from 0.02 to 0.12 for PBA-100 and PBA-200 gels, respectively. However, the frequency at which tan δMAX occurred in these gels was relatively unchanged (Figure 2a). The G’ also increased significantly with PBA concentration from 11.5 kPa to 21.1 kPa for PBA-100 and PBA-200 gels, respectively (Table 1 and Figure S2a). We attributed these observations to a reversible self-association reaction with pendant PBA moieties, such as hydrophobic interactions or boroxine formation, of which, the latter has been observed by others in aqueous solutions at concentrations above 100 mM [33,34]. Although the self-association reaction influences the overall magnitude of tan δ, it does not negatively affect our ability to tune the viscoelastic frequency spectra.
Figure 2:
Effect of PBA-Ac concentration on the viscoelastic properties of boronate hydrogels. (a) Frequency sweep of tan δ for hydrogels functionalized with PBA-Ac at 0 mM (purple circle, Ela), 50 mM (green diamond, PBA-50), 100 mM (red triangle, PBA-100), and 200 mM (blue triangle, PBA-200). (b) Frequency sweep of hydrogels functionalized with tethered _trans_-_vic_-diols (GluAc, 200 mM) and PBA (0 – 200 mM). (c) Frequency sweep of hydrogels functionalized with tethered _cis_-_vic_-diols (ManAc, 200 mM) and PBA (0 – 200 mM). (d) The tan δ (10 rad s−1) of hydrogels functionalized with PBA-only (green), _trans-vic_-diols (red, GluAc), and _cis_-_vic_-diols (black, ManAc) increase as a function of tethered PBA-Ac concentration. Data points represent the average measurement of three samples ± SD.
Table 1:
Hydrogel formulations used in this study. Concentrations are reported in mM. Storage (G’) and loss (G”) moduli were measured at 1 rad s−1. Formulations used in cellular experiments are highlighted.
| Ela | PBA 50 | PBA 100 | PBA 200 | Glu 100 | Glu 200 | Glu3 00 | Glu 400 | Man 100 | Man 200 | Man 300 | Man 400 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| PBA-Ac | 0 | 50 | 100 | 200 | 200 | 200 | 200 | 200 | 200 | 200 | 200 | 200 |
| Glu-Ac | 0 | 0 | 0 | 0 | 100 | 200 | 300 | 400 | 0 | 0 | 0 | 0 |
| Man-Ac | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 100 | 200 | 300 | 400 |
| PEG-MeO Ac | 700 | 650 | 700 | 500 | 400 | 300 | 200 | 100 | 400 | 300 | 200 | 100 |
| G’(kPa) | 13.8 | 12.1 | 11.5 | 21.1 | 16.8 | 14.8 | 12.8 | 9.3 | 13.8 | 11.6 | 9.8 | 6.5 |
| G“ (kPa) | 0.0 | 0.0 | 0.3 | 2.4 | 1.7 | 1.9 | 1.7 | 1.5 | 1.1 | 1.0 | 0.7 | 0.6 |
In order to determine if diols compete with the PBA self-association reaction, pendant _vic_-diols with two different configurations were incorporated into hydrogels at 200 mM by varying the PBA concentration between 50–200mM. The tan δ frequency spectra of hydrogels functionalized with PBA and _trans-vic_-diols (i.e., Glu-Ac) was practically identical to those of PBA-only hydrogels, and consequently, we concluded that PBA does not readily form boronate esters with _trans_-_vic_-diols (Figure 2b). The similar tan δ spectra of Glu-Ac and PBA-only hydrogels was presumably due to the low stability of borate esters formed with _trans_-_vic_-diols in pyranose structures (Keq = 0 – 1 M−1) [21,25,35]. In contrast to Glu-Ac gels, the tan δ frequency spectra of hydrogels functionalized with PBA and _cis-vic_-diols (i.e., Man-Ac) was markedly different from those of PBA-only hydrogels, which indicates that a new mode of viscoelasticity was achieved through boronate esterification of PBA and _cis_-_vic_-diols (Figure 2c). It is interesting to note that at 50 mM PBA-Ac, the tan δ spectra of Man-Ac functionalized gels was frequency dependent, as opposed to PBA-only gels. Additionally, it was found that the addition of untethered methyl-α-D-mannopyranoside (200 mM) to PBA-only gels and to Man-200 gels significantly decreased tan δ, presumably through competing and non-adaptable boronate esterification reactions (Figure S3). It was also observed that the tan δMAX of PBA-only hydrogels occurred at 0.6 rad s−1, which was significantly lower than all Man-Ac gels, where the tan δMAX occurred at ≥10 rad s−1. The higher frequency of tan δMAX for Man-Ac gels suggests that the average lifetime of boronate esters is shorter than the PBA self-association complex. Importantly, we found that the viscoelasticity from boronate esterification also increased with PBA concentration, as indicated by increased tan δ at 10 rad s−1 for Man-Ac gels (Figure 2d). Together, these results demonstrate that boronate esterification with _cis_-_vic_-diols significantly competes with the PBA self-reaction and also contributes to the viscoelasticity, which agrees with the larger stability of borate esters formed from _cis_-_vic_-diols in pyranose structures (Keq = 10 – 20 M−1) [21,25,35].
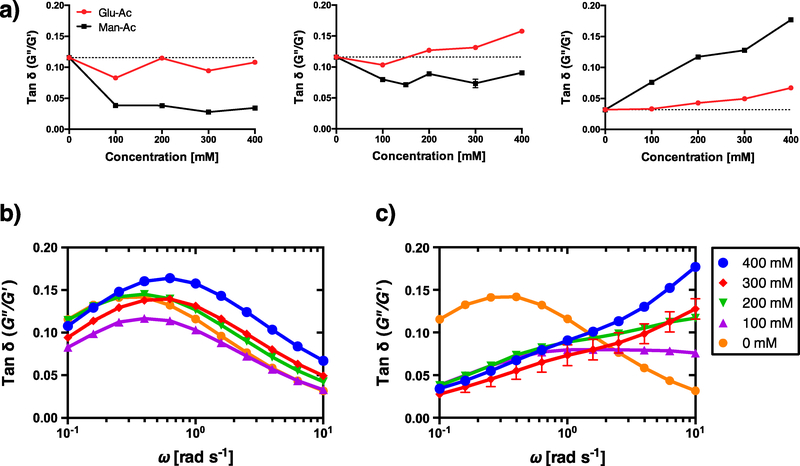
Next, we investigated the effect of diol concentration and configuration on the frequency spectra of tan δ in gels with 200 mM PBA. Interestingly, the G’ of the hydrogels decreased as a function of both the Glu-Ac concentration and Man-Ac concentration, which suggests that these saccharides interfere with the polymerization of acrylates (Figure S4a, b). Considering the retarded polymerization of Man-100 gels, and the decreased G’ of PBA-200 gels formed with the untethered mannopyranoside, it is likely that these saccharides inhibit the chain polymerization (Figure S3a). We noted that high frequency modes of viscoelasticity also scaled with Glu-Ac and Man-Ac concentration as indicated by increased tan δ at 10 rad s−1 (Figure 3a). Additionally, we noted that for Man-Ac functionalized hydrogels, the tan δ decreased at lower frequencies (e.g., 0.1 and 1 rad s−1). The tan δ spectrum of Glu-Ac functionalized gels was largely unaltered as the concentration of Glu-Ac increased, which agrees with our previous conclusion that Glu-Ac esterification does not significantly outcompete PBA self-association (Figure 3b). In contrast, one can see from the tan δ frequency spectra of Man-Ac hydrogels that as the Man-Ac concentration increased, the peak at 0.4 rad s−1 diminishes whilst a new peak emerges at ≥10 rad s−1 (Figure 3c). The alteration of the Man-Ac tan δ spectrum presumably corresponds to a transition from PBA self-association (0.4 rad s−1) to boronate esterification (≥ 10 rad s−1) with increased diol concentration. Interestingly, these results also suggest that the Keq of Man-Ac boronate esterification is large enough to compete with PBA self-association, and additionally, the koff rate of the PBA-Man complex is faster than that of the PBA self-association complex. Alternatively, it is possible that the high-frequency component of these spectra could represent an energy dissipative mechanism that is derived from the fundamentally different material properties that arise from Man-Ac incorporation. Regardless, these results show that the magnitudes of high-frequency loss tangents in these boronate ester hydrogels are positively correlated with diol concentration.
Figure 3:
Effect of _vic_-diol configuration and concentration on the viscoelasticity. (a) Tan δ measured at 0.1 rad s−1 (left), 1 rad s−1 (middle), and 10 rad s−1 (right), as a function of _trans_-vic (red, Glu-Ac) and _cis_-vic (black, Man-Ac) diol concentration. (b) Tan δ spectra of hydrogels functionalized with 200 mM PBA-Ac and Glu-Ac at 0 mM (orange circle), 100 mM (purple triangle), 200 mM (green triangle), 300 mM (red diamond), and 400 mM (blue circle). (c) Tan δ spectra of hydrogels functionalized with 200 mM PBA-Ac and Man-Ac at 0 – 400 mM Data points represent the average measurement of three samples ± SD.
To further verify that the viscoelasticity of these hydrogels is indeed dependent on the diol concentration and configuration, we measured creep resulting from 1 kPa shear stress over a 10 min period, and as expected, all gel formulations exhibited creep behavior (Figure S5). We also measured the strain for 10 min after the stress was released and found that all gel formulations exhibited elastic creep strain recovery and returned to their original shape on a timescale equivalent to the time needed to reach the equilibrium creep compliance (J e ) under applied stress. It was found that Glu-200 and Glu-400 gels shared essentially identical creep behavior with PBA-200, which is in accordance with the similar tan δ spectra of these gels (Figure S6a). In contrast, Man-200 and Man-400 gels both deformed quicker than PBA-200 gels (Figure S6b).
The methods of Ferry and Williams were used to calculate the approximate retardation spectrum (L), which represents the compliance magnitude at different timescales, using the experimental creep compliance, J(t), of each gel according to Equations 3–5 [30]. We found that the retardation spectrum of the Man-Ac gels was shifted towards shorter values of τ compared to PBA-only gels and Glu-Ac gels, which is in accordance with the shift of the tan δMAX to higher frequencies that was observed in the spectra of Man-Ac gels (Figure S6c). Moreover, L was also approximated from the frequency spectra of the complex modulus, according to Equations 6–8, and it was found that the values roughly matched the L spectra derived from creep experiments (Figure S6d). Furthermore, we identified a peak in the combined L spectra of PBA-only and Glu-Ac gels at approximately 3 s, which closely corresponds to the frequency of tan δMAX in these gels. For the combined L of Man-Ac gels, we identified a major peak at ≤0.1 s and a sub-peak at approximately 1 s, which presumably corresponds to the contribution of the boronate esterification and the PBA self-association, respectively, and agrees with the tan δ spectra of these gels. Collectively, the combined approximate L spectra corroborated the fidelity of dynamic and transient rheological experiments.
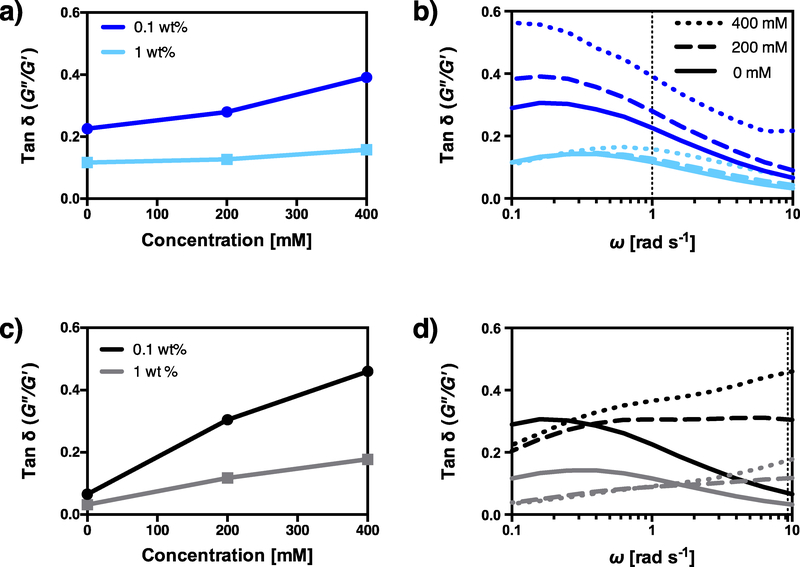
We next examined the effect of the cross-link density on the viscoelastic properties. First, we prepared PBA-200, Glu-200, and Glu-400 gels at 1 wt% and 0.1 wt% PEG-diAc. It was found that the tan δ for low cross-link density gels was much larger compared to the corresponding high cross-link density gels. Additionally, the tan δ (1 rad s−1) varied significantly more with Glu-Ac concentration in low cross-link density gels compared to high cross-link density gels (Figure 4a). Upon closer inspection of the tan δ frequency spectra of Glu-Ac hydrogels, one can see that tan δMAX occurs at a significantly lower frequency for low cross-link density gels (Figure 4b). Next, we prepared PBA-200, Man-200, and Man-400 gels at 1 wt% and 0.1 wt% PEG-diAc. Again, the tan δ for low cross-link density gels was much larger than the corresponding high cross-link density gels. Additionally, the tan δ (10 rad s−1) was more dependent on Man-Ac concentration in low cross-link density gels (Figure 4c). In contrast to Glu-Ac gels, however, the frequency spectra of low cross-link density Man-Ac gels were only slightly shifted towards lower frequencies (Figure 4d). Interestingly, the loss moduli for low cross-link density gels only differed from the corresponding high cross-link density gels by a factor of approximately 2, whereas the storage moduli differed by an order of magnitude (Figure S7a, b). While the difference in the storage moduli is clearly due to the cross-link density, the similarity of the loss moduli may be ascribed to equivalent PBA-Ac and diol concentrations — and thus, similar esterification kinetics — between high and low cross-link density gels. Altogether, the cross-linking density provides another method to tune the hydrogel viscoelasticity by modulating the G’ independent of the G”. By precisely tuning the viscoelastic frequency spectrum of this system, we next investigated the timescales at which cells likely probe the biophysical signals of their microenvironment.
Figure 4:
Effect of cross-linking density on viscoelasticity. (a) The tan δ measured at 1 rad s−1 for Glu-Ac functionalized hydrogels with a high cross-link density (cyan squares, 1 wt%) or a low cross-link density (blue circles, 0.1 wt%). (b) The tan δ frequency spectrum of hydrogels functionalized with Glu-Ac at 0 mM (solid), 200 mM (dashed), or 400 mM (dotted), at a high cross-link density (cyan) or a low cross-link density (blue). (c) The tan δ measured at 10 rad s−1 for Man-Ac functionalized hydrogels with a high crosslink density (grey squares, 1 wt%) or a low cross-link density (black circles, 0.1 wt%). (d) The tan δ frequency spectrum of hydrogels functionalized with Man-Ac at 0 mM (solid), 200 mM (dashed), or 400 mM (dotted), at a high cross-link density (grey) or a low cross-link density (black). Data points represent the average measurement of three samples ± SD.
2.3. Cellular response to the complex moduli spectra
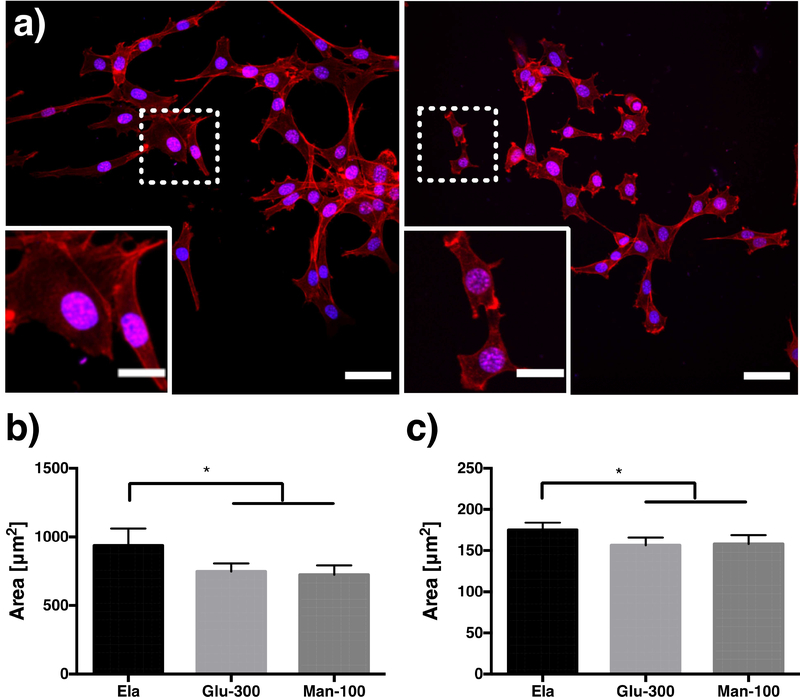
In an effort to determine the role that the spectrum of the complex modulus plays in cellular mechano-transduction, we cultured NIH-3T3 cells — which are known to be mechanosensitive [36–38] — for four days on glass, Ela, Glu-300, and Man-100 gels. It is important to note that we chose formulations of Ela, Glu-Ac, and Man-Ac gels that had similar G’ (13kPa, 1 rad s−1) to minimize the variation of substrate elasticity, which can lead to confounding effects that have been studied in great detail [4,39–41]. Over the course of the experiment, the fibroblast cell line spread and proliferated on the gels, indicating that the hydrogels were indeed compatible for promoting cell interactions and expansion (Figure 5a). To quantify the fibroblast interactions with the hydrogels, we first measured the projected cell area and the projected nuclear area; results showed statistically significant differences between the elastic gel and the viscoelastic gels (Figure 5b, c). We also noted that the projected nuclear area of cells cultured on glass coverslips was significantly larger than that of cells cultured on Ela gels (p < 0.001), although, there was no statistically significant difference found for the projected cell area (Fig S10a, b). Together, these data illustrated a trend of decreased projected cell area and projected nuclear area for cells cultured on viscoelastic hydrogels compared to elastic materials. No clear differences were observed between the substrates offering different frequency specific viscoelastic properties and these cellular morphologies.
Figure 5:
The effect of viscoelasticity on cellular morphology. (a) Representative images of cells stained for DAPI (blue) and f-actin (red) on elastic gels (left, Ela) and viscoelastic hydrogels (right, Man-Ac). Scale bar = 50 μm. Inset shows higher magnification image of the region in the dotted box, scalebar = 20 μm. The projected cell area (b) and the projected nuclear area (c) of cells cultured on elastic gels (Ela) and viscoelastic gels (Glu-100 and Man-100). Each data point represents the average and 95% CI of n≥9 images containing ≥900 cells total (* p < 0.05).
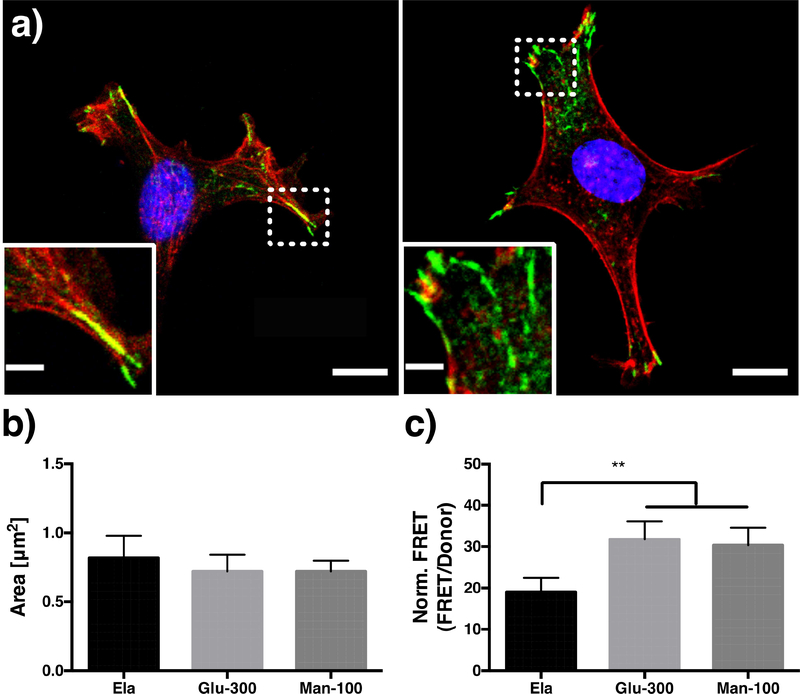
To assess the influence of the complex modulus spectrum on FA size, we stained cells for vinculin, a component of FAs. We observed that NIH 3T3s cultured on elastic substrates displayed prominent stress fibers terminating in large FAs, while the same cells on viscoelastic gels generally displayed smaller stress fibers, an actin cortex, and slightly smaller punctate FAs located at the cell periphery (Figure 6a, b). We then measured FA tension in cells expressing a Förster resonance energy transfer (FRET) based vinculin tension-sensor probe (VinTS) in an attempt to elucidate the underlying mechanism behind the morphological differences that we observed (Figure S8) [42]. Vinculin is situated in the “force transduction” layer of FAs where the head domain binds talin and the tail domain binds actin and paxillin [43]; consequently, the donor and acceptor fluorophores of VinTS are separated by the generation of active actomyosin tension, resulting in decreased FRET [42]. We first verified that the VinTS construct showed FRET and was capable of sensing substrate elasticity by expressing decreased FRET signal with increasing stiffness (Figure S9a, b). We observed that the FRET signal normalized to the donor signal was significantly enhanced on viscoelastic hydrogels (Glu-300 and Man-100) compared to elastic gels, revealing that FA tension was lower for cells on the viscoelastic gels (Figure 6c). We also found that the FRET signal of cells on viscoelastic gels was close to cells expressing a tail-less VinTS mutant, which lacks the actin binding domain and is thus perpetually in a low-tension configuration (Figure S9c) [42]. Additionally, there was no statistically significant difference between the FRET index of cells cultured on Ela gels and glass substrates (Figure S10d); given that cytoskeletal tension is known to increase as a function of substrate G’, this result indicates that the G’ of Ela gels is sufficient to elevate cytoskeletal tension to be similar to glass [44]. Together, these findings further demonstrate that FA tension is lowered on viscoelastic hydrogels compared to elastic gels with comparable G’. Finally, our results also suggest that low FA tension corresponds to smaller FA size, which is consistent with previous work demonstrating that cytoskeletal stress stabilizes and enlarges FAs [1,45].
Figure 6:
The effect of viscoelasticity on FA size and tension. (a) Representative images of a cell stained for DAPI (blue), f-actin (red), and vinculin (green) on elastic gels (left, Ela) and viscoelastic hydrogels (right, Man-Ac). Scale bar = 10 μm. Inset shows higher magnification image of the region in the dotted box, scalebar = 3 μm. The FA area (b) and the normalized FRET index (c) of cells cultured on elastic gels (Ela) and viscoelastic gels (Glu-100 and Man-100)‥ Each data point represents the average and 95% CI of n≥13 cells (** p < 0.01).
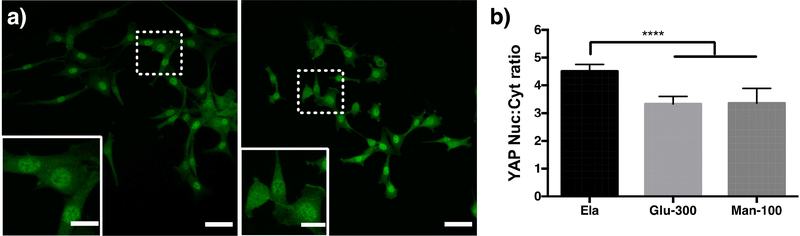
To better understand if biomechanical signals originating at the cell-matrix interface are also converted into biochemical signals in a frequency dependent manner, we stained for cellular pools of Yes associated protein (YAP) and transcriptional coactivator with PDZ-binding motif (TAZ) (Figure 7a), a transcriptional coactivator known to translocate into the nucleus with high cytoskeletal stress [46]. We found that the nuclear to cytosolic ratio of YAP/TAZ was significantly decreased in cells cultured on the viscoelastic gels compared to the elastic gel (Figure 7b). Furthermore, YAP/TAZ nuclear localization was found to be closely related to the level of cytoskeletal stress, which agrees with previous work [46]. Taken together, these results further demonstrate the ability of FAs to transduce biomechanical signals at the cell-matrix interface into intracellular biochemical signals that affect transcriptional activity. While we did not observe statistically significant differences in any of the cellular readouts between the two different viscoelastic gels (e.g., Glu-300 and Man-100), it is possible that the viscoelastic properties at the frequencies relevant for mechanotransduction are more similar than our rheological characterization has revealed. These similarities could be caused by, for example, cell mechano-sensing at a frequency outside of the frequency sweep window investigated, cell mechano-sensing by integration of material properties over a range of frequencies, or by hydrogel swelling, which may alter the tan δ spectrum. Interestingly, the creep retardation compliance was very similar for Glu-Ac and Man-Ac functionalized gels at very long timescales (e.g., 1 × 102 – 1 × 103 s), which supports the former two hypotheses (Figure S6c).
Figure 7:
The effect of viscoelasticity on sub-cellular YAP localization. (a) Representative images of cells stained for YAP on elastic gels (left, Ela) and viscoelastic hydrogels (right, Man-100). Scale bar = 50 μm. Inset shows higher magnification image of the region in the dotted box, scalebar = 20 μm. (b) Ratio of nuclear to cytosolic YAP for cells cultured on elastic gels (Ela) and viscoelastic gels (Glu-100 and Man-100). Each data point represents the average and 95% CI of n≥9 images containing ≥900 cells total (**** p < 0.0001).
In comparison with other reports in the literature that investigated viscoelastic mechanotransduction in two-dimensional cultures (i.e., cells residing on the surface of the hydrogel), the results presented herein were different from those of Chaudhuri et al. and Cameron et al., in which cell spreading and YAP/TAZ nuclear localization increased as a function of tan δ [12,17]. However, our boronate ester gels are distinguishable from the aforementioned systems with respect to plasticity; that is to say, our gels display elastic recovery of creep strain and return to their original shape upon the removal of shear stress (Figure S5). The absence of plastic deformation in our system is noteworthy because the clustering of adhesive ligands through plastic remodeling of the substrate is thought to be an important factor in enhanced spreading on viscoelastic substrates [17]. Accordingly, a recent work from Charrier et al. has demonstrated that the projected area and FA size of cells decreased when cultured on viscoelastic gels lacking plastic deformation, which is in good agreement with our results [10]. An additional difference between the system presented herein and that of Chaudhuri et al. is the glassy compliance (J g ), where the J g of our gels is an order of magnitude smaller. In fact, the model developed by Chaudhuri et al. predicts that cell spreading on the surface of viscoelastic substrates with a small J g will be less than cell spreading on primarily elastic substrates with an equivalent J g, which is also in good agreement with our results [17]. The results presented herein were also different from those of Tang et al., where the cell volume, nuclear volume, and YAP/TAZ nuclear localization of hMSCs in three-dimensional (3D) cultures (i.e., cells encapsulated within the hydrogel) were enhanced for viscoplastic boronate hydrogels compared to primarily elastic hydrogels [19]. However, two- and three-dimensional cell cultures are disparate cellular environments, where the surrounding matrix of 3D cultures hinders cell spreading, although, viscoplastic hydrogels can accommodate cell spreading and proliferation in 3D cultures via plastic deformation [9,47]. Collectively, these reports complement one another and illustrate a distinction between mechanotransduction on viscoelastic and viscoplastic substrates, and between mechanotransduction in two- and three-dimensional cultures.
3. Conclusions
In summary, the adaptable boronate ester hydrogels that we developed are biocompatible and provide a means to probe timescale specific cell-matrix interactions. The frequency-dependent viscoelastic properties were tuned by leveraging the equilibrium kinetics of boronate esterification in covalent adaptable cross-links. Specifically, the _vic_-diol configuration and concentration, as well as the self-association of PBA, afforded control of the G” frequency spectrum, while the cross-link density afforded control of the G’. The average value of tan δ scaled with PBA-Ac concentration, as well as _vic_-diol concentration; additionally, the tan δMAX shifted to higher frequencies with increased _vic_-diol concentration. Finally, we demonstrated that the cell area, nuclear area, focal adhesion area, focal adhesion tension, and YAP/TAZ sub-cellular localization were significantly different between the elastic gels and boronate ester-based viscoelastic gels even though they have comparable G’. We did not, however, find a statistically significant relationship between the frequency dependent viscoelastic material properties characterized in this study and cellular morphology, focal adhesion tension or subcellular YAP localization. Further work is needed to elucidate the range of timescales in which mechanotransduction occurs, where additional viscoelastic materials with distinct frequency spectra are characterized over a broader range of frequencies.
Supplementary Material
1
Figure S1: NMR spectra of Glu-Ac and Man-Ac
Figure S2: Frequency sweeps of the storage and loss modulus for gels functionalized with varying concentrations of PBA-Ac.
Figure S3: Inhibition of hydrogel viscoelasticity with untethered methyl-α-D-mannopyranoside.
Figure S4: Frequency sweeps of the storage and loss modulus for gels functionalized with varying concentrations and configurations of _vic_-diols.
Figure S5: Creep recovery of viscoelastic hydrogels.
Figure S6: Effect of _vic_-diol configuration and concentration on creep
Figure S7: Frequency sweeps of the storage and loss modulus for gels functionalized with varying concentrations of PEG-diAc cross-linker.
Figure S8: Representative FRET images
Figure S9: Validation of the vinculin tension sensor construct.
Acknowledgments
This work was supported financially in part by grants from the National Science Foundation (DMR 1408955), the National Institutes of Health (R01 DE016523), and the Australian Research Council Discovery Grant (DP140104217). The authors also gratefully acknowledge fellowship support from the NSF GFRP (I.A.M) and from the Whitaker International Program (I.A.M.). FRET imaging was performed at the BioFrontiers Institute Advanced Light Microscopy Core on a Nikon Ti-E microscope supported by the Howard Hughes Medical Institute. The authors would like to thank Dr. Joseph Dragavon for helpful discussions regarding FRET imaging, Dr. Kemal Arda Günay for critically reading the manuscript, as well as Dr. Pingping Han for assistance with plasmid production and transfection, Dr. Benoit Maisonneuve for helpful rheological discussions, and Prof. Martina Stenzel for insightful chemistry discussions.
Footnotes
Data availability
The raw data required to reproduce these findings are available to download from [INSERT PERMANENT WEB LINK(s)]. The processed data required to reproduce these findings are available to download from [INSERT PERMANENT WEB LINK(s)].
Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.
References
- [1].Wang N, Tytell JD, Ingber DE, Mechanotransduction at a distance: mechanically coupling the extracellular matrix with the nucleus, Nat. Rev. Mol. Cell Biol 10 (2009) 75–82. doi: 10.1038/nrm2594. [DOI] [PubMed] [Google Scholar]
- [2].Tajik A, Zhang Y, Wei F, Sun J, Jia Q, Zhou W, Singh R, Khanna N, Belmont AS, Wang N, Transcription upregulation via force-induced direct stretching of chromatin, Nat. Mater 15 (2016) 1287–1296. doi: 10.1038/nmat4729. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [3].Elosegui-Artola A, Andreu I, Beedle AEM, Lezamiz A, Uroz M, Kosmalska AJ, Oria R, Kechagia JZ, Rico-Lastres P, Le Roux AL, Shanahan CM, Trepat X, Navajas D, Garcia-Manyes S, Roca-Cusachs P, Force Triggers YAP Nuclear Entry by Regulating Transport across Nuclear Pores, Cell. 171 (2017) 1397–1410.e14. doi: 10.1016/j.cell.2017.10.008. [DOI] [PubMed] [Google Scholar]
- [4].Engler AJ, Sen S, Sweeney HL, Discher DE, Matrix elasticity directs stem cell lineage specification, Cell. 126 (2006) 677–689. doi: 10.1016/j.cell.2006.06.044. [DOI] [PubMed] [Google Scholar]
- [5].Chaudhuri O, Gu L, Klumpers D, Darnell M, Bencherif SA, Weaver JC, Huebsch N, Lee H, Lippens E, Duda GN, Mooney DJ, Hydrogels with tunable stress relaxation regulate stem cell fate and activity, Nat. Mater 15 (2015) 326–334. doi: 10.1038/nmat4489. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [6].Nam S, Lee J, Brownfield DG, Chaudhuri O, Viscoplasticity Enables Mechanical Remodeling of Matrix by Cells, Biophys. J 111 (2016) 2296–2308. doi: 10.1016/j.bpj.2016.10.002. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [7].Vinogradov GV, Malkin AY, Plotnikova EP, Sabsai OY, Nikolayeva NE, Viscoelastic Properties of Filled Polymers, 1972. doi: 10.1080/00914037208075296. [DOI] [Google Scholar]
- [8].Ferry JD, Viscoelastic properties of polymers, 1980. doi: 10.1080/00914037208075296. [DOI] [Google Scholar]
- [9].Richardson BM, Wilcox DG, Randolph MA, Anseth KS, Hydrazone covalent adaptable networks modulate extracellular matrix deposition for cartilage tissue engineering, Acta Biomater. (2018). doi: 10.1016/j.actbio.2018.11.014. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [10].Charrier EE, Pogoda K, Wells RG, Janmey PA, Control of cell morphology and differentiation by substrates with independently tunable elasticity and viscous dissipation, Nat. Commun 9 (2018) 1–13. doi: 10.1038/s41467-018-02906-9. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [11].Murrell M, Kamm R, Matsudaira P, Substrate viscosity enhances correlation in epithelial sheet movement, Biophys. J 101 (2011) 297–306. doi: 10.1016/j.bpj.2011.05.048. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [12].Cameron AR, Frith JE, Cooper-White JJ, The influence of substrate creep on mesenchymal stem cell behaviour and phenotype, Biomaterials. 32 (2011) 5979–5993. doi: 10.1016/j.biomaterials.2011.04.003. [DOI] [PubMed] [Google Scholar]
- [13].Cameron AR, Frith JE, Gomez GA, Yap AS, Cooper-White JJ, The effect of time-dependent deformation of viscoelastic hydrogels on myogenic induction and Rac1 activity in mesenchymal stem cells., Biomaterials. 35 (2014) 1857–68. doi: 10.1016/j.biomaterials.2013.11.023. [DOI] [PubMed] [Google Scholar]
- [14].Plotnikov SV, Pasapera AM, Sabass B, Waterman CM, Force fluctuations within focal adhesions mediate ECM-rigidity sensing to guide directed cell migration, Cell. 151 (2012) 1513–1527. doi: 10.1016/j.cell.2012.11.034. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [15].Chan CE, Odde DJ, Traction dynamics of filopodia on compliant substrates, Science (80-. ). 322 (2008) 1687–1691. doi: 10.1126/science.1163595. [DOI] [PubMed] [Google Scholar]
- [16].Bangasser BL, Rosenfeld SS, Odde DJ, Determinants of Maximal Force Transmission in a Motor-Clutch Model of Cell Traction in a Compliant Microenvironment, Biophys. J 105 (2013) 581–592. doi: 10.1016/j.bpj.2013.06.027. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [17].Chaudhuri O, Gu L, Darnell M, Klumpers D, Bencherif SA, Weaver JC, Huebsch N, Mooney DJ, Substrate stress relaxation regulates cell spreading, Nat. Commun 6 (2015) 6365. doi: 10.1038/ncomms7365. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [18].Saito A, Konno T, Ikake H, Kurita K, Ishihara K, Control of cell function on a phospholipid polymer having phenylboronic acid moiety., Biomed. Mater 5 (2010) 054101. doi: 10.1088/1748-6041/5/5/054101. [DOI] [PubMed] [Google Scholar]
- [19].Tang S, Ma H, Tu HC, Wang HR, Lin PC, Anseth KS, Adaptable Fast Relaxing Boronate-Based Hydrogels for Probing Cell–Matrix Interactions, Adv. Sci 5 (2018) 1–8. doi: 10.1002/advs.201800638. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [20].Cambre JN, Sumerlin BS, Biomedical applications of boronic acid polymers, Polymer (Guildf). 52 (2011) 4631–4643. doi: 10.1016/j.polymer.2011.07.057. [DOI] [Google Scholar]
- [21].Peters JA, Interactions between boric acid derivatives and saccharides in aqueous media: Structures and stabilities of resulting esters, Coord. Chem. Rev 268 (2014) 1–22. doi: 10.1016/j.ccr.2014.01.016. [DOI] [Google Scholar]
- [22].Brooks WLA, Sumerlin BS, Synthesis and Applications of Boronic Acid-Containing Polymers: From Materials to Medicine, Chem. Rev. 116 (2016) 1375–1397. doi: 10.1021/acs.chemrev.5b00300. [DOI] [PubMed] [Google Scholar]
- [23].Smithmyer ME, Deng CC, Cassel SE, Levalley PJ, Sumerlin BS, Kloxin AM, Self-Healing Boronic Acid-Based Hydrogels for 3D Co-cultures, ACS Macro Lett. 7 (2018) 1105–1110. doi: 10.1021/acsmacrolett.8b00462. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [24].Deng CC, Brooks WLA, Abboud KA, Sumerlin BS, Boronic acid-based hydrogels undergo self-healing at neutral and acidic pH, ACS Macro Lett. 4 (2015) 220–224. doi: 10.1021/acsmacrolett.5b00018. [DOI] [PubMed] [Google Scholar]
- [25].van den Berg R, Peters JA, van Bekkum H, The structure and (local) stability constants of borate esters of mono- and di-saccharides as studied by11B and13C NMR spectroscopy, Carbohydr. Res. 253 (1994) 1–12. doi: 10.1016/0008-6215(94)80050-2. [DOI] [PubMed] [Google Scholar]
- [26].Martínez-Aguirre MA, Villamil-Ramos R, Guerrero-Alvarez JA, Yatsimirsky AK, Substituent effects and pH profiles for stability constants of arylboronic acid diol esters, J. Org. Chem 78 (2013) 4674–4684. doi: 10.1021/jo400617j. [DOI] [PubMed] [Google Scholar]
- [27].Dawber JCG, Green SIE, Dawber JCG, Gabrail S, A polarimetric and 11B and 13C nuclear magnetic resonance study of the reaction of the tetrahydroxyborate ion with polyols and carbohydrates, J. Chem. Soc. Faraday Trans. 1 84 (1988) 41–56. doi: 10.1039/F19888400041. [DOI] [Google Scholar]
- [28].Fairbanks BD, Schwartz M, Bowman CN, Anseth KS, Photoinitiated polymerization of PEG-diacrylate with lithium phenyl-2, 4, 6-trimethylbenzoylphosphinate: polymerization rate and cytocompatibility, Biomaterials. 30 (2009) 6702–6707. doi: 10.1016/j.biomaterials.2009.08.055.Photoinitiated. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [29].Albertin L, Stenzel MH, Barner-Kowollik C, Foster LJR, Davis TP, Well-defined diblock glycopolymers from RAFT polymerization in homogeneous aqueous medium, Macromolecules. 38 (2005) 9075–9084. doi: 10.1021/ma051310a. [DOI] [Google Scholar]
- [30].Ferry JD, Williams ML, Second approximation methods for determining the relaxation time spectrum of a viscoelastic material, J. Colloid Sci 7 (1952) 347–353. doi: 10.1016/0095-8522(52)90001-9. [DOI] [Google Scholar]
- [31].Schindelin J, Arganda-Carreras I, Frise E, Kaynig V, Longair M, Pietzsch T, Preibisch S, Rueden C, Saalfeld S, Schmid B, Tinevez JY, White DJ, Hartenstein V, Eliceiri K, Tomancak P, Cardona A, Fiji: An open-source platform for biological-image analysis, Nat. Methods 9 (2012) 676–682. doi: 10.1038/nmeth.2019. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [32].Feige JN, Sage D, Wahli W, Desvergne B, Gelman L, PixFRET, an ImageJ plug-in for FRET calculation that can accommodate variations in spectral bleed-throughs, Microsc. Res. Tech 68 (2005) 51–58. doi: 10.1002/jemt.20215. [DOI] [PubMed] [Google Scholar]
- [33].Ingri N, Sillen L, Equilibrium studies of polyanions, Acta Chem. Scand 11 (1957) 1034–1058. [Google Scholar]
- [34].Smith HD, Wiersema RJ, Boron-11 Nuclear Magnetic Resonance Study of Polyborate Ions in Solution, Inorg. Chem 11 (1972) 1152–1154. doi: 10.1021/ic50111a056. [DOI] [Google Scholar]
- [35].Lorand JP, Edwards JO, Polyol Complexes and Structure of the Benzeneboronate Ion, J. Org. Chem 24 (1959) 769–774. doi: 10.1021/jo01088a011. [DOI] [Google Scholar]
- [36].Hinz B, Celetta G, Tomasek JJ, Gabbiani G, Chaponnier C, Alpha-Smooth Muscle Actin Expression Upregulates Fibroblast Contractile Activity, Mol. Biol. Cell 12 (2001) 2730–2741. doi: 10.1091/mbc.12.9.2730. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [37].Beningo KA, Dembo M, Wang Y. l. Y. -l., Responses of fibroblasts to anchorage of dorsal extracellular matrix receptors, Proc. Natl. Acad. Sci 101 (2004) 18024–18029. doi: 10.1073/pnas.0405747102. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [38].Bellin RM, Kubicek JD, Frigault MJ, Kamien AJ, Steward RL, Barnes HM, DiGiacomo MB, Duncan LJ, Edgerly CK, Morse EM, Park CY, Fredberg JJ, Cheng C-M, LeDuc PR, Defining the role of syndecan-4 in mechanotransduction using surface-modification approaches, Proc. Natl. Acad. Sci 106 (2009) 22102–22107. doi: 10.1073/pnas.0902639106. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [39].Kloxin AM, Benton JA, Anseth KS, In situ elasticity modulation with dynamic substrates to direct cell phenotype, Biomaterials. 31 (2010) 1–8. doi: 10.1016/j.biomaterials.2009.09.025. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [40].Swift J, Ivanovska IL, Buxboim A, Harada T, Dingal PCDP, Pinter J, Pajerowski JD, Spinler KR, Shin JW, Tewari M, Rehfeldt F, Speicher DW, Discher DE, Nuclear lamin-A scales with tissue stiffness and enhances matrix-directed differentiation, Science (80-. ). 341 (2013). doi: 10.1126/science.1240104. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [41].Wen JH, Vincent LG, Fuhrmann A, Choi YS, Hribar KC, Taylor-Weiner H, Chen S, Engler AJ, Interplay of matrix stiffness and protein tethering in stem cell differentiation, Nat. Mater 13 (2014) 979–987. doi: 10.1038/nmat4051. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [42].Grashoff C, Hoffman BD, Brenner MD, Zhou R, Parsons M, Yang MT, McLean MA, Sligar SG, Chen CS, Ha T, Schwartz MA, Measuring mechanical tension across vinculin reveals regulation of focal adhesion dynamics, Nature. 466 (2010) 263–266. doi: 10.1038/nature09198. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [43].Kanchanawong P, Shtengel G, Pasapera AM, Ramko EB, Davidson MW, Hess HF, Waterman CM, Nanoscale architecture of integrin-based cell adhesions, Nature. 468 (2010) 580–584. doi: 10.1038/nature09621. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [44].Solon J, Levental I, Sengupta K, Georges PC, Janmey PA, Fibroblast adaptation and stiffness matching to soft elastic substrates, Biophys. J 93 (2007) 4453–4461. doi: 10.1529/biophysj.106.101386. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [45].Sun Z, Guo SS, Fässler R, Integrin-mediated mechanotransduction J Cell Biol. 215 (2016) 445–456. doi: 10.1083/jcb.201609037. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [46].Dupont S, Morsut L, Aragona M, Enzo E, Giulitti S, Cordenonsi M, Zanconato F, Le Digabel J, Forcato M, Bicciato S, Elvassore N, Piccolo S, Role of YAP/TAZ in mechanotransduction, Nature. 474 (2011) 179–183. doi: 10.1038/nature10137. [DOI] [PubMed] [Google Scholar]
- [47].Brown TE, Carberry BJ, Worrell BT, Dudaryeva OY, McBride MK, Bowman CN, Anseth KS, Photopolymerized dynamic hydrogels with tunable viscoelastic properties through thioester exchange, Biomaterials. 178 (2018) 496–503. doi: 10.1016/j.biomaterials.2018.03.060. [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
1
Figure S1: NMR spectra of Glu-Ac and Man-Ac
Figure S2: Frequency sweeps of the storage and loss modulus for gels functionalized with varying concentrations of PBA-Ac.
Figure S3: Inhibition of hydrogel viscoelasticity with untethered methyl-α-D-mannopyranoside.
Figure S4: Frequency sweeps of the storage and loss modulus for gels functionalized with varying concentrations and configurations of _vic_-diols.
Figure S5: Creep recovery of viscoelastic hydrogels.
Figure S6: Effect of _vic_-diol configuration and concentration on creep
Figure S7: Frequency sweeps of the storage and loss modulus for gels functionalized with varying concentrations of PEG-diAc cross-linker.
Figure S8: Representative FRET images
Figure S9: Validation of the vinculin tension sensor construct.