Euclid's Elements, Book I, Proposition 23 (original) (raw)

To construct a rectilinear angle equal to a given rectilinear angle on a given straight line and at a point on it.

Let the angle DCE be the given rectilinear angle, AB the given straight line, and A the point on it.

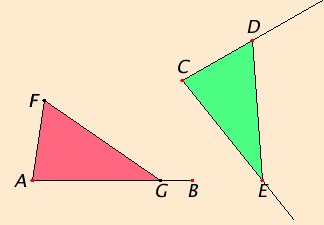
It is required to construct a rectilinear angle equal to the given rectilinear angle DCE on the given straight line AB and at the point A on it.

Take the points D and E at random on the straight lines CD and CE respectively, and join DE. Out of three straight lines which equal the three straight lines CD, DE, and CE construct the triangle AFG in such a way that CD equals AF, CE equals AG, and DE equals FG.

Since the two sides DC and CE equal the two sides FA and AG respectively, and the base DE equals the base FG, therefore the angle DCE equals the angle FAG.

Therefore on the given straight line AB, and at the point A on it, the rectilinear angle FAG has been constructed equal to the given rectilinear angle DCE.

Q.E.F.

In all there are ten circles and one line that must be drawn. The lines in the intermediate stages may be suppressed as usual since they’re only needed to verify the construction is correct.

Although it may appear that the triangles are to be in the same plane, that is not necessary. Indeed, the construction in this proposition is used to construct an angle in a different plane in proposition XI.31.