Euclid's Elements, Book XI, Definitions 14 through 17 (original) (raw)
Definition 14
When a semicircle with fixed diameter is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a sphere.
Definition 15
The axis of the sphere is the straight line which remains fixed and about which the semicircle is turned.
Definition 16
The center of the sphere is the same as that of the semicircle.
Definition 17
A diameter of the sphere is any straight line drawn through the center and terminated in both directions by the surface of the sphere.
Guide
There are alternative definitions for a sphere, but Euclid chose this one, perhaps, to be analogous to the definitions of cone in XI.Def.18 and cylinder in XI.Def.22. These are all defined as solids of revolution, that is, solids generated by rotating a plane figure around a straight line called the axis of revolution.
Another book would probably be required to develop the theory of spheres to the degree that Euclid developed the theory of circles in Book III, but that, apparently, was not his goal. The lack of propositions is so severe that it is not even shown that any two points on the surface of a sphere are equidistant from the center. (Any point on the surface of the sphere is a point on the circumference of one of the rotated semicircles, and all the points on any of these semicircles are equidistant from the center of the semicircles.)
| In the illustration at the right there is a semicircle ADB with center C and diameter AB in a plane. When the semicircle is revolved around AB, a sphere results. The sphere’s axis is AB, and its center is C. If E is any point on the sphere and F the antipodal point, then the line EF is a diameter of the sphere.There are very few propositions about spheres in the Elements. Proposition XII.17 allows a kind of approximation of spheres by polyhedra preliminary to proposition XII.18 on the ratio of volumes of spheres. Also, regular polyhedra are inscribed in spheres in Book XIII With so few propositions there are gaps in the proofs. For instance, in XII.17 it is claimed that the the intersection of a plane and a sphere is a circle, but a justification is lacking. | 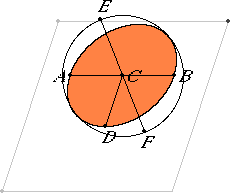 |
|---|