Attosecond electron wave packet interferometry (original) (raw)
The development of intense femtosecond lasers, capable of generating electric fields with strengths comparable to the Coulomb fields in atoms and molecules, has motivated extensive fundamental research in the past twenty years. Ionization and dissociation processes are increasingly well understood, and there have been important spin-offs, such as the development of coherent light sources in the extreme-ultraviolet (XUV) range by high-harmonic emission, in particular the generation of attosecond light pulses3,4. This research field is now beginning to make important contributions in other areas of science. For example, electrons ionized by a strong laser field have been used to probe molecular structure8 and molecular ground-state wavefunctions7. The new idea put forward in our work is to make use of interferences between continuum electron wave packets9 that are prepared by a sequence of attosecond laser pulses. This allows us to probe the dynamics of continuum electrons in an infrared (IR) laser field, to investigate the fundamental process of atomic photoionization and to characterize the electronic wave packet created in the process.
Our method closely resembles the traditional implementation of interferometry in optics, where a light wave E(x) is split into two replicas that travel different paths. The two replicas interfere when they are recombined, and from the interference pattern, |E(x)+E(x)e_i_ δ ϕ(x)|2∝1+cos_δ_ ϕ(x), the difference in the accumulated phase, δ ϕ(x), in the two paths can be extracted. The interference pattern in this case does not depend on the phase of the initial field, but on the optical path difference. In another implementation, the field itself can be characterized if a ‘shear’ δ x is added to one of the two replicas. If |E(x)|≈|E(x+δ x)| in the region of overlap,
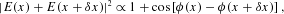
where φ(x) is the phase of E(x). In this case, information about the phase φ(x) can be retrieved. This technique has been applied extensively in optics, for the measurement of wavefronts2 when a spatial shear is induced, and for the determination of the spectral phase of ultrashort optical pulses1,5 when a spectral shear is induced.
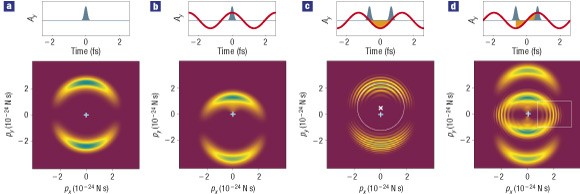
The principle of our measurement technique is illustrated, for helium, in Fig. 1. The photoelectron-momentum distribution obtained when a helium atom is ionized by a single attosecond pulse is shown in Fig. 1a. It is a ring-shaped distribution peaked along the direction of polarization of the electromagnetic field10, which here corresponds to the p y axis. When an IR field is present at the time of ionization, the momentum distribution is shifted by the amount of momentum transferred from the field to the continuum electron wave packet, as shown in Fig. 1b. Here, the infrared intensity is I_IR=3×1013 W cm−2 and the momentum transfer is equal to −_e A(τ)=1×10−24 N s, where A is the vector potential of the IR field, polarized in the same direction (y) as the XUV field, τ is the instant of ionization and e is the electron charge. This effect, which strongly depends on the initial timing11,12, has previously been used in attosecond metrology to determine the characteristics of single attosecond pulses13 as well as of the IR laser field14.
Figure 1: Principle of interferometry measurements.
The results of calculations carried out by solving the time-dependent Schrödinger equation in helium within the single active electron approximation. a, The momentum distribution of an electron ionized by a single 180-attosecond 46-eV pulse (shown schematically above the figure). The width of the ring is related to the attosecond pulse bandwidth (11 eV). The blue plus sign indicates the centre of the momentum distribution. In b, the distribution is displaced because the ionization occurs in the presence of an IR laser field that is linearly polarized along the y axis. The momentum shift is equal to −e A(τ), where A is the vector potential, indicated in red above the figure, and τ is the instant of ionization. In c,d, the photoionization is due to a sequence of two attosecond pulses separated by half the IR laser cycle, as shown schematically above the figure. In c, the ionization occurs at zeros of A(τ) and the interference structure depends on the difference in accumulated phase between the electron wave packets. It consists of circles, as shown by the white line, centred at (p _x_=0,p y_=−_e A(τ)/π). In d, the ionization occurs at a maximum or minimum of A(τ), and there is a momentum shear between the wave packets that affects the interference pattern in the region of overlap. The difference in accumulated phase between the two electron wave packets is proportional to the integral of the vector potential and is illustrated by the orange area at the top of c,d.
When the atom is ionized by two attosecond pulses separated by half the IR laser period, two electron wave packets are created which will interfere where their momentum distributions overlap. This is very similar to the traditional Young’s double-slit experiment, where the slits here are provided by the double-attosecond pulse excitation. When the electron wave packets are formed in the presence of an IR field at times when the vector potential is zero (Fig. 1c), they have approximately the same final momentum distributions but different accumulated phases due to the different amount of time spent in the dressed continuum. As explained in the Supplementary Information, using a semiclassical formulation15,16 for _δ_-pulses, the phase difference between the two interfering wave packets can be written as
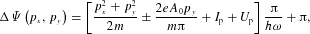
where m is the electron mass, ħ is the Planck constant divided by 2_p_, ω is the IR frequency, _I_p is the ionization energy, _A_0 is the amplitude of the vector potential, and _U_p=e_2_A_02/4_m is the ponderomotive energy. The total energy absorbed in the ionization process is 
. As shown in Fig. 1c, the interference pattern, obtained by solving the time-dependent Schrödinger equation in helium for 180-attosecond pulses, follows the semiclassical predictions (indicated by the white circle) remarkably well. This shows that neither the presence of the ion core, modifying the electron dynamics, nor the non-zero length of the attosecond pulses, significantly affect the interferogram, which consists of circles centred at (p _x_=0,p y_=±2_e _A_0/π). The sign depends on the direction (up or down) of the vector potential during the electron trajectory (in Fig. 1c, it is positive). The position of the centre of the circles can be used to determine the intensity of the IR field, and their radii allow us to cross-check the value of the ponderomotive shift _U_p (here equal to ≈1.8 eV). However, no information about the phase of the ‘initial’ continuum electron wave packet can be deduced. (‘Initial’ here and throughout the text refers to the wave packet created in the absence of an infrared laser field.)
When the attosecond pulses instead coincide with the maxima and minima of the vector potential (Fig. 1d), the momentum transfer from the IR field is maximal and opposite in direction for the two wave packets. The difference in accumulated phase between the two ionization events due to the interaction with the field is equal to zero in this case. The phase that governs the interference pattern can be written as (see the Supplementary Information)
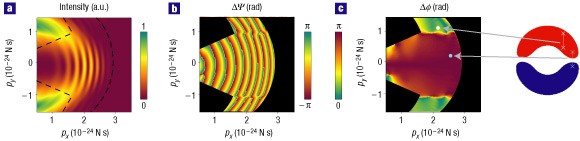
This allows us to determine the difference between the phases of the initial electron wave packet (φ) at two different positions in momentum space, in the region where the two final momentum distributions overlap. To illustrate this point, the interference pattern inside the white rectangle in Fig. 1d is analysed (Fig. 2a). The phase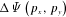 
can be extracted from the interferometric measurement by using a Fourier-transform method17, allowing us to extract the phase of the oscillating fringes at different points in momentum space using straightforward mathematical manipulations. The most remarkable feature exhibited in the phase of the fringes, represented in Fig. 2b, is the abrupt change of phase by π at p y_≈±_e A_0, which corresponds to the momentum shift induced by the IR field. In Fig. 2c, we extract 
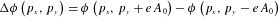. Δ_φ is approximately constant, equal to π for |p y|≤|e _A_0| and 0 elsewhere. This indicates that the phases of the initial wave packet at 
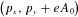and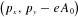 
differ by 0 or π, depending on whether the momenta are located on the same side or on opposite sides of the p _y_=0 plane (see the schematic diagram) respectively. This result is expected for photoionization of the helium ground state, and leads to a _p_-continuum wavefunction which changes sign across the p _y_=0 plane.
Figure 2: Analysis of the interferogram in helium.
Analysis of a, the theoretical results obtained in helium when ionization occurs at times such that the vector potential is maximum. The dotted lines indicate the region of overlap where the Fourier analysis is carried out. b, Δ_Ψ_. c, Δ_φ_. The sampling is illustrated by a schematic diagram. A phase difference of 0 is obtained when the interference measurement involves two momentum components lying on the same side of the p _y_=0 plane, and π when the two momentum components lie on opposite sides.
More generally, our interferometric technique should allow us to visualize any phase variation of the initial continuum wave packet that is in the region of overlap of the final momentum wave packets. However, in this proof-of-principle example, the phase retrieval is somewhat limited. To improve our technique towards a more-complete phase reconstruction, we must (1) use a momentum shear in two perpendicular directions, as in lateral-shearing interferometry2, to enable the reconstruction of the phase in two dimensions; (2) use a combination of small momentum shear to obtain a larger region of overlap and to better sample the momentum distribution, and large momentum shear to probe the difference of phase between the different parts of the electron wave packet.
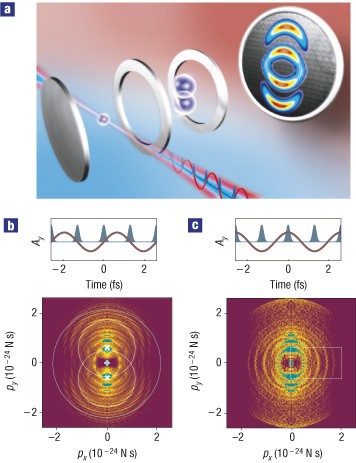
In our experiment (Fig. 3a), a train of attosecond pulses, generated through high-order harmonic generation in argon18, is used to ionize argon, resulting in a train of electron wave packets with 200±30-attosecond duration, a central kinetic energy of 11 eV and a bandwidth of 11 eV. The pulse duration of the electron wave packets are determined by a RABITT (reconstruction of attosecond beating by interference of two-photon transition) analysis3. The IR field, which accelerates the continuum electron wave packets, is a fraction of the laser beam used to generate the harmonics. The key results of this work are presented in Fig. 3b,c, where two-dimensional cuts through the three-dimensional momentum distributions19,20 obtained in argon using a velocity-map imaging technique21,22 are presented for two delays between the XUV and IR fields. The distributions are more complex than those presented in helium. This is partly because of the properties of our attosecond pulses, which are generated as a train, containing more than two pulses. An additional interference structure is superposed on that described previously, which consists of circles centred at the origin, simply expressing energy conservation. This interference effect is very similar to that leading to electron peaks separated by ħ_ω_, when atoms are ionized by intense laser pulses23,24. In addition, argon has three different initial states, 3_p_,_m_=0,±1 and, for one-photon absorption, several possible final states with s or d symmetry. The interferences discussed above are, however, clearly visible. Figure 3b shows a complex interference structure, with three sets of circles that have different origins: (p _x_=0,p _y_=0) (large circle), (p _x_=0,p y_=±2_e _A_0/π) (small circles). An analysis of this interference pattern allows us to determine the IR intensity to be _I_IR=2.5×1013 W cm−2, a value that agrees well with that estimated in our experimental conditions.
Figure 3: Experimental method and results.
a, The principle of our experiment. An IR laser field and a train of attosecond pulses are focused into the sensitive region of a velocity-map imaging electron spectrometer, where they intersect a beam of Ar atoms. The polarizations of the IR and XUV fields are parallel to each other, and are perpendicular to the detection axis of the velocity-map imaging electron spectrometer. Electrons created in the interaction region are extracted using a high voltage d.c. electric field and are accelerated towards an imaging microchannel plate assembly, then towards a phosphor screen and a charge-coupled device camera system that record the positions of the electron impacts on the detector. The three-dimensional velocity distribution at a given delay between the XUV and IR fields is recovered from the measured projection using an iterative procedure. b,c, Experimental data at two different relative delays between the attosecond pulses and the IR laser field. The attosecond pulses coincide with the zeros of the vector potential in b and with its maxima and minima in c. Both cases show complex interference patterns. In b, the fringes resulting from the interference of consecutive electron wave packets are circles centred on the p y axis, as shown by the small circles centred on the white crosses. Those owing to interferences between electron wave packets separated by one IR cycle are circles centred at the origin, as shown by the large circle centred on the blue plus sign. In c, the most remarkable effect is the pronounced interference structure crossing the p x axis, as shown inside the white rectangle. The upper and lower parts of the momentum distribution obtained at the maximum energy transfer delay are not shown in this figure, where the highest electron energy is ∼25 eV.
The complexity of the interference pattern obtained in Fig. 3c, combined with experimental statistical noise, prevent us from carrying out an analysis as in Fig. 2, except close to the p x axis. This is equivalent to discussing the behaviour of the interference pattern in the region outlined by the white rectangle. In Fig. 4a, we compare these interference fringes with theoretical results obtained in argon for similar conditions as in the experiment (a train of Fourier-transform-limited 190-attosecond pulses, centred at 27-eV energy, and an IR intensity equal to I_IR=2.5×1013 W cm−2). In this case, the momentum distribution measured is the incoherent sum of two contributions, originating from the 3_p _m_=0 and the _m_=±1 states. Interestingly, because the corresponding ground-state wavefunctions have different symmetry properties (antisymmetric and symmetric relative to p _y_=0, respectively), the interference fringes will be shifted by π along the p x axis. Theoretical results obtained by separating the two contributions (Fig. 4b) clearly show this phase difference. Our experimental (and theoretical) results show that in this region, photoionization of argon is dominated by the contributions from the _m_=±1 states.
Figure 4: Comparison between theory and experiment in argon.
a, Comparison of experimental (top) and theoretical (bottom) results obtained in argon in similar conditions, in the region of overlap of the two electron wave packet final momenta distributions. b, The theoretical results for only one ground-state wavefunction, _m_=0 (top) and _m_=1 (bottom). The blue and white dots indicate the position of the maxima and minima of the interference fringes on the p x axis. Together with the dashed lines, they help to show that the interference patterns are inverted. For two momenta lying on opposite sides of the p _y_=0 plane, the phase difference of the intial electron wave packet is 0 for _m_=0 (top) and π for _m_=1 (bottom).
By increasing or decreasing the laser intensity, by varying the delay while taking the added accumulated phase due to the interaction with the IR field into account, and by using different polarization states for the XUV and IR light fields combined with a polarization-independent detection technique21, more information could be obtained in a larger region in momentum space. This opens exciting possibilities for investigating the continuum and ground-state electronic wavefunctions of complex, chemically relevant molecules and also for following the dynamics of time-dependent superposition of states.