Spine-neck geometry determines NMDA receptor-dependent Ca2+ signaling in dendrites - PubMed (original) (raw)

Comparative Study

Spine-neck geometry determines NMDA receptor-dependent Ca2+ signaling in dendrites

Jun Noguchi et al. Neuron. 2005.

Abstract

Increases in cytosolic Ca2+ concentration ([Ca2+]i) mediated by NMDA-sensitive glutamate receptors (NMDARs) are important for synaptic plasticity. We studied a wide variety of dendritic spines on rat CA1 pyramidal neurons in acute hippocampal slices. Two-photon uncaging and Ca2+ imaging revealed that NMDAR-mediated currents increased with spine-head volume and that even the smallest spines contained a significant number of NMDARs. The fate of Ca2+ that entered spine heads through NMDARs was governed by the shape (length and radius) of the spine neck. Larger spines had necks that permitted greater efflux of Ca2+ into the dendritic shaft, whereas smaller spines manifested a larger increase in [Ca2+]i within the spine compartment as a result of a smaller Ca2+ flux through the neck. Spine-neck geometry is thus an important determinant of spine Ca2+ signaling, allowing small spines to be the preferential sites for isolated induction of long-term potentiation.

PubMed Disclaimer

Figures

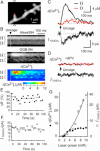
Figure 1

Figure 1. NMDAR-Mediated Ca2+ Signaling in Single Spines of CA1 Pyramidal Neurons in Response to Two-Photon Uncaging of MNI-Glutamate

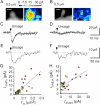
(A) A three-dimensionally stacked two-photon fluorescence image of a region of a dendrite labeled with Alexa Fluor 594. Arrowheads indicate the line of laser scanning at 830 nm for fluorescence imaging, and the red dot represents the point of uncaging of MNI-glutamate. (B) Line-scan images of the spine shown in (A) for Alexa Fluor 594 (top), OGB-5N (middle), and Δ[Ca2+]i obtained from the ratio of OGB-5N to Alexa Fluor 594 (bottom). The time of uncaging of MNI-glutamate is indicated by the arrowhead. The magnitude of Δ[Ca2+]i is pseudocolor coded as indicated. (C) Δ[Ca2+]i in the spine head (“H”) and at the base of the spine (“D”) shown in (A) as well as NMDAR-mediated current (INMDA) recorded in the whole-cell patch-clamp mode. Regions of averaging of fluorescence to estimate Δ[Ca2+]i are indicated as “H” and “D” in (B). (D) Elimination by APV (100 μM) of the [Ca2+]i and INMDA responses to uncaging of MNI-glutamate in the neuron shown in (A). (E and F) Stability of the maximal increase in the fluorescence ratio (ΔR) between OGB-5N and Alexa Fluor 594(E) and of the maximal INMDA (F) in a spine head subjected to uncaging of MNI-glutamate for >20 times. Horizontal lines indicate averages of three consecutive amplitudes. (G) Dependence of Δ[Ca2+]i and NMDAR-mediated current on the laser power for uncaging in a spine head with a volume of 0.12 μm3.

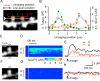
Figure 2

Figure 2. Spatial Spread of NMDAR Activation and of Δ[Ca2+]i in CA1 Pyramidal Neurons

(A) A stacked Alexa Fluor 594 image of a dendritic region. The red scale indicates the line along which uncaging of MNI-glutamate was sequentially induced, and the white line represents the scanning line for acquisition of fluorescence images. (B) NMDAR-mediated current as well as Δ[Ca2+]i in the left (green circles) and right (orange circles) spines elicited by uncaging of MNI-glutamate at points along the red line in (A). (C) Stacked Alexa Fluor 594 image of a spine for which line scanning was applied at the line indicated by the arrowheads and uncaging of MNI-glutamate was induced at the red spot. (D and E) Line-scan images for Δ[Ca2+]i (D) and averaged traces of Δ[Ca2+]i (E) obtained from the spine shown in (C) during uncaging of MNI-glutamate at the red spot. Regions of averaging of fluorescence for Δ[Ca2+]i are indicated as “H” and “D” in (D). (F–H) Panels corresponding to (C)–(E), respectively, for uncaging of MNI-glutamate at the position on the dendritic shaft indicated by the red dot in (F).

Figure 3

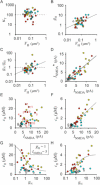
Figure 3. Dependence of NMDAR-Mediated Current and Ca2+ Signaling on Spine-Head Volume

(A) Stacked Alexa Fluor 594 image of a dendritic region in which uncaging of MNI-glutamate was induced at the numbered spines and line-scan imaging was performed along the axis of each spine. Original xy images are shown in Figure S5. (B–D) VH dependence of NMDAR-mediated current (INMDA) (B) as well as of Δ[Ca2+]i in the spine head (cH) (C) and in the dendritic shaft at the base of the spine (cD) (D). Straight lines in (B) are linear regression lines. Uncaging was induced at the spines (red circles) indicated in (A) and at spines in three other dendrites (yellow, blue, and white circles). The amplitude of currents was measured at their peak, and Δ[Ca2+]i was measured at the time of the maximal value in spine heads. (E) VH dependence of the coupling ratio (cR = cD/cH) for each spine. (F and G) VH dependence of Ca2+ conductance of the spine neck (gN) and its double logarithmic plot, respectively. Smooth curves in (C)–(G) were drawn according to (24), (25), (26), (27) and (28) with four different sets of dendritic parameters as described in “Spine Ca2+ Model.”

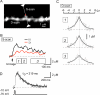
Figure 4

Figure 4. Spread of Ca2+ along the Axis of a Dendritic Shaft

(A) Stacked Alexa Fluor 594 fluorescence image of a dendritic region subjected to line scanning along both the axis of a spine (S-scan) and that of the parent dendritic shaft (D-scan). Uncaging of MNI-glutamate was performed at the position indicated by the red dot. (B) Averaged Δ[Ca2+]i in the spine head (H) and at the base of the spine (D). (C) Spatial gradient of Δ[Ca2+]i at three different time periods (boxes 1, 2, 3) depicted in (B). Smooth curves are exponential functions with length constants of 1.6 μm, 1.9 μm, and 2.4 μm for the periods 1, 2, and 3, respectively. (D) Time course of Δ[Ca2+]i in a dendritic shaft whose soma was depolarized to −10 mV for 40 ms. The decay of Δ[Ca2+]i was fitted with an exponential curve with a time constant (τD) of 219 ms.

Figure 5

Figure 5. Spine-Neck Geometries

(A) Stacked Alexa Fluor 594 fluorescence images of dendritic spines with different cR values. Necks appear to be shorter and thicker for spines with a larger cR. Two values of spine-neck Ca2+ conductance, gN and gN*, were estimated from cR and from fluorescence analysis as described below, respectively. (B) Stacked Alexa Fluor 594 fluorescence image of a spine subjected to analysis of neck geometry. (C) Fluorescence profile along the dashed line for the spine shown in (B). Four regions were selected for fluorescence measurement. The neck region (1) is flanked by the edges of the spine head and dendritic shaft, which are determined by curve fitting (see Experimental Procedures). For those stubby spines whose neck structures were not well resolved, we assumed lN as 0.05 μm and obtained rN at the base of the spine. Regions of background fluorescence for the spine head (2) and the dendritic shaft (3) are placed at the predicted edge of a spherical spine and cylindrical shaft. A region of nonspecific background fluorescence (4) was positioned between 2 and 5 μm from the shaft. (D) Relation between gN* and gN. Data obtained from the same dendrites are represented by the same colors as in Figure 3. We estimated gN* from neck fluorescence according to Equation 4 and assuming Dapp = 12 μm2 s−1 (see Experimental Procedures). (E) Double logarithmic plot of the VH dependence of gN*. Straight lines in (D) and (E) are linear regression lines. Triangles in (D) and (E) indicate those stubby spines whose values of gN* were obtained by assuming lN = 0.05 μm.

Figure 6

Figure 6. Determinants of Spine Ca2+ Signaling

(A and B) VH dependence of κT and gH, respectively, among spines. (C) VH dependence of the gN/gH ratio. (D) Predicted values of NMDAR-mediated current (INMDA*) from Equation 8 plotted against actual INMDA for each spine. Values of INMDA were obtained at the peak of spine [Ca2+]i as shown in Figure 8E. (E and F) INMDA dependence of cH and cD, respectively, among spines. Straight lines in (A)–(D) and (F) are linear regression lines. (G and H) gN dependence of cH and cD, respectively, among spines. Data obtained from the same dendrites are represented by the same colors as those in Figure 3. The smooth lines in (G) and (H) were obtained from (21) and (22), respectively, assuming gH = 1 μm3 s−1, gD = 7 μm3 s−1, INMDA = 5 pA, κT = 102, and gN = 250 VH2.

Figure 7

Figure 7. Functional Expression of AMPARs and NMDARs in the Same Spines

(A and B) Fluorescence images (left) of spines and their maps of AMPAR-mediated current (right). Current amplitude is pseudocolor coded as indicated. (C and D) Maximal AMPAR-mediated currents in the spines shown in (A) and (B), respectively. (E and F) NMDAR-mediated currents evoked by uncaging of MNI-glutamate at the tips of the spines show in (A) and (B), respectively. (G) VH dependence of the maximal AMPAR-mediated currents for 31 spines on four dendrites represented by different colors. (H) Relation between the maximal amplitudes of NMDAR-mediated and AMPAR-mediated currents recorded from the same spines. Data in (G) and (H) were obtained from preparations different from those shown in Figure 3.

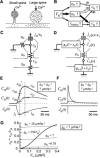
Figure 8

Figure 8. Spine Ca2+ Signals

(A) NMDAR-mediated influx of Ca2+ (dots) in small and large spines. The narrow neck of small spines results in larger and more confined increases in [Ca2+]i in the spine head, allowing induction of LTP at the level of the single spine. The thick neck of large spines gives rise to smaller increases in spine [Ca2+]i and greater outflow of Ca2+ into the dendritic shaft. (B) Diagram showing the relative effects of spine-head volume (VH) on spine-neck Ca2+ conductance (gN), spine-head Ca2+ conductance (gH), and INMDA, as well as of the latter parameters on spine-head [Ca2+]i (cH) and [Ca2+]i in the dendritic shaft adjacent to the spine (cD). (C) Stationary model of Ca2+ signaling in a spine, where gD, jH, and κ T represent Ca2+ conductance of the dendritic shaft, Ca2+ influx, and the total Ca2+ binding ratio, respectively. (D) Time-dependent model, where τH and τD represent the time constants of [Ca2+]i in the spine head and dendritic shaft, respectively.ĴH(s), ĈH(s), and ĈD(s) are the Laplace transforms of JH (t), CH (t), and CD (t), respectively, which represent the time courses of Ca2+ influx and of [Ca2+]i in the spine head and in the dendritic shaft adjacent to the spine, respectively. (E and F) Simulation of CH(t) and CD(t) based on the model in (D) and (29) and (30), where JH(t) is either EPSC-like (E) or impulsive (F), and gN, gD , and gH are all set to 7.0 μm3 s−1 . As indicated in (E), cH, cD, and jH are obtained at the time when CH(t) is maximal, and cIH, cIH′, cID, and jI are obtained at the time when JH(t) is maximal. (G) VH dependence of cR (equal to cD/cH) in the time-dependent model in (D), where cD and cH are obtained as indicated in (E). The value of gN is either fixed (thin lines) or VH dependent (thick line). The values of gD, τD, τH are set at 7 μm3 s−1 , 0.2 s, 0.05 s, respectively, and gH is altered according to VH/τH.

Similar articles

Cited by

References

    1. Allbritton NL, Meyer T, Stryer L. Range of messenger action of calcium ion and inositol 1,4,5-trisphosphate. Science. 1992;258:1812–1815. - PubMed
    1. Bonhoeffer T, Yuste R. Spine motility. Phenomenology, mechanisms, and function. Neuron. 2002;35:1019–1027. - PubMed
    1. Coss RG, Perkel DH. The function of dendritic spines: a review of theoretical issues. Behav. Neural Biol. 1985;44:151–185. - PubMed
    1. Crick F. Do dendritic spines twitch? Trends Neurosci. 1982;5:44–46.
    1. DiGregorio DA, Vergara JL. Localized detection of action potential-induced presynaptic calcium transients at a Xenopus neuromuscular junction. J. Physiol. 1997;505:585–592. - PMC - PubMed

Publication types

MeSH terms

Substances

LinkOut - more resources