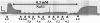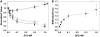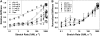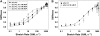The effects of force inhibition by sodium vanadate on cross-bridge binding, force redevelopment, and Ca2+ activation in cardiac muscle - PubMed (original) (raw)
The effects of force inhibition by sodium vanadate on cross-bridge binding, force redevelopment, and Ca2+ activation in cardiac muscle
D A Martyn et al. Biophys J. 2007.
Abstract
Strongly bound, force-generating myosin cross-bridges play an important role as allosteric activators of cardiac thin filaments. Sodium vanadate (Vi) is a phosphate analog that inhibits force by preventing cross-bridge transition into force-producing states. This study characterizes the mechanical state of cross-bridges with bound Vi as a tool to examine the contribution of cross-bridges to cardiac contractile activation. The K(i) of force inhibition by Vi was approximately 40 microM. Sinusoidal stiffness was inhibited with Vi, although to a lesser extent than force. We used chord stiffness measurements to monitor Vi-induced changes in cross-bridge attachment/detachment kinetics at saturating [Ca(2+)]. Vi decreased chord stiffness at the fastest rates of stretch, whereas at slow rates chord stiffness actually increased. This suggests a shift in cross-bridge population toward low force states with very slow attachment/detachment kinetics. Low angle x-ray diffraction measurements indicate that with Vi cross-bridge mass shifted away from thin filaments, implying decreased cross-bridge/thin filament interaction. The combined x-ray and mechanical data suggest at least two cross-bridge populations with Vi; one characteristic of normal cycling cross-bridges, and a population of weak-binding cross-bridges with bound Vi and slow attachment/detachment kinetics. The Ca(2+) sensitivity of force (pCa(50)) and force redevelopment kinetics (k(TR)) were measured to study the effects of Vi on contractile activation. When maximal force was inhibited by 40% with Vi pCa(50) decreased, but greater force inhibition at higher [Vi] did not further alter pCa(50). In contrast, the Ca(2+) sensitivity of k(TR) was unaffected by Vi. Interestingly, when force was inhibited by Vi k(TR) increased at submaximal levels of Ca(2+)-activated force. Additionally, k(TR) is faster at saturating Ca(2+) at [Vi] that inhibit force by > approximately 70%. The effects of Vi on k(TR) imply that k(TR) is determined not only by the intrinsic properties of the cross-bridge cycle, but also by cross-bridge contribution to thin filament activation.
Figures
FIGURE 1
Force was maximally activated at pCa 4.5. After steady-state force was reached, force was inhibited with 0.3 mM Vi, followed by relaxation in pCa 9.2. Force was subsequently measured at various submaximal [Ca2+] (indicated below the force trace), each with 0.3 mM Vi. The trabeculae was again relaxed. The relaxed trabecula was finally activated in pCa 4.5 to allow force recovery (typically >80%). Initial sarcomere length was 2.3 _μ_m and diameter was 125 _μ_M. The calibration bars represent 30 mg (vertical) and 1 min (horizontal).
FIGURE 2
Representative traces of force redevelopment following the rapid release/restretch protocol used to determine _k_TR are shown; _k_TR was determined during steady force at each [Ca2+] and [Vi]. Force was normalized to the maximum for each condition and is shown for preinhibition controls, following force inhibition by 1.0 mM Vi and subsequent to force recovery from Vi inhibition. The time course of force development in controls and following recovery from inhibition nearly superimpose. The corresponding unnormalized traces are shown in the inset; _k_TR was 15.9 s−1 in pC 4.5 controls, 23 s−1 with 1.0 mM Vi and 14.9 s−1 following recovery from inhibition in pCa 4.5.
FIGURE 3
The dependence of force on [Vi] with maximal activating [Ca2+] (pCa 4.5) at SL = 2.3 (•) and 2.0 (○) _μ_m, along with sinusoidal stiffness (▵) at 2.0 _μ_m initial SL, is illustrated in A. Also shown in A is the dependence of the maximal rate of isometric force redevelopment (_k_TR; ▾) on [Vi] at SL = 2.0 _μ_m. At pCa 4.5 with 1.0 mM Vi, _k_TR (▾*) was significantly faster than control (P < 0.05). All variables in panel A have been normalized to the corresponding values obtained at pCa 4.5 without Vi; data were obtained from seven trabeculae. The force and stiffness data in A are replotted in B to illustrate Vi effects on the stiffness/force ratio at pCa 4.5 and 2.0 _μ_m SL.
FIGURE 4
Skinned trabeculae were stretched at constant amplitude (0.5 %ML) at varying rates and the resulting changes in stiffness were measured at various [Vi], as indicated in the inset. In A the results were compared to maximum Ca2+ activation (no Vi; ○) and rigor (•). Vi reduced stiffness at all rates of stretch above ∼10 %ML s−1, whereas below this rate stiffness increased. In A data are normalized to the maximum stiffness at high stretch rates in uninhibited controls. Chord stiffness in B is expressed relative to the maximum stiffness value at high rates of stretch for each [Vi]. The data in B emphasize the peak in chord stiffness at slower rates of stretch, implying the presence of a cross-bridge population with slowed attachment/detachment kinetics. The curves in panels A and B were obtained by nonlinear fitting of the data with Peak-Fit (SPSS, Chicago, IL).
FIGURE 5
The equatorial x-ray reflection intensity ratio (_I_1,1/_I_1,0; Fig. 5 A) and myofilament lattice spacing (_D_1,0; Fig. 5 B) was determined at various [Ca2+] from pCa 9.2 to 4.5 in the absence (solid symbols; n = 6 trabeculae) and presence (open symbols; n = 4 trabeculae) of 1.0 mM Vi. _I_1,1/_I_1,0 (A) and _D_1,0 (B) for rigor conditions (▪; n = 4) are included for comparison.
FIGURE 6
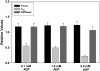
The effects 0.1, 1.0, and 5.0 mM ADP on maximum Ca2+-activated force (black bars), _k_TR (light gray bar), and sinusoidal stiffness (dark gray bar) are illustrated. Data were obtained from six trabeculae. Values are expressed relative to those obtained with no added ADP.
FIGURE 7
The influence of increasing [ADP] and 30 mM Pi on chord stiffness measurements is illustrated in panels A and B, respectively. Data were obtained from six trabeculae in A and three trabeculae in B. In panel A data were obtained at pCa 4.5 with 0.1 mM (○; heavy dashed line), 1.0 mM (▾; dotted line) and 5.0 mM (▵; dashed-dot-dot) ADP. Respective control measurements with no ADP or Pi added to solutions are shown in A and B (•; solid lines). Chord stiffness at each rate of stretch is normalized to the maximal level of stiffness at high rates of stretch for each condition. Initial SL was 2.0 _μ_m.
FIGURE 8
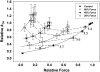
The Ca2+ dependence of force (A) and _k_TR (B) are shown at different [Vi]. Force in panel A and _k_TR in panel B are normalized to the maximum for each condition. Data were pooled from fibers that produced ∼60% (○; dashed line), ∼40% (▾; dashed-dot line), and ∼20% (▿; dotted line) of maximum Ca2+-activated force in control fibers. The force data in panel A were fit with the Hill equation and pCa50 and _n_H are included in Table 1, along with corresponding values of _F_max and maximal _k_TR. The Hill fit parameters for control _k_TR-pCa data in panel B were 5.62 ± 0.01 and 3.0 ± 0.14, for pCa50 and _n_H, respectively.
FIGURE 9
The data in Fig. 8 are replotted as _k_TR versus isometric force (relative to pCa 4.5 in controls), to emphasize the activation dependence of _k_TR in skinned cardiac trabeculae at increasing levels of force inhibition with Vi. Data obtained at a given [Ca2+] is connected by dashed lines, with the pCa being given at the right.
Figure
Similar articles
- Cooperative cross-bridge activation of thin filaments contributes to the Frank-Starling mechanism in cardiac muscle.
Smith L, Tainter C, Regnier M, Martyn DA. Smith L, et al. Biophys J. 2009 May 6;96(9):3692-702. doi: 10.1016/j.bpj.2009.02.018. Biophys J. 2009. PMID: 19413974 Free PMC article. - Cross-bridge versus thin filament contributions to the level and rate of force development in cardiac muscle.
Regnier M, Martin H, Barsotti RJ, Rivera AJ, Martyn DA, Clemmens E. Regnier M, et al. Biophys J. 2004 Sep;87(3):1815-24. doi: 10.1529/biophysj.103.039123. Biophys J. 2004. PMID: 15345560 Free PMC article. - Cooperative mechanisms in the activation dependence of the rate of force development in rabbit skinned skeletal muscle fibers.
Fitzsimons DP, Patel JR, Campbell KS, Moss RL. Fitzsimons DP, et al. J Gen Physiol. 2001 Feb;117(2):133-48. doi: 10.1085/jgp.117.2.133. J Gen Physiol. 2001. PMID: 11158166 Free PMC article. - Regulation of contraction in striated muscle.
Gordon AM, Homsher E, Regnier M. Gordon AM, et al. Physiol Rev. 2000 Apr;80(2):853-924. doi: 10.1152/physrev.2000.80.2.853. Physiol Rev. 2000. PMID: 10747208 Review. - Characterization of cross-bridge elasticity and kinetics of cross-bridge cycling during force development in single smooth muscle cells.
Warshaw DM, Rees DD, Fay FS. Warshaw DM, et al. J Gen Physiol. 1988 Jun;91(6):761-79. doi: 10.1085/jgp.91.6.761. J Gen Physiol. 1988. PMID: 3047311 Free PMC article. Review.
Cited by
- The force of the myosin motor sets cooperativity in thin filament activation of skeletal muscles.
Caremani M, Marcello M, Morotti I, Pertici I, Squarci C, Reconditi M, Bianco P, Piazzesi G, Lombardi V, Linari M. Caremani M, et al. Commun Biol. 2022 Nov 18;5(1):1266. doi: 10.1038/s42003-022-04184-0. Commun Biol. 2022. PMID: 36400920 Free PMC article. - Role of cardiac troponin I carboxy terminal mobile domain and linker sequence in regulating cardiac contraction.
Meyer NL, Chase PB. Meyer NL, et al. Arch Biochem Biophys. 2016 Jul 1;601:80-7. doi: 10.1016/j.abb.2016.03.010. Epub 2016 Mar 10. Arch Biochem Biophys. 2016. PMID: 26971468 Free PMC article. - Sarcomere length dependent effects on the interaction between cTnC and cTnI in skinned papillary muscle strips.
Li KL, Ghashghaee NB, Solaro RJ, Dong W. Li KL, et al. Arch Biochem Biophys. 2016 Jul 1;601:69-79. doi: 10.1016/j.abb.2016.02.030. Epub 2016 Mar 2. Arch Biochem Biophys. 2016. PMID: 26944554 Free PMC article. - Synergistic role of ADP and Ca(2+) in diastolic myocardial stiffness.
Sequeira V, Najafi A, McConnell M, Fowler ED, Bollen IA, Wüst RC, dos Remedios C, Helmes M, White E, Stienen GJ, Tardiff J, Kuster DW, van der Velden J. Sequeira V, et al. J Physiol. 2015 Sep 1;593(17):3899-916. doi: 10.1113/JP270354. Epub 2015 Jul 14. J Physiol. 2015. PMID: 26096258 Free PMC article. - In situ time-resolved FRET reveals effects of sarcomere length on cardiac thin-filament activation.
Li KL, Rieck D, Solaro RJ, Dong W. Li KL, et al. Biophys J. 2014 Aug 5;107(3):682-693. doi: 10.1016/j.bpj.2014.05.044. Biophys J. 2014. PMID: 25099807 Free PMC article.
References
- Gordon, A. M., E. Homsher, and M. Regnier. 2000. Regulation of contraction in striated muscle. Physiol. Rev. 80:853–924. - PubMed
- Pirani, A., C. Xu, V. Hatch, R. Craig, L. S. Tobacman, and W. Lehman. 2005. Single particle analysis of relaxed and activated muscle thin filaments. J. Mol. Biol. 346:761–772. - PubMed
- Lehrer, S. S., and M. A. Geeves. 1998. The muscle thin filament as a classical cooperative/allosteric regulatory system. J. Mol. Biol. 277:1081–1089. - PubMed
- Geeves, M. A., and K. C. Holmes. 1999. Structural mechanism of muscle contraction. Annu. Rev. Biochem. 68:687–728. - PubMed
- Hofmann, P. A., and F. Fuchs. 1987. Evidence for a force-dependent component of calcium binding to cardiac troponin C. Am. J. Physiol. 253:C541–C546. - PubMed
Publication types
MeSH terms
Substances
Grants and funding
- T32 EB001650/EB/NIBIB NIH HHS/United States
- R01 HL067071/HL/NHLBI NIH HHS/United States
- ImNIH/Intramural NIH HHS/United States
- R01 HL061683/HL/NHLBI NIH HHS/United States
- R01 HL065497/HL/NHLBI NIH HHS/United States
- HL 67071/HL/NHLBI NIH HHS/United States
- HL61683/HL/NHLBI NIH HHS/United States
LinkOut - more resources
Full Text Sources
Miscellaneous