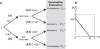Can influenza epidemics be prevented by voluntary vaccination? - PubMed (original) (raw)

Can influenza epidemics be prevented by voluntary vaccination?

Raffaele Vardavas et al. PLoS Comput Biol. 2007 May.

Abstract

Previous modeling studies have identified the vaccination coverage level necessary for preventing influenza epidemics, but have not shown whether this critical coverage can be reached. Here we use computational modeling to determine, for the first time, whether the critical coverage for influenza can be achieved by voluntary vaccination. We construct a novel individual-level model of human cognition and behavior; individuals are characterized by two biological attributes (memory and adaptability) that they use when making vaccination decisions. We couple this model with a population-level model of influenza that includes vaccination dynamics. The coupled models allow individual-level decisions to influence influenza epidemiology and, conversely, influenza epidemiology to influence individual-level decisions. By including the effects of adaptive decision-making within an epidemic model, we can reproduce two essential characteristics of influenza epidemiology: annual variation in epidemic severity and sporadic occurrence of severe epidemics. We suggest that individual-level adaptive decision-making may be an important (previously overlooked) causal factor in driving influenza epidemiology. We find that severe epidemics cannot be prevented unless vaccination programs offer incentives. Frequency of severe epidemics could be reduced if programs provide, as an incentive to be vaccinated, several years of free vaccines to individuals who pay for one year of vaccination. Magnitude of epidemic amelioration will be determined by the number of years of free vaccination, an individuals' adaptability in decision-making, and their memory. This type of incentive program could control epidemics if individuals are very adaptable and have long-term memories. However, incentive-based programs that provide free vaccination for families could increase the frequency of severe epidemics. We conclude that incentive-based vaccination programs are necessary to control influenza, but some may be detrimental. Surprisingly, we find that individuals' memories and flexibility in adaptive decision-making can be extremely important factors in determining the success of influenza vaccination programs. Finally, we discuss the implication of our results for controlling pandemics.

PubMed Disclaimer

Conflict of interest statement

Competing interests. The authors have declared that no competing interests exist.

Figures

Figure 1

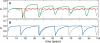
Figure 1. Results of the Model without Incentives

The vaccination coverage dynamic has a memory parameter s = 0.7, an adaptability parameter ε = 1, a critical vaccination coverage level p c = 0.6 (dashed line), and a probability q(0) = 0.8 of getting infected if no one participates in the voluntary vaccination program. (A) Dynamics of yearly coverage (p) for a population of N = 105 individuals (black data), and the corresponding dynamics of the prevalence (red data). The dynamics of the yearly coverage is approximately cyclic: as p approaches pc from below, it eventually fluctuates above pc and then abruptly drops below pc. (B) The probability that individual i decides to be vaccinated in season n is w (i) n. The figure shows w (i) n versus time for two individuals in the population. In contrast to the simple dynamics of the coverage, individuals go through complex vaccination decision behavior. (C) Normalized distributions ρ(w (i)) versus w (i) n for a population with N = 107 for improved accuracy. The distribution when the coverage fluctuates above pc is shown by the black data, and the distribution in the successive year when the coverage abruptly drops below pc is shown by the blue data. Individuals tend to strongly segregate into two groups. The individuals in one group are highly unlikely to get vaccinated the next season. The black data show that the individuals in the other group are highly likely to get vaccinated (i.e., w = 1). The blue data show that the individuals in the second group are less likely to get vaccinated than previously (i.e., given that no epidemic occurred in the previous season, w = s).

Figure 2

Figure 2. Vaccine Coverage for Different Public Health Programs.

The dynamics of the vaccination coverage p is calculated for N = 105 individuals using a memory parameter s = 0.7, an adaptability parameter ε = 1, a critical vaccination coverage level pc = 0.6, and a probability q(0) = 0.8 of getting infected when p = 0. (A) Individuals who pay for one vaccination are then given y = 3 (red data) and y = 15 (green data) free years of vaccination; the vaccine coverage when individuals are given no incentive to get vaccinated (i.e., no free vaccine) is shown by the black data for comparison. (B) The head of the family makes the decision as to whether or not their family gets vaccinated. The vaccine coverage when the family size is eight (C = 8) is shown by the blue data; the vaccine coverage when each individual makes voluntary vaccination decisions independently (rather than as a family) is shown by the black data for comparison. Similar results were obtained for family sizes of two and four.

Figure 3

Figure 3. The Structure of the Average Prevalence Level (i Panels) and the Average Coverage Level (ii Panels) in the Parameter Space ɛ − s

(A) Individuals receive no incentives (i.e., no free vaccines)—i.e., y = 0 (gray data). (B) Individuals pay for one vaccine and are then given y = 3 years of vaccination (red data). (C) Individuals pay for one vaccine and are then given y = 15 years of vaccination (green data).

Figure 4

Figure 4. Diagrammatic Description of the Adaptive Decision-Making Model

(A) Diagram illustrating the evaluation tree. An individual who decides to get vaccinated (branch (a)) will base their decision on whether there was an influenza epidemic that season. If the coverage p was equal to or greater than the critical coverage pc (i.e., p ≥ pc) (branch (a1)), they will conclude that their decision to get vaccinated that season was unnecessary to avoid infection. Otherwise, if the coverage was lower than the critical coverage (i.e., p < pc) (branch (a2)), they will conclude that their decision was beneficial for avoiding infection. An individual who decides not to get vaccinated that season (branch (b)) will base their decision on whether they were infected. If they did get infected (branch (b1)) they will conclude that their decision to not get vaccinated was detrimental and that vaccination would have been necessary for avoiding infection. Instead, if by chance they avoided infection (branch (b2)), they will conclude that vaccination was unnecessary. (B) The probability of getting infected with influenza q(p) versus the vaccination coverage p.

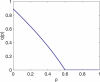
Figure 5

Figure 5. The Probability of Getting Infected q(p) versus the Vaccination Coverage p for the SIR Model with No Vital Dynamics

The parameters are N = 105, β = 5/6 day−1, γ = 1/3 day−1, and T = 200 days.

Similar articles

Cited by

References

    1. Viboud C, Boelle PY, Carrat F, Valleron AJ, Flahault A. Prediction of the spread of influenza epidemics by the method of analogues. Am J Epidemiol. 2003;158:996–1006. - PubMed
    1. Viboud C, Boelle PY, Pakdaman K, Carrat F, Valleron AJ, et al. Influenza epidemics in the United States, France, and Australia, 1972–1997. Emerg Infect Dis. 2004;10:32–39. - PMC - PubMed
    1. Ferguson NM, Cummings DA, Cauchemez S, Fraser C, Riley S, et al. Strategies for containing an emerging influenza pandemic in Southeast Asia. Nature. 2005;437:209–214. - PubMed
    1. Longini IM, Jr, Halloran ME, Nizam A, Yang Y. Containing pandemic influenza with antiviral agents. Am J Epidemiol. 2004;159:623–633. - PubMed
    1. Mills CE, Robins JM, Lipsitch M. Transmissibility of 1918 pandemic influenza. Nature. 2004;432:904–906. - PMC - PubMed

Publication types

MeSH terms

Substances

LinkOut - more resources