What is flux balance analysis? - PubMed (original) (raw)

What is flux balance analysis?

Jeffrey D Orth et al. Nat Biotechnol. 2010 Mar.

Abstract

Flux balance analysis is a mathematical approach for analyzing the flow of metabolites through a metabolic network. This primer covers the theoretical basis of the approach, several practical examples and a software toolbox for performing the calculations.

PubMed Disclaimer

Figures

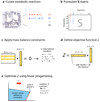
Figure 1

Figure 1

Formulation of an FBA problem.(a) First, a metabolic network reconstruction is built, consisting of a list of stoichiometrically balanced biochemical reactions. (b) Next, this reconstruction is converted into a mathematical model by forming a matrix (labeled S) in which each row represents a metabolite and each column represents a reaction. (c) At steady state, the flux through each reaction is given by the equation Sv = 0. Since there are more reactions than metabolites in large models, there is more than one possible solution to this equation. (d) An objective function is defined as Z = cTv, where c is a vector of weights (indicating how much each reaction contributes to the objective function). In practice, when only one reaction is desired for maximization or minimization, c is a vector of zeros with a one at the position of the reaction of interest. When simulating growth, the objective function will have a 1 at the position of the biomass reaction. (e) Finally, linear programming can be used to identify a particular flux distribution that maximizes or minimizes this objective function while observing the constraints imposed by the mass balance equations and reaction bounds.

Figure 2

Figure 2

The conceptual basis of constraint-based modeling and FBA. With no constraints, the flux distribution of a biological network may lie at any point in a solution space. When mass balance constraints imposed by the stoichiometric matrix S (1) and capacity constraints imposed by the lower and upper bounds (ai and bi) (2) are applied to a network, it defines an allowable solution space. The network may acquire any flux distribution within this space, but points outside this space are denied by the constraints. Through optimization of an objective function, FBA can identify a single optimal flux distribution that lies on the edge of the allowable solution space.

Similar articles

Cited by

References

    1. Duarte NC, et al. Global reconstruction of the human metabolic network based on genomic and bibliomic data. Proceedings of the National Academy of Sciences of the United States of America. 2007;104:1777–1782. - PMC - PubMed
    1. Feist AM, et al. A genome-scale metabolic reconstruction for Escherichia coli K-12 MG1655 that accounts for 1260 ORFs and thermodynamic information. Molecular systems biology. 2007;3 - PMC - PubMed
    1. Feist AM, Palsson BO. The growing scope of applications of genome-scale metabolic reconstructions using Escherichia coli. Nat Biotech. 2008;26:659–667. - PMC - PubMed
    1. Reed JL, Vo TD, Schilling CH, Palsson BO. An expanded genome-scale model of Escherichia coli K-12 (iJR904 GSM/GPR) Genome biology. 2003;4:R54.51–R54.12. - PMC - PubMed
    1. Oberhardt MA, Palsson BO, Papin JA. Applications of genome-scale metabolic reconstructions. Molecular systems biology. 2009;5:320. - PMC - PubMed

Publication types

MeSH terms

Substances

LinkOut - more resources