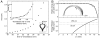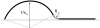Finessing the fracture energy barrier in ballistic seed dispersal - PubMed (original) (raw)
Finessing the fracture energy barrier in ballistic seed dispersal
Robert D Deegan. Proc Natl Acad Sci U S A. 2012.
Abstract
Fracture is a highly dissipative process in which much of the stored elastic energy is consumed in the creation of new surfaces. Surprisingly, many plants use fracture to launch their seeds despite its seemingly prohibitive energy cost. Here we use Impatiens glandulifera as model case to study the impact of fracture on a plant's throwing capacity. I. glandulifera launches its seeds with speeds up to 4 m/s using cracks to trigger an explosive release of stored elastic energy. We find that the seed pod is optimally designed to minimize the cost of fracture. These characteristics may account for its success at invading Europe and North America.
Conflict of interest statement
The author declares no conflict of interest.
Figures
Fig. 1.
(A) Impatiens glandulifera seedpod with two seams indicated and top/bottom convention marked. (B) An unconstrained pod valve. The relaxed state is curled. (C) Top and side view of a valve glued to a glass slide. Each valve is approximately 20 mm long, and consists of a rigid section approximately 15 mm long with a triangular cross-section which widens and thickens along the length and a thin-walled and floppy 5 mm long membrane. The (red) dashed line demarcates the boundary between the floppy and the rigid parts. The scale bar corresponds to 5.6 mm. (D) Photographic sequence of the pod opening extracted from a high speed video. From left to right t = 0; 263; 789; 1,578; 2,104 μs. (see also
Movie S1
). Note that only the upper rigid section curls and accelerates the load.
Fig. 2.
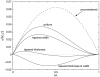
(A) Force vs. displacement for a 26 mm long seedpod pulled from a relaxed state to its original length. Bottom right inset: cartoon of force-displacement apparatus showing a pod in an almost fully relaxed state. Top inset: Same data as in main figure but with the end-to-end distance normalized by the length. The solid line shows the result of a finite element calculation for the same geometry with a Mooney-Rivlin constitutive relation. (B) Results of finite element calculation. Elastic energy per valve (top) and energy release rate (bottom) for a seedpod (17.5 mm rigid section length) with a crack of length L - s extending from the stem downward. When the pod is fully sealed the elastic energy is 0.2 mJ per valve. As the crack extends virtually no energy is released until the crack is longer than 12 mm. Conversely, the pod is able to maintain its structural integrity even if the seams are split along 70% of their length. The energy release rates were calculated by differentiating the fit to the data for the elastic energy per valve (shown as solid line in top). Dashed lines shown the threshold value for unstable crack growth for a seam with 25 μm and 250 μm thickness. Inset: finite element model with arc length s shown as the distance from the bottom. The z = 0 plane is impenetrable and represents a neighboring valve.
Fig. 3.
Results of elastica computation for beams of varying cross section. The dashed curve shows the relaxed state of the beam. The solid curves show the shape of the beam for various tapers for the boundary conditions y = 0 at s = 0 and dy/ds = 0 at s = L corresponding physically to the attachment of the valve to its neighbor at a point (at s = 0) and along a line (at s = L). The result for the uniform and tapered width cases indicate that the beginning part of the valve will relax towards its preferred curvature κ o. Conversely, the beam crosses the y = 0 plane for both cases in which the thickness is tapered. This result occurs because it is energetically favorable to allow the thicker portions of the beam relax towards the preferred curvature and use the thinner portions to satisfy the y = 0 boundary condition. However, in a pod the valve would be prevented from crossing the y = 0 plane by its neighbor, and thus the valve will do the next best thing which is to press flat against its neighbor.
Fig. 4.
Illustration of negative curvature (indicated by dashed segment) produced when part of the pod relaxes to its natural curvature κ o while maintaining the boundary conditions dictated by bonding to neighboring valves. In the absence of a taper the decrease in energy from the relaxed portion outweighs the increase due to the negatively curved portion. With a taper in the thickness of the valve the energy balance is reversed: the energy increase due to the negatively curved segment outweighs the energy decrease due to relaxed segment because the negative curvature is on a thicker—and thus more rigid—segment.
Fig. 5.
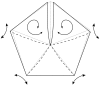
Schematic illustrating the coupling between valves as viewed down the symmetry axis of the pod. When the bond between adjacent valves cracks, the valves begin to curl which in turn causes all the valves to rotate about the unbroken seams. The original uncracked arrangement of valves is shown in light gray. The mechanical model constructed from paper is given in
Fig. S1
and its mechanical response to bending is given in
Fig. S2
.
Similar articles
- The mechanics of explosive seed dispersal in orange jewelweed (Impatiens capensis).
Hayashi M, Feilich KL, Ellerby DJ. Hayashi M, et al. J Exp Bot. 2009;60(7):2045-53. doi: 10.1093/jxb/erp070. Epub 2009 Mar 25. J Exp Bot. 2009. PMID: 19321647 Free PMC article. - The ability of seeds to float with water currents contributes to the invasion success of Impatiens balfourii and I. glandulifera.
Najberek K, Olejniczak P, Berent K, Gąsienica-Staszeczek M, Solarz W. Najberek K, et al. J Plant Res. 2020 Sep;133(5):649-664. doi: 10.1007/s10265-020-01212-0. Epub 2020 Jul 3. J Plant Res. 2020. PMID: 32621067 Free PMC article. - The seed dispersal catapult of Cardamine parviflora (Brassicaceae) is efficient but unreliable.
Hayashi M, Gerry SP, Ellerby DJ. Hayashi M, et al. Am J Bot. 2010 Oct;97(10):1595-601. doi: 10.3732/ajb.1000173. Epub 2010 Sep 16. Am J Bot. 2010. PMID: 21616794 - Seed dispersal by neotropical seed predators.
Norconk MA, Grafton BW, Conklin-Brittain NL. Norconk MA, et al. Am J Primatol. 1998;45(1):103-26. doi: 10.1002/(SICI)1098-2345(1998)45:1<103::AID-AJP8>3.0.CO;2-#. Am J Primatol. 1998. PMID: 9573445 Review. - Seeds of future past: climate change and the thermal memory of plant reproductive traits.
Fernández-Pascual E, Mattana E, Pritchard HW. Fernández-Pascual E, et al. Biol Rev Camb Philos Soc. 2019 Apr;94(2):439-456. doi: 10.1111/brv.12461. Epub 2018 Sep 6. Biol Rev Camb Philos Soc. 2019. PMID: 30188004 Review.
Cited by
- Fracture-driven power amplification in a hydrogel launcher.
Wang X, Pan C, Xia N, Zhang C, Hao B, Jin D, Su L, Zhao J, Majidi C, Zhang L. Wang X, et al. Nat Mater. 2024 Oct;23(10):1428-1435. doi: 10.1038/s41563-024-01955-4. Epub 2024 Jul 23. Nat Mater. 2024. PMID: 39043929 - Digital image correlation techniques for motion analysis and biomechanical characterization of plants.
Mylo MD, Poppinga S. Mylo MD, et al. Front Plant Sci. 2024 Jan 11;14:1335445. doi: 10.3389/fpls.2023.1335445. eCollection 2023. Front Plant Sci. 2024. PMID: 38273955 Free PMC article. Review. - Elastic pinch biomechanisms can yield consistent launch speeds regardless of projectile mass.
Jorge JF, Patek SN. Jorge JF, et al. J R Soc Interface. 2023 Aug;20(205):20230234. doi: 10.1098/rsif.2023.0234. Epub 2023 Aug 23. J R Soc Interface. 2023. PMID: 37608709 Free PMC article. - Diffusion-Limited Processes in Hydrogels with Chosen Applications from Drug Delivery to Electronic Components.
Lavrentev FV, Shilovskikh VV, Alabusheva VS, Yurova VY, Nikitina AA, Ulasevich SA, Skorb EV. Lavrentev FV, et al. Molecules. 2023 Aug 7;28(15):5931. doi: 10.3390/molecules28155931. Molecules. 2023. PMID: 37570901 Free PMC article. Review. - The cracking of Scots pine (Pinus sylvestris) cones.
Horstmann M, Buchheit H, Speck T, Poppinga S. Horstmann M, et al. Front Plant Sci. 2022 Oct 18;13:982756. doi: 10.3389/fpls.2022.982756. eCollection 2022. Front Plant Sci. 2022. PMID: 36330256 Free PMC article.
References
- Skotheim JM, et al. Physical limits and design principles for plant and fungal movements. Science. 2005;308:1308–1310. - PubMed
- Vogel S, et al. Cat’s Paws and Catapults. New York: WW. Norton & Company, Inc; 1998.
- Usher MB, et al. Invasibility and wildlife conservation: invasive species on nature reserves [and discussion] Philos T R Soc Lond S-B. 1986;314:695–710.
- Williamson M, et al. Biological Invasions. London: Chapman and Hall; 1996.
- Kollmann J, et al. Latitudinal trends in growth and phenology of the invasive alien plant impatiens glandulifera (balsaminaceae) Divers Distrib. 2004;10:377–385.
Publication types
MeSH terms
LinkOut - more resources
Full Text Sources