GSVA: gene set variation analysis for microarray and RNA-seq data - PubMed (original) (raw)
GSVA: gene set variation analysis for microarray and RNA-seq data
Sonja Hänzelmann et al. BMC Bioinformatics. 2013.
Abstract
Background: Gene set enrichment (GSE) analysis is a popular framework for condensing information from gene expression profiles into a pathway or signature summary. The strengths of this approach over single gene analysis include noise and dimension reduction, as well as greater biological interpretability. As molecular profiling experiments move beyond simple case-control studies, robust and flexible GSE methodologies are needed that can model pathway activity within highly heterogeneous data sets.
Results: To address this challenge, we introduce Gene Set Variation Analysis (GSVA), a GSE method that estimates variation of pathway activity over a sample population in an unsupervised manner. We demonstrate the robustness of GSVA in a comparison with current state of the art sample-wise enrichment methods. Further, we provide examples of its utility in differential pathway activity and survival analysis. Lastly, we show how GSVA works analogously with data from both microarray and RNA-seq experiments.
Conclusions: GSVA provides increased power to detect subtle pathway activity changes over a sample population in comparison to corresponding methods. While GSE methods are generally regarded as end points of a bioinformatic analysis, GSVA constitutes a starting point to build pathway-centric models of biology. Moreover, GSVA contributes to the current need of GSE methods for RNA-seq data. GSVA is an open source software package for R which forms part of the Bioconductor project and can be downloaded at http://www.bioconductor.org.
Figures
Figure 1
GSVA methods outline. The input for the GSVA algorithm are a gene expression matrix in the form of log2 microarray expression values or RNA-seq counts and a database of gene sets. 1. Kernel estimation of the cumulative density function (kcdf). The two plots show two simulated expression profiles mimicking 6 samples from microarray and RNA-seq data. The _x_-axis corresponds to expression values where each gene is lowly expressed in the four samples with lower values and highly expressed in the other two. The scale of the kcdf is on the left _y_-axis and the scale of the Gaussian and Poisson kernels is on the right _y_-axis. 2. The expression-level statistic is rank ordered for each sample. 3. For every gene set, the Kolmogorov-Smirnov-like rank statistic is calculated. The plot illustrates a gene set consisting of 3 genes out of a total number of 10 with the sample-wise calculation of genes inside and outside of the gene set. 4. The GSVA enrichment score is either the maximum deviation from zero (top) or the difference between the two sums (bottom). The two plots show two simulations of the resulting scores under the null hypothesis of no gene expression change (see main text). The output of the algorithm is a matrix containing pathway enrichment scores for each gene set and sample.
Figure 2
Comparison of statistical power and type-I error rate between GSVA, PLAGE, single sample GSEA (ssGSEA) and combined z-score (zscore). The averaged results of 1,000 simulations are depicted as function of the sample size on the _x_-axis, for each of the GSE methods. On the _y_-axis either the statistical power (A, C, E, G) or the empirical type-I error rate (B, D, F, H) is shown. Data were simulated from a linear additive model with sample and probe effects (see Methods) for _p_=1,000 genes. GSE scores were calculated with each method with respect to two gene sets, one of them differentially expressed (DE) and the other one not. Statistical power and empirical type-I error rates were estimated by performing a _t_-test on the DE and non-DE gene sets, respectively, at a significance level of _α_=0.05. These simulations were carried out under the following four different scenarios for the DE gene set: (A,B) weak signal-to-noise ratio, 50% of DE genes in the DE gene set; (C,D) strong signal-to-noise ratio, 50% of DE genes in the DE gene set; (E, F) weak signal-to-noise ratio, 80% of DE genes in the DE gene set; (G, H) strong signal-to-noise ratio, 80% of DE genes in the DE gene set.
Figure 3
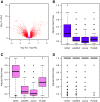
Comparison of differential pathway activity identification of GSVA, PLAGE, single sample GSEA (ssGSEA) and combined z-score (zscore). Each panel shows the area under the ROC curve (AUC) on the _y_-axis for differentially expressed genes predicted by each method at 5% FDR over 100 simulations (see Methods). On top of each boxplot the p-value of the _t_-test for no difference in means between GSVA and the corresponding method is reported. The two panels on top correspond to simulations where 50% of the genes in DE gene sets were DE while the two at the bottom contained 80% of DE genes on those DE gene sets. The two panels on the left correspond to a weak signal-to-noise ratio in the DE magnitude while the two on the right correspond to a strong one. Diamonds indicate mean values in boxplots.
Figure 4
Comparison of the predictive power for survival analysis of gene-level, GSVA, PLAGE, single sample GSEA (ssGSEA) and combined z-score (zscore) on simulated data. Each panel corresponds to a different sample size of the simulated data. The _y_-axis shows the concordance index values of predicting survival risk on test data from 100 independent simulations. On top of each boxplot the p-value of the _t_-test for no difference in means between GSVA and the corresponding method is reported. The method gene refers to a simple gene-level survival model built from the top 10 genes with lowest p-values reported by the Wald test performed on the training data. Diamonds indicate mean values in boxplots.
Figure 5
Comparison of differential pathway activity identification of GSVA, PLAGE, single sample GSEA (ssGSEA) and combined z-score (zscore) on a leukemia data set. (A) Volcano plot of gene expression changes in the Leukemia data set. Genes highlighted in red form the first tercile of largest absolute fold changes, violet indicates the second tercile and blue the third tercile. (B-D) Adjusted rand index (ARI) indicating the accuracy of classifying the two groups of samples by hierarchical clustering of the enrichment scores produced by each of the compared methods at the top-5 differentially activated gene sets. The distribution of ARI values is formed by bootstrapping 1,000 times 10 samples from each sample group. Colors match the key given for genes in the volcano plot of (A) and show that, as expected, genes with larger fold changes lead to larger ARI values. However, when fold changes are small (B-C) and the underlying signature becomes extremely subtle, GSVA produces enrichment scores that lead to differentially activated gene sets which classify the two sample groups substantially better than using ssGSEA, zscore or PLAGE.
Figure 6
Survival analysis in a TCGA ovarian cancer data set. Predictive performance in the survival analysis of a TCGA ovarian cancer microarray data set of _n_=588 samples, measured by the concordance index obtained from a 5-fold cross-validation from (A) the training data and (B) the test data. Diamonds indicate means in boxplots. Except in the training data using the gene-level model, GSVA provides higher mean and median concordance index values than the other compared methods in both training and testing cross-validated data sets.
Figure 7
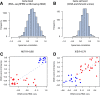
GSVA for RNA-seq (Argonne). A. Distribution of Spearman correlation values between gene expression profiles of RNA-seq and microarray data. B. Distribution of Spearman correlation values between GSVA enrichment scores of gene sets calculated from RNA-seq and microarray data. C and D. Comparison of GSVA enrichment scores obtained from microarray and RNA-seq data for two gene sets containing genes with sex-specific expression: MSY formed by genes of the male-specific region of the Y chromosome (male-specific), and XiE formed by genes that escape X-inactivation in females (female-specific). Red and blue points represent female and male samples, respectively. In both cases GSVA scores show very high correlation between the two profiling technologies where female samples show higher enrichment scores in the female-specific gene set and male samples show higher enrichment scores in the male-specific gene set.
Similar articles
- A flexible count data model to fit the wide diversity of expression profiles arising from extensively replicated RNA-seq experiments.
Esnaola M, Puig P, Gonzalez D, Castelo R, Gonzalez JR. Esnaola M, et al. BMC Bioinformatics. 2013 Aug 21;14:254. doi: 10.1186/1471-2105-14-254. BMC Bioinformatics. 2013. PMID: 23965047 Free PMC article. - Importing ArrayExpress datasets into R/Bioconductor.
Kauffmann A, Rayner TF, Parkinson H, Kapushesky M, Lukk M, Brazma A, Huber W. Kauffmann A, et al. Bioinformatics. 2009 Aug 15;25(16):2092-4. doi: 10.1093/bioinformatics/btp354. Epub 2009 Jun 8. Bioinformatics. 2009. PMID: 19505942 Free PMC article. - Combining multiple tools outperforms individual methods in gene set enrichment analyses.
Alhamdoosh M, Ng M, Wilson NJ, Sheridan JM, Huynh H, Wilson MJ, Ritchie ME. Alhamdoosh M, et al. Bioinformatics. 2017 Feb 1;33(3):414-424. doi: 10.1093/bioinformatics/btw623. Bioinformatics. 2017. PMID: 27694195 Free PMC article. - Comparing bioinformatic gene expression profiling methods: microarray and RNA-Seq.
Mantione KJ, Kream RM, Kuzelova H, Ptacek R, Raboch J, Samuel JM, Stefano GB. Mantione KJ, et al. Med Sci Monit Basic Res. 2014 Aug 23;20:138-42. doi: 10.12659/MSMBR.892101. Med Sci Monit Basic Res. 2014. PMID: 25149683 Free PMC article. Review. - Open source software for the analysis of microarray data.
Dudoit S, Gentleman RC, Quackenbush J. Dudoit S, et al. Biotechniques. 2003 Mar;Suppl:45-51. Biotechniques. 2003. PMID: 12664684 Review.
Cited by
- KRAS mutation promotes the colonization of Fusobacterium nucleatum in colorectal cancer by down-regulating SERTAD4.
Chen Y, Zheng Y, Liu S. Chen Y, et al. J Cell Mol Med. 2024 Oct;28(20):e70182. doi: 10.1111/jcmm.70182. J Cell Mol Med. 2024. PMID: 39462261 Free PMC article. - Construction of a Prognostic Model for Mitochondria and Macrophage Polarization Correlation in Glioma Based on Single-Cell and Transcriptome Sequencing.
Chen P, Wang H, Zhang Y, Qu S, Zhang Y, Yang Y, Zhang C, He K, Dang H, Yang Y, Li S, Yu Y. Chen P, et al. CNS Neurosci Ther. 2024 Nov;30(11):e70083. doi: 10.1111/cns.70083. CNS Neurosci Ther. 2024. PMID: 39491527 Free PMC article. - An inflammatory cytokine signature predicts IgA nephropathy severity and progression.
Chen L, Chen X, Cai G, Jiang H, Chen X, Zhang M. Chen L, et al. MedComm (2020). 2024 Nov 3;5(11):e783. doi: 10.1002/mco2.783. eCollection 2024 Nov. MedComm (2020). 2024. PMID: 39492831 Free PMC article. - Comprehensive analysis of the cancer driver genes constructs a seven-gene signature for prediction of survival and tumor immunity in hepatocellular carcinoma.
Zou J, Qin W. Zou J, et al. Front Genet. 2022 Aug 9;13:937948. doi: 10.3389/fgene.2022.937948. eCollection 2022. Front Genet. 2022. PMID: 36017503 Free PMC article. - Prognostic analysis and validation of diagnostic marker genes in patients with osteoporosis.
Wang X, Pei Z, Hao T, Ariben J, Li S, He W, Kong X, Chang J, Zhao Z, Zhang B. Wang X, et al. Front Immunol. 2022 Oct 13;13:987937. doi: 10.3389/fimmu.2022.987937. eCollection 2022. Front Immunol. 2022. PMID: 36311708 Free PMC article.
References
- Goeman JJ, Geer SAvd, Kort Fd, Houwelingen HCv. A global test for groups of genes: testing association with a clinical outcome. Bioinformatics. 2004;20:93–99. [ http://bioinformatics.oxfordjournals.org/content/20/1/93.abstract] - PubMed
- Mootha VK, Lindgren CM, Eriksson KF, Subramanian A, Sihag S, Lehar J, Puigserver P, Carlsson E, Ridderstråle M, Laurila E, Houstis N, Daly MJ, Patterson N, Mesirov JP, Golub TR, Tamayo P, Spiegelman B, Lander ES, Hirschhorn JN, Altshuler D, Groop LC. PGC-1alpha-responsive genes involved in oxidative phosphorylation are coordinately downregulated in human diabetes. Nature Genet. 2003;34(3):267–273. [ http://www.ncbi.nlm.nih.gov/pubmed/12808457] - PubMed
- Sweet-Cordero A, Mukherjee S, Subramanian A, You H, Roix JJ, Ladd-Acosta C, Mesirov J, Golub TR, Jacks T. An oncogenic KRAS2 expression signature identified by cross-species gene-expression analysis. Nature Gen. 2005;37:48–55. [ http://www.ncbi.nlm.nih.gov/pubmed/15608639] - PubMed
- Subramanian A, Tamayo P, Mootha VK, Mukherjee S, Ebert BL, Gillette MA, Paulovich A, Pomeroy SL, Golub TR, Lander ES, Mesirov JP. Gene set enrichment analysis: A knowledge-based approach for interpreting genome-wide expression profiles. Proc Natl Acad Sci USA. 2005;102(43):15545–15550. [ http://www.pnas.org/content/102/43/15545.abstract] - PMC - PubMed
- Barbie DA, Tamayo P, Boehm JS, Kim SY, Moody SE, Dunn IF, Schinzel AC, Sandy P, Meylan E, Scholl C, Fröhling S, Chan EM, Sos ML, Michel K, Mermel C, Silver SJ, Weir BA, Reiling JH, Sheng Q, Gupta PB, Wadlow RC, Le H, Hoersch S, Wittner BS, Ramaswamy S, Livingston DM, Sabatini DM, Meyerson M, Thomas RK, Lander ES, Mesirov JP, Root DE, Gilliland DG, Jacks T, Hahn WC. Systematic RNA interference reveals that oncogenic KRAS-driven cancers require TBK1. Nature. 2009;462(7269):108–112. [ http://www.nature.com/nature/journal/v462/n7269/abs/nature08460.html] - PMC - PubMed
Publication types
MeSH terms
LinkOut - more resources
Full Text Sources
Other Literature Sources
Molecular Biology Databases
Research Materials