Terahertz conductivity of topological surface states in Bi₁.₅Sb₀.₅Te₁.₈Se₁.₂ - PubMed (original) (raw)
Terahertz conductivity of topological surface states in Bi₁.₅Sb₀.₅Te₁.₈Se₁.₂
Chi Sin Tang et al. Sci Rep. 2013.
Abstract
Topological insulators are electronic materials with an insulating bulk and conducting surface. However, due to free carriers in the bulk, the properties of the metallic surface are difficult to detect and characterize in most topological insulator materials. Recently, a new topological insulator Bi₁.₅Sb₀.₅Te₁.₇Se₁.₃ (BSTS) was found, showing high bulk resistivities of 1-10 Ω.cm and greater contrast between the bulk and surface resistivities compared to other Bi-based topological insulators. Using Terahertz Time-Domain Spectroscopy (THz-TDS), we present complex conductivity of BSTS single crystals, disentangling the surface and bulk contributions. We find that the Drude spectral weight is 1-2 orders of magnitude smaller than in other Bi-based topological insulators, and similar to that of Bi₂Se₃ thin films, suggesting a significant contribution of the topological surface states to the conductivity of the BSTS sample. Moreover, an impurity band is present about 30 meV below the Fermi level, and the surface and bulk carrier densities agree with those obtained from transport data. Furthermore, from the surface Drude contribution, we obtain a ~98% transmission through one surface layer--this is consistent with the transmission through single-layer or bilayer graphene, which shares a common Dirac-cone feature in the band structure.
Figures
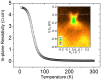
Figure 1. In-plane DC resistivity.
Inset = ARPES data of BSTS single crystal. Notice the band dispersion near the Fermi level.
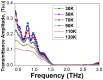
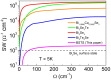
Figure 2. Amplitude of complex transmittance, , at various temperatures.
Figure 3
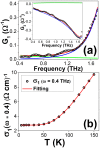
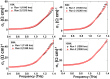
(a) Real conductance _G_1(ω) at 30 K. Inset shows the imaginary conductance _G_1(ω). (O) = data. Solid lines = Drude (green) and Lorentz (blue) contributions, and the fit to data (red). (b) Low-frequency real conductivity σ1 versus temperature. (O) = data. Solid line = fit to Eq. (3).
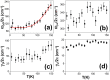
Figure 4. Temperature dependence of the (a) Drude plasma frequency ωpD, (b) Lorentz plasma frequency ωpL, (c) Drude scattering rate γD, and (d) Lorentz scattering rate γL.
Solid line = fit to Eq. (3).
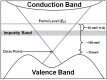
Figure 5. Schematic of band diagram of BSTS, with the (donor) impurity band lying just below the Fermi level.
The relative positions of the valence band, Dirac point, Fermi level and conduction band were taken from Ref. for a Bi1.5Sb0.5Te1.7Se1.3 sample. Δ = activation gap.
Figure 6. Spectral weight of various Bi-based topological insulators at 5 K calculated using Eq. 4.
Dashed line represents the contribution from topological surface carriers of Bi2Se3 thin film. Adapted with permission from Fig. 4 of Ref. . Copyright 2012 by the American Physical Society.
Figure 7. Real conductivity [_σ_1(ω)] of a 90-μm-thick BSTS sample at 10 K, 50 K, 90 K and 150 K. Experimental data were taken in the sequence: 10 K → 50 K → 90 K → 150 K → 10 K → 50 K → 90 K → 150 K.
Similar articles
- Tunable Dirac cone in the topological insulator Bi(2-x)Sb(x)Te(3-y)Se(y).
Arakane T, Sato T, Souma S, Kosaka K, Nakayama K, Komatsu M, Takahashi T, Ren Z, Segawa K, Ando Y. Arakane T, et al. Nat Commun. 2012 Jan 24;3:636. doi: 10.1038/ncomms1639. Nat Commun. 2012. PMID: 22273674 - Intrinsic topological insulator Bi(1.5)Sb(0.5)Te(3-x)Se(x) thin crystals.
Wang W, Li L, Zou W, He L, Song F, Zhang R, Wu X, Zhang F. Wang W, et al. Sci Rep. 2015 Jan 21;5:7931. doi: 10.1038/srep07931. Sci Rep. 2015. PMID: 25604579 Free PMC article. - Realization of tunable Dirac cone and insulating bulk states in topological insulators (Bi(1-x)Sb(x))(2)Te(3).
Niu C, Dai Y, Zhu Y, Ma Y, Yu L, Han S, Huang B. Niu C, et al. Sci Rep. 2012;2:976. doi: 10.1038/srep00976. Epub 2012 Dec 13. Sci Rep. 2012. PMID: 23240080 Free PMC article. - Metal-insulator transition in variably doped (Bi(1-x)Sb(x))2Se3 nanosheets.
Lee CH, He R, Wang Z, Qiu RL, Kumar A, Delaney C, Beck B, Kidd TE, Chancey CC, Sankaran RM, Gao XP. Lee CH, et al. Nanoscale. 2013 May 21;5(10):4337-43. doi: 10.1039/c3nr01155k. Nanoscale. 2013. PMID: 23563061 - Molecular beam epitaxial growth of topological insulators.
Chen X, Ma XC, He K, Jia JF, Xue QK. Chen X, et al. Adv Mater. 2011 Mar 4;23(9):1162-5. doi: 10.1002/adma.201003855. Adv Mater. 2011. PMID: 21360770 Review.
Cited by
- New insights into the diverse electronic phases of a novel vanadium dioxide polymorph: a terahertz spectroscopy study.
Lourembam J, Srivastava A, La-o-vorakiat C, Rotella H, Venkatesan T, Chia EE. Lourembam J, et al. Sci Rep. 2015 Mar 17;5:9182. doi: 10.1038/srep09182. Sci Rep. 2015. PMID: 25777320 Free PMC article. - Intrinsically core-shell plasmonic dielectric nanostructures with ultrahigh refractive index.
Yue Z, Cai B, Wang L, Wang X, Gu M. Yue Z, et al. Sci Adv. 2016 Mar 25;2(3):e1501536. doi: 10.1126/sciadv.1501536. eCollection 2016 Mar. Sci Adv. 2016. PMID: 27051869 Free PMC article. - Anisotropic Terahertz Emission from Bi2Se3 Thin Films with Inclined Crystal Planes.
Hamh SY, Park SH, Han J, Jeon JH, Kahng SJ, Kim S, Choi SH, Bansal N, Oh S, Park J, Kim JS, Kim JM, Noh do Y, Lee JS. Hamh SY, et al. Nanoscale Res Lett. 2015 Dec;10(1):489. doi: 10.1186/s11671-015-1190-y. Epub 2015 Dec 15. Nanoscale Res Lett. 2015. PMID: 26694079 Free PMC article. - Topological-insulator-based terahertz modulator.
Wang XB, Cheng L, Wu Y, Zhu DP, Wang L, Zhu JX, Yang H, Chia EEM. Wang XB, et al. Sci Rep. 2017 Oct 18;7(1):13486. doi: 10.1038/s41598-017-13701-9. Sci Rep. 2017. PMID: 29044164 Free PMC article. - Study on Bulk-Surface Transport Separation and Dielectric Polarization of Topological Insulator Bi1.2Sb0.8Te0.4Se2.6.
Zheng Y, Xu T, Wang X, Sun Z, Han B. Zheng Y, et al. Molecules. 2024 Feb 15;29(4):859. doi: 10.3390/molecules29040859. Molecules. 2024. PMID: 38398611 Free PMC article.
References
- Moore J. E. The birth of topological insulators. Nature 464, 194 (2010). - PubMed
- Hasan M. Z. & Kane C. L. Topological insulators. Rev. Mod. Phys. 82, 3045 (2010).
- Hsieh D. et al. A topological Dirac insulator in a quantum spin Hall phase. Nature (London) 452, 970–974 (2008). - PubMed
- Checkelsky J. G. et al. Quantum Interference in Macroscopic Crystals of Nonmetallic Bi2Se3. Phys. Rev. Lett. 103, 246601 (2009). - PubMed
- Eto K. et al. Angular-dependent oscillations of the magnetoresistance in Bi2Se3 due to the three-dimensional bulk Fermi surface. Phys. Rev. B 81, 195309 (2010).
Publication types
LinkOut - more resources
Full Text Sources
Other Literature Sources