Capillary pumping independent of the liquid surface energy and viscosity - PubMed (original) (raw)
Capillary pumping independent of the liquid surface energy and viscosity
Weijin Guo et al. Microsyst Nanoeng. 2018.
Abstract
Capillary pumping is an attractive means of liquid actuation because it is a passive mechanism, i.e., it does not rely on an external energy supply during operation. The capillary flow rate generally depends on the liquid sample viscosity and surface energy. This poses a problem for capillary-driven systems that rely on a predictable flow rate and for which the sample viscosity or surface energy are not precisely known. Here, we introduce the capillary pumping of sample liquids with a flow rate that is constant in time and independent of the sample viscosity and sample surface energy. These features are enabled by a design in which a well-characterized pump liquid is capillarily imbibed into the downstream section of the pump and thereby pulls the unknown sample liquid into the upstream pump section. The downstream pump geometry is designed to exert a Laplace pressure and fluidic resistance that are substantially larger than those exerted by the upstream pump geometry on the sample liquid. Hence, the influence of the unknown sample liquid on the flow rate is negligible. We experimentally tested pumps of the new design with a variety of sample liquids, including water, different samples of whole blood, different samples of urine, isopropanol, mineral oil, and glycerol. The capillary filling speeds of these liquids vary by more than a factor 1000 when imbibed to a standard constant cross-section glass capillary. In our new pump design, 20 filling tests involving these liquid samples with vastly different properties resulted in a constant volumetric flow rate in the range of 20.96-24.76 μL/min. We expect this novel capillary design to have immediate applications in lab-on-a-chip systems and diagnostic devices.
Conflict of interest statement
The authors declare that they have no conflict of interest.
Figures
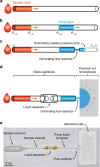
Fig. 1. Design and implementation of the capillary pump principle.
a The capillary flow of a sample liquid in a constant cross-section capillary depends on the sample liquid properties; b adding a second liquid, the pump liquid generates a flow dependency on both the sample liquid and the pump liquid; c creating a flow restrictor and decreasing the capillary cross-section in the pump liquid region generates a flow dependency solely on the pump liquid; d sketch of the capillary pump using three concatenated glass capillary tubes and precision cut nitrocellulose paper; and e photograph of a capillary pump.
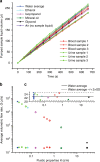
Fig. 2
a Measurements of the pumped sample volume versus time for our novel capillary pump. Solid lines are for visualization purposes only. b Capillary volumetric flow rate, Q, versus κ for the sample liquids tested in our novel capillary pump (solid markers) and in standard capillaries (hollow markers). The volumetric flow rate was determined as the ratio of the measured total pumped sample volume over the measured total pumping time. The value depicted for water is the average over ten measurements. c Zoomed view of the novel capillary pump flow rate results with the ten flow rate values for water plotted separately. The solid and dashed lines indicate the average flow rate for water and two times the standard deviation of the flow rate for water, respectively.
Similar articles
- Capillary Pumping Independent of Liquid Sample Viscosity.
Guo W, Hansson J, van der Wijngaart W. Guo W, et al. Langmuir. 2016 Dec 6;32(48):12650-12655. doi: 10.1021/acs.langmuir.6b03488. Epub 2016 Nov 14. Langmuir. 2016. PMID: 27798835 - Bubble pump: scalable strategy for in-plane liquid routing.
Oskooei A, Günther A. Oskooei A, et al. Lab Chip. 2015 Jul 7;15(13):2842-53. doi: 10.1039/c5lc00326a. Epub 2015 May 28. Lab Chip. 2015. PMID: 26016773 - Dynamic control of capillary flow in porous media by electroosmotic pumping.
Rosenfeld T, Bercovici M. Rosenfeld T, et al. Lab Chip. 2019 Jan 15;19(2):328-334. doi: 10.1039/c8lc01077c. Lab Chip. 2019. PMID: 30566158 - Capillary interactions between particles bound to interfaces, liquid films and biomembranes.
Kralchevsky PA, Nagayama K. Kralchevsky PA, et al. Adv Colloid Interface Sci. 2000 Mar 31;85(2-3):145-92. doi: 10.1016/s0001-8686(99)00016-0. Adv Colloid Interface Sci. 2000. PMID: 10768480 Review. - Fundamental structural aspects and features in the bioengineering of the gas exchangers: comparative perspectives.
Maina JN. Maina JN. Adv Anat Embryol Cell Biol. 2002;163:III-XII, 1-108. doi: 10.1007/978-3-642-55917-4. Adv Anat Embryol Cell Biol. 2002. PMID: 11892241 Review.
Cited by
- Wicking pumps for microfluidics.
Aghajanloo B, Losereewanich W, Pastras CJ, Inglis DW. Aghajanloo B, et al. Biomicrofluidics. 2024 Nov 1;18(6):061501. doi: 10.1063/5.0218030. eCollection 2024 Dec. Biomicrofluidics. 2024. PMID: 39494378 Free PMC article. Review. - Methods to spontaneously generate three dimensionally arrayed microdroplets triggered by capillarity for bioassays and bioengineering.
Yasuga H. Yasuga H. Biophys Physicobiol. 2023 Jun 9;20(2):e200029. doi: 10.2142/biophysico.bppb-v20.0029. eCollection 2023. Biophys Physicobiol. 2023. PMID: 38496237 Free PMC article. - A Miniaturized Archimedean Screw Pump for High-Viscosity Fluid Pumping in Microfluidics.
Gucluer S. Gucluer S. Micromachines (Basel). 2023 Jul 12;14(7):1409. doi: 10.3390/mi14071409. Micromachines (Basel). 2023. PMID: 37512720 Free PMC article. - Eliminating viscosity bias in lateral flow tests.
Kainz DM, Breiner BJ, Früh SM, Hutzenlaub T, Zengerle R, Paust N. Kainz DM, et al. Microsyst Nanoeng. 2021 Sep 6;7:72. doi: 10.1038/s41378-021-00296-5. eCollection 2021. Microsyst Nanoeng. 2021. PMID: 34567784 Free PMC article. - Nested-channel for on-demand alternation between electrospray ionization regimes.
Li M, Li H, Allen NR, Wang T, Li L, Schwartz J, Li A. Li M, et al. Chem Sci. 2020 Dec 22;12(5):1907-1914. doi: 10.1039/d0sc06221a. Chem Sci. 2020. PMID: 34163954 Free PMC article.
References
- Chi, S. W. Heat Pipe Theory and Practice 256 (McGraw-Hill Book Co., New York, 1976).
- Siegel R. Transient capillary rise in reduced and zero-gravity fields. J. Appl. Mech. 1961;28:165. doi: 10.1115/1.3641647. - DOI
LinkOut - more resources
Full Text Sources
Other Literature Sources
Miscellaneous