The cost of noise: Stochastic punishment falls short of sustaining cooperation in social dilemma experiments - PubMed (original) (raw)
The cost of noise: Stochastic punishment falls short of sustaining cooperation in social dilemma experiments
Mohammad Salahshour et al. PLoS One. 2022.
Abstract
Identifying mechanisms able to sustain costly cooperation among self-interested agents is a central problem across social and biological sciences. One possible solution is peer punishment: when agents have an opportunity to sanction defectors, classical behavioral experiments suggest that cooperation can take root. Overlooked from standard experimental designs, however, is the fact that real-world human punishment-the administration of justice-is intrinsically noisy. Here we show that stochastic punishment falls short of sustaining cooperation in the repeated public good game. As punishment noise increases, we find that contributions decrease and punishment efforts intensify, resulting in a 45% drop in gains compared to a noiseless control. Moreover, we observe that uncertainty causes a rise in antisocial punishment, a mutually harmful behavior previously associated with societies with a weak rule of law. Our approach brings to light challenges to cooperation that cannot be explained by economic rationality and strengthens the case for further investigations of the effect of noise-and not just bias-on human behavior.
Conflict of interest statement
The authors have declared that no competing interests exist.
Figures
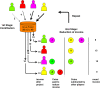
Fig 1. Experimental design.
Each round of our PGG experiments consists of two stages. In the first stage, participants invest an amount from 0 to 10 MUs in a public good (“group project”). The returns to this investment (the total amount invested times r = 2) are then shared equally between all participants, irrespective of their contribution. In the second stage, participants are given an opportunity to reduce the income, i.e. punish, other participants. For this, they can spend up to 10 MUs (“coins”) to punish other participants; each MU is multiplied by a factor β and the resulting amount is substrated from the punishee’s account. In the control group, β is fixed to the value 3; in treatment groups, β is a random variable with mean 3 and varying standard deviation.
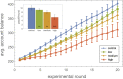
Fig 2. The cost of noise.
The average account balance during the course of the experiment, for the control (blue), and the low (green), medium (orange), and high (red) noise conditions. Averages are taken over all groups. Inset: Mean payoff per round (the after contribution and punishment stages). Error bars are standard errors, and stars refer to Wilcoxon rank-sum tests comparing each treatment with the control (p = 0.006, p = 0.004 and p = 0.0008 respectively).
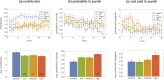
Fig 3. Lower contributions, stronger punishment.
Time series (top row) and time-averaged (bottom row) contribution to the public good (a), probability to punish at least one player (b), and total cost paid to punish (c). Error bars are standard errors, and stars refer to Wilcoxon rank-sum tests comparing each treatment with the control.
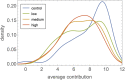
Fig 4. Hedging one’s bets.
Density of per-player average contributions in the four groups. While most players settled on near-maximal contributions in the control group (≃10 MU), noise induced many participants to “hedge their bets” and contribute intermediate amounts (≃5 MU). Densities above 10 or below 0 MU are an artifact of the smoothing procedure.
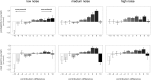
Fig 5. Uncertainty begets antisociality.
The probability to punish a player (top row) and the average cost assigned to punish them (bottom row) as a function of the contribution difference = (punishee’s contribution) − (punisher’s contribution), compared to the control. Under noise, the probability and intensity of strongly prosocial punishment (negative contribution difference) are reduced, and the probability and intensity of antisocial punishment (positive contribution difference) are increased.
Similar articles
- Retaliation and antisocial punishment are overlooked in many theoretical models as well as behavioral experiments.
Dreber A, Rand DG. Dreber A, et al. Behav Brain Sci. 2012 Feb;35(1):24. doi: 10.1017/S0140525X11001221. Behav Brain Sci. 2012. PMID: 22289313 - Reciprocity: weak or strong? What punishment experiments do (and do not) demonstrate.
Guala F. Guala F. Behav Brain Sci. 2012 Feb;35(1):1-15. doi: 10.1017/S0140525X11000069. Behav Brain Sci. 2012. PMID: 22289303 - The punishment that sustains cooperation is often coordinated and costly.
Bowles S, Boyd R, Mathew S, Richerson PJ. Bowles S, et al. Behav Brain Sci. 2012 Feb;35(1):20-1. doi: 10.1017/S0140525X1100118X. Behav Brain Sci. 2012. PMID: 22289309 - Overpunishing is not necessary to fix cooperation in voluntary public goods games.
Dercole F, De Carli M, Della Rossa F, Papadopoulos AV. Dercole F, et al. J Theor Biol. 2013 Jun 7;326:70-81. doi: 10.1016/j.jtbi.2012.11.034. Epub 2012 Dec 7. J Theor Biol. 2013. PMID: 23228364 - On the interaction of the stick and the carrot in social dilemmas.
Milinski M, Rockenbach B. Milinski M, et al. J Theor Biol. 2012 Apr 21;299:139-43. doi: 10.1016/j.jtbi.2011.03.014. Epub 2011 Mar 31. J Theor Biol. 2012. PMID: 21458464 Review.
Cited by
- Evolution of cooperation and consistent personalities in public goods games.
Salahshour M. Salahshour M. Sci Rep. 2021 Dec 9;11(1):23708. doi: 10.1038/s41598-021-03045-w. Sci Rep. 2021. PMID: 34887470 Free PMC article. - Cooperation without punishment.
Battu B, Rahwan T. Battu B, et al. Sci Rep. 2023 Jan 21;13(1):1213. doi: 10.1038/s41598-023-28372-y. Sci Rep. 2023. PMID: 36681708 Free PMC article.
References
- Hamilton WD. The evolution of altruistic behavior. The American Naturalist. 1963. Sep 1;97(896):354–6. doi: 10.1086/497114 - DOI
- Olson M. The Logic of Collective Action. Harvard University Press; 1965.
- Yamagishi T. The provision of a sanctioning system as a public good. Journal of Personality and social Psychology. 1986. Jul;51(1):110. doi: 10.1037/0022-3514.51.1.110 - DOI
MeSH terms
Grants and funding
Funding for this work was provided by the Alexander von Humboldt Foundation in the framework of the Sofja Kovalevskaja Award endowed by the German Federal Ministry of Education and Research. The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.
LinkOut - more resources
Full Text Sources
Research Materials