MATHEMATICA tutorial, part 2.5: Hermite expansion (original) (raw)
Return to computing page for the first course APMA0330
Return to computing page for the second course APMA0340
Return to computing page for the fourth course APMA0360
Return to Mathematica tutorial for the first course APMA0330
Return to Mathematica tutorial for the second course APMA0340
Return to Mathematica tutorial for the fourth course APMA0360
Return to the main page for the first course APMA0330
Return to the main page for the second course APMA0340
Return to the main page for the fourth course APMA0360
Return to Part I of the course APMA0340
Introduction to Linear Algebra with Mathematica
Preface
Since the Hermite polynomials and Hermite functions are eigenfunctions of corresponding singular Sturm--Liouvivve problems for a second order differential operator, they can be used for expansion of functions into series with respect to these eigenfunctions. The Hermite polynomials, conventionally denoted by _H_n(x) were introduced in 1859 by Pafnuty Chebyshev. Later, in 1864 they were studied by the French mathematician Charles Hermite (1822--1901).
Any polynomial (a Hermite polynomial is a particular case) is unbounded on the real axis ℝ = (−∞, ∞), so it does not belong to the Hilbert space 𝔏²(ℝ) of square integrable functions. It turns out that the Hermite polynomials belong to another Hilvert space 𝔏²(ℝ, _e_−x²) and also the space of tempered distributions, 𝒮'(ℝ). Fortunately, Hermite polynomials can be used for expansions of tempered distributions into Fourier--hermite series.
Hermite Polynomials and Functions
The Hermite polynomials \( H_n (x) \) can be defined "explicitly" as
\begin{equation} \label{EqHermite.1} H_n (x) = n! \, \sum_{k=0}^{\left\lfloor n/2 \right\rfloor} \frac{(-1)^k}{k! \left( n-2k \right)!} \left( 2x \right)^{n-2k} , \qquad n=0,1,2,\ldots . \end{equation}
Mathematica has a build-in command for evaluation of the Hermite polynomial: **HermiteH[n, x]**. Since these polynomials contain either even powers of x or odd powers of x, depending on the parity of index n, they possess a symmetry relation:
\[ H_n (-x) = (-1)^n \,H_n (x) , \qquad n=0,1,2,\ldots . \]
Hermite polynomials are eigenfunctions of the singular Sturm--Liouville problem for the Hermite differential equation on the infinite interval (−∞, ∞):
\[ y'' -2x\,y' + \lambda\,y =0 , \qquad \mbox{or} \qquad \frac{{\text d}}{{\text d}x} \left( e^{-x^2} \, \frac{{\text d}y}{{\text d}x} \right) + \lambda e^{-x^2}\, y =0, \qquad \lambda = 2n , \quad n\in \mathbb{N} = \{ 0, 1, 2, \ldots \} , \]
subject to the condition that its solutions grow at infinity no faster than a polynomial. So H_n(x) corresponds to eigenvalue λn = 2_n, with n being a positive integer.
Hermite polynomials can be defined also via Rodrigues formula:
\[ H_n (x) = \frac{\sqrt{\pi}}{2} \left( -1 \right)^n e^{x^2} \frac{{\text d}^{n+1}}{{\text d} x^{n+1}} \mbox{erf}(x) , \qquad \mbox{erf}(x) = \frac{2}{\sqrt{\pi}} \int_0^x e^{-t^2} {\text d}t . \]
Since the leading coefficient in the Hermite polynomial _H_n(x) = 2n_x_n + ··· grows exponentially. It is convenient to consider similar polynomials but with leading coefficient to be 1. There exists another sequence { _He_n(x)} of classical orthogonal polynomial called Chebyshev--Hermite polynomials that are defined explicitly:
\begin{equation} \label{EqHermite.2} He_n (x) = n! \, \sum_{k=0}^{\left\lfloor n/2 \right\rfloor} \frac{(-1)^k}{2^k \,k! \left( n-2k \right)!} \,x^{n-2k} , \qquad n=0,1,2,\ldots . \end{equation}
These polynomials are eigenfunctions of the following singular Sturm--Liouville problem
\[ y'' -x\,y' + \lambda\,y =0, \qquad \lambda = n \in \mathbb{N}. \]
One can define the (normalized) Hermite functions:
\begin{equation} \label{EqHermite.3} \psi_n (x) = \left( 2^n n! \,\sqrt{\pi} \right)^{-1/2} e^{-x^2 /2} \,H_n (x) = (-1)^n \left( 2^n n! \,\sqrt{\pi} \right)^{-1/2} e^{x^2 /2} \,\frac{{\text d}^n}{{\text d} x^n} \left( e^{-x^2} \right) \qquad n=0,1,2,\ldots \end{equation}
that form a complete orthonormal basis in 𝔏²(− ∞, ∞). Their Fourier transforms are
\[ \psi_n^F (\xi ) = \hat{\psi}_n (\xi ) = 𝔉_{x\to\xi}\left[ \psi_n (x) \right] (\xi ) = \mbox{P.V.} \int_{-\infty}^{\infty} e^{{\bf j}x\xi} \psi_n (x)\,{\text d}x = {\bf j}^n \sqrt{2\pi}\,\psi_n (\xi ) , \qquad {\bf j}^2 = -1. \]
Assuming[t > 0, Integrate[ Exp[I*x*t]*HermiteH[8, x]*Exp[-x^2/2], {x, -Infinity, Infinity}]]
6 E^(-(t^2/2)) Sqrt[ 2 \[Pi]] (105 - 840 t^2 + 840 t^4 - 224 t^6 + 16 t^8)
The Hermite functions ψn(x) is the eigenfunction of the harmnic oscillator:
\[ \left( -\frac{{\text d}^2}{{\text d} x^2} + x^2 \right) \psi_n (x) = \left( 2n+1 \right) \psi_n (x) , \qquad n=0,1,2,\ldots . \]
There exists another sequence of functions { _h_n(x) }n≥0, called Chebyshev--Hermite functions that can be defined by the Rodrigues formula:
\[ h_n (x) = (-1)^n \left( \sqrt{2\pi}\, n! \right)^{-1/2} e^{x^2 /4} \frac{{\text d}^n}{{\text d} x^n} \left( e^{- x^2 /2} \right) , \qquad n=0,1,2,\ldots . \]
They are eigenfunction of the harmnic oscillator:
\[ \left( -\frac{{\text d}^2}{{\text d} x^2} + \frac{x^2}{4} \right) h_n (x) = \frac{2n+1}{2}\, h_n (x) , \qquad n=0,1,2,\ldots . \]
h[n_, x_] = (-1)^n *(Sqrt[2*Pi]*Factorial[n])^(-1/2) *Exp[x^2 /4] * D[Exp[-x^2 /2], {x, n}];
FullSimplify[(D[h[7, x], {x, 2}] - x^2*h[7, x]/4)/h[7, x]]
-(15/2)
Orthogonality of Hermite Polynomials
Let us consider a set of real-values functions for which the integral (in Lebesque sense)
\[ \left\langle\, f, f \,\right\rangle_w = \| f \|^2 = \int_{-\infty}^{+\infty} \left\vert f(x) \right\vert^2 e^{-x^2} {\text d}x < \infty \]
is finite, where w = _e_−x² is the weight function. We denote this set as 𝔏²(ℝ, _e_−x²) because its norm \( \| \cdot \| \) depends on the weight function. With respect to this norm, 𝔏²(ℝ, _e_−x²) becomes complete. Upon introducing the inner product
\[ \langle f\,\vert\, g \rangle_w = \langle f,g \rangle_w = \int_{-\infty}^{\infty} f(x)\,g(x)e^{−x^2} \,{\text d}x , \qquad w(x) = e^{-x^2} , \]
the space 𝔏²(ℝ, _e_−x²) of square Lebesque integrable functions becomes a Hilbert space. The Hermite polynomials are orthogonal with respect to this inner product:
\begin{equation} \label{EqHermite.4} \langle H_m (x), H_n (x) \rangle = \int_{-\infty}^{\infty} e^{-x^2} H_m (x)\,H_n (x) \,{\text d} x = \begin{cases} 2^n n! \,\sqrt{\pi} , & \quad \mbox{if n=mn=mn=m}, \\ 0 , & \quad \mbox{if nnemn\ne mnnem}. \end{cases} \end{equation}
Similarly, we introduce the Hilbert space 𝔏²(ℝ, _e_−x²/2) of square Lebesque integrable functions that is equiped with the inner product
\[ \langle f\,\vert\, g \rangle_w = \langle f,g \rangle_w = \int_{-\infty}^{\infty} f(x)\,g(x)e^{−x^2 /2} \,{\text d}x , \qquad w(x) = e^{-x^2 /2} . \]
Then with respect to this inner product, the Chebyshev--Hermite polynomials become orthogonal:
\begin{equation} \label{EqHermite.5} \langle He_m (x), He_n (x) \rangle = \int_{-\infty}^{\infty} e^{-x^2 /2} He_m (x)\,He_n (x) \,{\text d} x = \begin{cases} n!\,\sqrt{2\pi} , & \quad \mbox{if n=mn=mn=m}, \\ 0 , & \quad \mbox{if nnemn\ne mnnem}. \end{cases} \end{equation}
In the Hilbert space 𝔏²(ℝ, d_x_) = 𝔏²(ℝ) of square <achref="https: en.wikipedia.org="" wiki="" lebesgue_integration"="">Lebesque integrable functions, both the Hermite functions and the Chebushev--Hermite functions are orthonormal with respect to standard inner product: </achref="https:>
\[ \int_{-\infty}^{\infty} \psi_n (x) \,\psi_m (x) \,{\text d} x = \delta_{n,m} = \begin{cases} 1 , & \quad \mbox{if n=mn=mn=m}, \\ 0 , & \quad \mbox{if nnemn\ne mnnem}; \end{cases} \qquad \mbox{and} \qquad \int_{-\infty}^{\infty} h_n (x) \,h_m (x) \,{\text d} x = \delta_{n,m} = \begin{cases} 1 , & \quad \mbox{if n=mn=mn=m}, \\ 0 , & \quad \mbox{if nnemn\ne mnnem}. \end{cases} \]
Hermite functions can be efficiently computed using a three-term recursion relation from two starting values:
\[ \psi_{n+1} (x) = \sqrt{\frac{2}{n+1}} \,x\,\psi_n (x) - \sqrt{\frac{n}{n+1}}\,\psi_{n-1} (x) , \qquad \psi_0 (x) = \pi^{-1/4} e^{-x^2 /2} , \quad \psi_1 (x) = \pi^{-1/4} \sqrt{2}\,x \,e^{-x^2 /2} . \]
Similarly,
\[ x\,h_n (x) = \sqrt{n+1}\, h_{n+1} (x) + \sqrt{n}\, h_{n-1} (x) , \qquad n=1,2,\ldots . \]
Hermite Series
Since the sequence of Hermite polynomials and Chebyshev--Hermite polynomials form an orthogonal basis of the Hilbert space of functions 𝔏² with weight function \( e^{-x^2} \quad \mbox{or} \quad e^{-x^2 /2} , \) respectively, a square integrable function with such weight, _f_∈𝔏²(ℝ, w), can be expanded into the Hermite series:
\begin{equation} \label{EqHermite.7} f (x) = \sum_{n\ge 0} a_n H_n (x) , \qquad a_n = \frac{1}{2^n n! \,\sqrt{\pi}}\, \int_{-\infty}^{\infty} f(x)\, e^{-x^2} \,H_n (x) \,{\text d} x, \qquad n\in \mathbb{N} . \end{equation}
So its coefficients are ratios:
\[ a_n = \frac{\langle f, H_n \rangle}{\langle H_n , H_n \rangle} = \frac{\langle f, H_n \rangle}{\| H_n \|^2_w} = \frac{1}{n!\,2^n \sqrt{\pi}} \int_{-\infty}^{+\infty} f(t) \,e^{-t^2} H_n (t)\,{\text d}t , \qquad n=0,1,2,\ldots . \]
Similarly, for f ∈ 𝔏²(ℝ, _e_−x²/2), we have
\begin{equation} \label{EqHermite.6} f (x) = \sum_{n\ge 0} b_n He_n (x) , \qquad b_n = \frac{1}{n! \,\sqrt{2\pi}}\, \int_{-\infty}^{\infty} f(x)\, e^{-x^2/2} \,He_n (x) \,{\text d} x, \qquad n\in \mathbb{N} . \end{equation}
From these formulas, we get for f ∈ 𝔏²(ℝ, _e_−x²) the Bessel inequality
\[ \sum_{n\ge 0} \frac{\langle \, f(x), H_n (x) \,\rangle^2}{\| H_n (x) \|^2} = \sum_{n\ge 0} \left( \int_{\mathbb{R}} f(t) \,e^{-t^2} H_n (t)\,{\text d}t \right)^2 \le \| f \|^2_w = \int_{-\infty}^{\infty} \left\vert f(x) \right\vert^2 e^{-x^2} {\text d}x . \]
This series converges in 𝔏p for \( p \in \left( \frac{4}{3} , 4 \right) . \)
Theorem (Parseval): Let f: (−∞, ∞) → ℝ belongs to the Hilbert space f ∈ 𝔏²(ℝ, _e_−x²). Then Parseval's identity holds
\[ \| f(x) \|^2 = \int_{-\infty}^{\infty} \left\vert f(x) \right\vert^2 e^{-x^2} {\text d}x = \sum_{n\ge 0} a_n^2 \| H_n (x) \|^2 = \sqrt{\pi} \sum_{n\ge 0} a_n^2 2^n n! . \]
Similarly, for f ∈ 𝔏²(ℝ, _e_−x²/2), we have
\[ \| f(x) \|^2 = \int_{-\infty}^{\infty} \left\vert f(x) \right\vert^2 e^{-x^2 /2} {\text d}x = \sum_{n\ge 0} b_n^2 \| He_n (x) \|^2 = \sqrt{2\pi} \sum_{n\ge 0} b_n^2 n! . \]
A function f(x)∈𝔏²(ℝ, d_x_) can be expanded over Hermite functions as well (which equivalent to Eq.\eqref{EqHermite.7})
\begin{equation} \label{EqHermite.9} f(x) = \sum_{n\ge 0} c_n \psi_n (x) , \qquad c_n = \int_{\mathbb{R}} f(x)\,\psi_n (x)\,{\text d}x , \quad n=0,1,2,\ldots . \end{equation}
A function f ∈ 𝔏²(ℝ) can be also expanded into Fourier--Hermite series with respect to Chebyshev--Hermite functions:
\begin{equation} \label{EqHermite.10} f(x) = \sum_{n\ge 0} d_n h_n (x) , \qquad d_n = \int_{\mathbb{R}} f(x)\,h_n (x)\,{\text d}x , \quad n=0,1,2,\ldots . \end{equation}
The series \eqref{EqHermite.10} or \eqref{EqHermite.9}, known as Fourier--Hermite series, converges in 𝔏² sense; however, its pointwise convergence is a very delicated matter.
Theorem (Parseval): Let f: (−∞, ∞) → ℝ belongs to the Hilbert space f ∈ 𝔏²(ℝ). Then Parseval's identities hold
\[ \| f(x) \|^2 = \int_{-\infty}^{\infty} \left\vert f(x) \right\vert^2 {\text d}x = \sum_{n\ge 0} c_n^2 = \sum_{n\ge 0} d_n^2 . \]
Theorem 3 (Hill--Hermite): The domain of convergence of a Hermite series \eqref{EqHermite.7} or \eqref{EqHermite.9} is the infinite strip about the real _x_-axis |Im(x)| < w.
When the function f(x) decays only algebraically with |x|, the convergence of the Hermite series is extremely slow, and thousands of terms may be necessary to obtain an accurate answer.
Theorem 4 (Askey--Wainger): The partial sums \( S_N [f](x) = \sum_{n=0}^N c_n \psi_n (x) \) of the Fourier--Hermite series \eqref{EqHermite.7} converges to f in the 𝔏p norm if and only if 4/3 < p < 4.
This statement was proved in
Askey, R. and Wainger S., Mean convergence of expansions in Laguerre and Hermite series, American Journal of Mathematics,1965, Vol. 87, pp.695--708.
Theorem 5 (Hermite Truncation Error): for f(x) ∈ 𝔏²(−∞, ∞), we have
\[ \left\vert f(x) - \sum_{n=0}^N c_n \psi_n (x) \right\vert \le 0.816 \, \sum_{n\ge N+1} \left\vert c_n \right\vert . \]
because \( \left\vert \psi_n (x) \right\vert \le 0.816 . \)
Boyd’s book contains a thorough discussion of numerical use of Hermite functions. The rate of convergence of a Hermiteseries depends upon the analytical properties of the solution. In general, both the rateat which the function decays along the real _x_-axis and also the location of singularities of f(x) in the complex _x_-plane affect the asymptotic behavior of the Hermitecoefficients.
We illustrate Hermite series expansions in the following examples. Let us start with a simple monoid function---a power function that can be expanded into finite sum of Hermite's polynomials
\[ x^n = \frac{n!}{2^n} \,\sum_{k= 0}^{\left\lfloor n/2 \right\rfloor} \frac{1}{k! \,(n-2k)!} \, H_{n-2k} (x) = n! \sum_{k= 0}^{\left\lfloor n/2 \right\rfloor} \frac{1}{k! \,2^k \,(n-2k)!} \, He_{n-2k} (x) , \qquad n \in \mathbb{Z}_{+} . \]
Example 1:
We demonstrate the Hermite expansion for the following polynomial \( p(x) = 1 + x + 3 x^2 + 7 x^3 . \) First, we determine its Hermite coefficients and then expand p(x) into Hermite series.
p[x_] = 1 + x + 3 x^2 + 7 x^3
coeffs = Table[
Integrate[HermiteH[n, x]*p[x]*Exp[-x^2], {x, -Infinity, Infinity}]/
Integrate[HermiteH[n, x]^2*Exp[-x^2], {x, -Infinity, Infinity}], {n, 0, 3}]
Out[1]= {5/2, 23/4, 3/4, 7/8}
coeffs.Table[HermiteH[n, x], {n, 0, 3}] // Expand
Out[2]= 1 + x + 3 x^2 + 7 x^3
For polynomials, you don't need to do any integrals to find the expansion. Take a polynomial p and a list basis containing the basis functions. Then define a function that takes these two, identifies the variable x, and solves for the coefficients in basis that make the two polynomials equal in terms of their CoefficientLists.
expandPoly[p_, basis_, x_] :=
# /. First@Solve[CoefficientList[#.basis, x] == #2, #] &[
Array["a", Length[#]], #] & @
PadRight[CoefficientList[p, x], Length[basis]]
expandPoly[1 + x + 3 x^2 + 7 x^3, HermiteH[Range[4] - 1, x], x]
Out[4]= {5/2, 23/4, 3/4, 7/8}
If you already know that you're only interested in a basis of HermiteH, you could incorporate that into the function and do away with the specification of the variable basis as follows:
expandPoly[p_, x_] := # /.
First @ Solve[
CoefficientList[#.HermiteH[Range[Length[#]] - 1, x],
x] == #2, #] &[Array["a", Length[#]], #] & @ CoefficientList[p, x]
expandPoly[1 + x + 3 x^2 + 7 x^3, x]
Out[6]= {5/2, 23/4, 3/4, 7/8}
a quick change of basis function for polynomials.
hermiteIP[f_, g_, x_] := With[{coeff = CoefficientList[f g, x]},
coeff.Table[1/2 (1 + (-1)^(-1 + n)) Gamma[n/2], {n, Length@coeff}]];
hermiteExpand[poly_, var_] /; PolynomialQ[poly, var] :=
Sum[hermiteIP[poly, HermiteH[n, var], var] H[n, var]/(Sqrt[Pi] 2^n n!),
{n, 0, Exponent[poly, var]}]
I used H[n, x] as a place holder for HermiteH[n, x].
hermiteExpand[(1 + x)^5, x]
Out[9]= 39/4 H[0, x] + 95/8 H[1, x] + 25/4 H[2, x] + 15/8 H[3, x] + 5/16 H[4, x] + 1/32 H[5, x]
hermiteExpand[(1 + x)^5, x] /. H -> HermiteH
Out[10]= 39/4 H[0, x] + 95/8 H[1, x] + 25/4 H[2, x] + 15/8 H[3, x] + 5/16 H[4, x] + 1/32 H[5, x]
% // Factor
Out[11]= 1/32 (312 H[0, x] + 380 H[1, x] + 200 H[2, x] + 60 H[3, x] + 10 H[4, x] + H[5, x])
Whenever I want to convert some polynomial expressed with respect to a certain basis in terms of another polynomial basis, my go-to algorithm is Salzer's algorithm. It's rather fast, since it relies only on recurrences. Here's a specialization of that algorithm for the case of monomial-Hermite conversion:
- Herbert E. Salzer, A recurrence scheme for converting from one orthogonal expansion into another. Communications of the ACM, CACM Homepage archive, 1973, Volume 16, Issue 11, Nov. 1973. https://doi.org/10.1145/35561 Pages 705-707 .
- H.E. Salzer, Orthogonal Polynomials Arising in the Numerical Evaluation of Inverse Laplace Transforms, Mathematics of Computation, 1955, Vol. 9, 164-177 (1955)
- C.P. Jeffreson, E.-P. Chow, Least squares coefficients for a quadrature formula for Laplace transform inversion, Journal of Computational and Applied Mathematic, 1978, Vol. 4, Issue 1, pp. 53-58. doi: 10.1016/0771-050x(78)90020-7
poly = 1 - x + 2 x^2 - 5 x^4;
c = CoefficientList[poly, x];
n = Length[c] - 1; Remove[a];
a[0, 2] = c[[n - 1]] + c[[n + 1]]/2;
a[1, 2] = c[[n]]; a[2, 2] = c[[n + 1]]/2;
Do[
a[0, k + 1] = c[[n - k]] + a[1, k]/2;
a[1, k + 1] = a[0, k] + a[2, k]/2;
Do[
a[m, k + 1] = (a[m + 1, k] + a[m - 1, k])/2,
{m, 2, k - 1}];
a[k, k + 1] = a[k - 1, k]/2;
a[k + 1, k + 1] = a[k, k]/2,
{k, 2, n - 1}];
ccof = Table[a[m, n], {m, 0, n}]
{1/8, -1, -3/2, 0, -5/8}
monomialToHermite[cofs_?VectorQ] := Module[{n = Length[cofs] - 1, a},
a[0, 0] = cofs[[n + 1]]; a[0, 1] = cofs[[n]];
a[1, 1] = cofs[[n + 1]]/2;
Do[ a[0, k + 1] = cofs[[n - k]] + a[1, k];
Do[ a[m, k + 1] = (m + 1) a[m + 1, k] + a[m - 1, k]/2, {m, k - 1}];
a[k, k + 1] = a[k - 1, k]/2; a[k + 1, k + 1] = a[k, k]/2, {k, n - 1}];
Table[a[m, n], {m, 0, n}]]
The algorithm as I presented it here uses an implicit two-dimensional array, a, to clearly show off the recurrence. The algorithm can be easily rewritten so that it uses only a pair or so of one-dimensional arrays, but I'll leave out that version for now.
Here's a test of Salzer's method:
monomialToHermite[{1, 1, 3, 7}]
Out[13]= {5/2, 23/4, 3/4, 7/8}
{1, 1, 3, 7}.x^Range[0, 3] == {5/2, 23/4, 3/4, 7/8}.HermiteH[Range[0, 3], x] // Expand
Out[14]= True
CoefficientList[(1 + x)^5, x] // monomialToHermite
Out[15]= {39/4, 95/8, 25/4, 15/8, 5/16, 1/32}
%.HermiteH[Range[0, 5], x] == (1 + x)^5 // Expand
Out[16]= True
As another variation, here's another method based on repeated greedy division:
Reap[Fold[Block[{q, r}, {q, r} = PolynomialQuotientRemainder[#1, #2, x];
Sow[q]; r] &, x^3 + x^2 + x + 1, HermiteH[Range[3, 0, -1], x]]][[-1, 1]] // Reverse
Out[18]= {3/2, 5/4, 1/4, 1/8}
Check:
%.HermiteH[Range[0, 3], x] == x^3 + x^2 + x + 1 // Expand
Out[19]= True
Example 1A: For arbitrary positive integer _p_∈ℕ, we consider the power function
\[ x_{+}^p = \begin{cases} x^p , & \ \mbox{ for} \quad x \ge 0, \\ 0, & \ \mbox{ for} \quad x \le 0. \end{cases} \]
This function does not belong to Hilbert space 𝔏²(−∞, ∞), but it belongs to 𝔏²(ℝ, _e_−x²) and 𝔏²(ℝ, _e_−x²/2). Expanding this function into Hermite series, we get
\[ x_{+}^p = \sum_{n\ge 0} a_{p,n} H_n (x) = \sum_{n\ge 0} b_{p,n} He_n (x) , \]
where
\[ a_{p,n} = \frac{1}{\| H_n \|^2} \left\langle x_{+}^p , H_n (x) \right\rangle = \frac{1}{2^n n!\,\sqrt{\pi}} \int_0^{\infty} x^p H_n (x) \, e^{-x^2} {\text d} x , \qquad n=0,1,2,\ldots , \]
\[ b_{p,n} = \frac{1}{\| He_n \|^2} \left\langle x_{+}^p , He_n (x) \right\rangle = \frac{1}{n!\,\sqrt{2\pi}} \int_0^{\infty} x^p He_n (x) \, e^{-x^2 /2} {\text d} x , \qquad n=0,1,2,\ldots , \]
Assuming p = 2_m_, _m_∈ℕ, we obtain
\[ x_{+}^{2m} = \sum_{n\ge 0} a_{2n} H_{2n} (x) = \sum_{n\ge 0} b_{2n} He_{2n} (x) , \]
where
\begin{align*} a_{2n} &= \frac{1}{2^{2n} (2n)! \,\sqrt{\pi}} \int_0^{\infty} x^{2m} e^{-x^2} H_{2n} (x) \,{\text d} x , \\ b_{2n} &= \frac{1}{(2n)! \,\sqrt{2\pi}} \int_0^{\infty} x^{2m} e^{-x^2 /2} He_{2n} (x) \,{\text d} x . \end{align*}
Using Rodrigues formulae
\begin{align*} H_{2n} (x) &= (-1)^{2n} e^{x^2} \frac{{\text d}^{2n}}{{\text d} x^{2n}} \, e^{-x^2} , \\ He_{2n} (x) &= (-1)^{2n} e^{x^2 /2} \frac{{\text d}^{2n}}{{\text d} x^{2n}} \, e^{-x^2/2} , \end{align*}
we get the values of coefficients
\begin{align*} a_{2n} &= \frac{1}{2^{2n} (2n)! \,\sqrt{\pi}} \int_0^{\infty} x^{2m} \frac{{\text d}^{2n}}{{\text d} x^{2n}} \,e^{-x^2} {\text d} x , \\ b_{2n} &= \frac{1}{(2n)! \,\sqrt{2\pi}} \int_0^{\infty} x^{2m} \frac{{\text d}^{2n}}{{\text d} x^{2n}} \,e^{-x^2 /2} {\text d} x . \end{align*}
Using integration by parts, we derive the formula:
\begin{align*} \int_0^{\infty} x^{2m} \frac{{\text d}^{2n}}{{\text d} x^{2n}} \,e^{-x^2} {\text d} x &= \frac{(2m)!}{(2m-2n)!} \int_0^{\infty} x^{2m-2n} e^{-x^2} {\text d} x , \\ \int_0^{\infty} x^{2m} \frac{{\text d}^{2n}}{{\text d} x^{2n}} \,e^{-x^2 /2} {\text d} x &= \end{align*}
So we continue:
\begin{align*} a_{2n} &= \frac{1}{2^{2n} (2n)! \,\sqrt{\pi}} \cdot \frac{(2m)!}{(2m-2n)!} \int_0^{\infty} x^{2m-2n} e^{-x^2} {\text d} x , \\ b_{2n} &= \frac{1}{(2n)! \,\sqrt{2\pi}} \cdot \end{align*}
Remembering the definition of the gamma function
\[ \Gamma (\nu ) = \int_0^{\infty} e^{-t} t^{\nu -1} {\text d} t , \qquad \Re\nu > 0, \]
we simplify the formulas
\begin{align*} a_{2n} &= \frac{1}{2^{2n} (2n)! \,\sqrt{\pi}} \cdot \frac{(2m)!}{(2m-2n)!} \cdot \Gamma \left( m-n + \frac{1}{2} \right) , \\ b_{2n} &= \frac{1}{(2n)! \,\sqrt{2\pi}} \cdot \end{align*}
Using the formula for half argument of gamma-function
\[ \Gamma \left( m - n + \frac{1}{2} \right) = \sqrt{\pi} \cdot \frac{(2m-2n)!\, 2^{2n}}{2^{2m} (m-n)!} , \]
we simplify coeffiocients further
\begin{align*} a_{2n} &= \frac{(2m)!}{2^{2m} (2n)!\,(m-n)!} , \\ b_{2n} &= \end{align*}
This allows us to derive the following expressions for Hermite expansions
\[ x^{2m}_{+} = \frac{(2m)!}{4^m} \sum_{n= 0}^m \frac{H_{2n} (x)}{(2n)! \,(m-n)!} \]
and
\[ x^{2m}_{+} = \sum_{n= 0}^m \]
Example 1B: For arbitrary positive integer _p_∈ℕ, we consider the power function
\[ x_{+}^p = \begin{cases} x^p , & \ \mbox{ for} \quad x \ge 0, \\ 0, & \ \mbox{ for} \quad x \le 0. \end{cases} \]
This function does not belong to Hilbert space 𝔏²(−∞, ∞), but it belongs to 𝔏²(ℝ, _e_−x²) and 𝔏²(ℝ, _e_−x²/2). Expanding this function into Hermite series, we get
\[ x_{+}^p = \sum_{n\ge 0} a_{p,n} H_n (x) = \sum_{n\ge 0} b_{p,n} He_n (x) , \]
where
\[ a_{p,n} = \frac{1}{\| H_n \|^2} \left\langle x_{+}^p , H_n (x) \right\rangle = \frac{1}{2^n n!\,\sqrt{\pi}} \int_0^{\infty} x^p H_n (x) \, e^{-x^2} {\text d} x , \qquad n=0,1,2,\ldots , \]
\[ b_{p,n} = \frac{1}{\| He_n \|^2} \left\langle x_{+}^p , He_n (x) \right\rangle = \frac{1}{n!\,\sqrt{2\pi}} \int_0^{\infty} x^p He_n (x) \, e^{-x^2 /2} {\text d} x , \qquad n=0,1,2,\ldots , \]
Assuming p = 2_m_+1, _m_∈ℕ, we obtain
\[ x_{+}^{2m+1} = \sum_{n\ge 0} a_{2n+1} H_{2n+1} (x) = \sum_{n\ge 0} b_{2n+1} He_{2n+1} (x) , \]
where
\begin{align*} a_{2n+1} &= \frac{1}{2^{2n+1} (2n+1)! \,\sqrt{\pi}} \int_0^{\infty} x^{2m+1} e^{-x^2} H_{2n+1} (x) \,{\text d} x , \\ b_{2n+1} &= \frac{1}{(2n+1)! \,\sqrt{2\pi}} \int_0^{\infty} x^{2m+1} e^{-x^2 /2} He_{2n+1} (x) \,{\text d} x . \end{align*}
Using Rodrigues formulae
\begin{align*} H_{2n+1} (x) &= (-1)^{2n+1} e^{x^2} \frac{{\text d}^{2n+1}}{{\text d} x^{2n+1}} \, e^{-x^2} , \\ He_{2n+1} (x) &= (-1)^{2n+1} e^{x^2 /2} \frac{{\text d}^{2n+1}}{{\text d} x^{2n+1}} \, e^{-x^2/2} , \end{align*}
we get the values of coefficients
\begin{align*} a_{2n+1} &= \frac{1}{2^{2n+1} (2n)! \,\sqrt{\pi}} \int_0^{\infty} x^{2m} \frac{{\text d}^{2n+1}}{{\text d} x^{2n+1}} \,e^{-x^2} {\text d} x , \\ b_{2n+1} &= \frac{1}{(2n+1)! \,\sqrt{2\pi}} \int_0^{\infty} x^{2m} \frac{{\text d}^{2n+1}}{{\text d} x^{2n+1}} \,e^{-x^2 /2} {\text d} x . \end{align*}
Example 2: The Hermite expansions for exponential functions follow from its generating function:
\[ e^{ax} = e^{a^2 /4} \sum_{n\ge 0} \frac{a^n}{n! \,2^n} \, H_n (x) , \qquad a\in \mathbb{C}, \quad x\in \mathbb{R} . \]
From this expansion, we have
\[ e^{2x} = e \sum_{n\ge 0} \frac{1}{n!}\, H_n (x) , \qquad e^{-2x} = e \sum_{n\ge 0} \frac{(-1)^n}{n!}\, H_n (x) . \]
Similarly, we get
\[ e^{-a^2 x^2} = \sum_{n\ge 0} \frac{(-1)^n a^{2n}}{n! \left( 1 + a^2 \right)^{n + 1/2} 2^{2n}}\, H_{2n} (x) . \]
Upon integration, we obtain Hermite expansion for the error function:
\[ \mbox{erf}(x) = \frac{2}{\sqrt{\pi}} \int_0^x e^{-t^2} {\text d}t = \frac{1}{\sqrt{2\pi}} \,\sum_{k\ge 0} \frac{(-1)^k}{k! \left( 2k+1 \right) 2^{3k}}\, H_{2k} (x) . \]
For hyperbolic functions, we have
\[ \cosh (2x) = e \sum_{k\ge 0} \frac{1}{(2k)!}\, H_{2k} (x) , \qquad \sinh (2x) = e \sum_{k\ge 0} \frac{1}{(2k+1)!} \, H_{2k+1} (x) . \]
Example 3: From the generating function, we immediately find expansions:
\[ e^{z^2} \cos (2xz) = \sum_{k\ge 0} (-1)^k \frac{z^{2k}}{(2k)!} \, H_{2k} (x) \tag{7.1} \]
and
\[ e^{z^2} \sin (2xz) = \sum_{k\ge 0} (-1)^k \frac{z^{2k+1}}{(2k+1)!} \, H_{2k+1} (x) \tag{7.2} \]
This allows us to expand cos(x) and sin(x into Hermite series.
\[ \cos (x) = e^{-1/4} \,\sum_{k\ge 0} \frac{(-1)^k}{2^{2k} \, (2k)!} \, H_{2k} (x) . \tag{7.3} \]
and
\[ \sin (x) = e^{-1/4} \,\sum_{k\ge 0} \frac{(-1)^k}{2^{2k+1} \, (2k+1)!} \, H_{2k+1} (x) . \tag{7.4} \]
Using Mathematica, we find coefficients in its expansion: Then we plot its approximation with 10 terms:
Integrate[Cos[x]*HermiteH[6, x]*Exp[-x^2], {x, -Infinity, Infinity}]
hercos[m_] = Exp[-1/4]* Sum[(-1)^k/(2^(2*k) *Factorial[2*k])*HermiteH[2*k, x], {k, 0, m}]
Plot[{Cos[x], hercos[14], hercos[7]}, {x, -15, 15}, PlotStyle -> {{Thick, Blue}, {Thick, Red}, {Thick, Orange}}, PlotRange -> {-2, 2}]
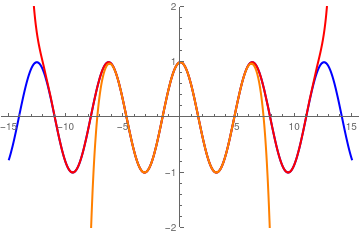
In particular,
\[ \cos (2x) = e^{-1} \sum_{k\ge 0} \frac{(-1)^k}{(2k)!}\, H_{2k} (x) . \]
For sine function, we have
\[ \sin (2kx) = e^{-k^2} \sum_{n\ge 0} (-1)^n \frac{H_{2n+1} (x)}{(2n+1)!} \, k^{2n+1} . \]
This expansion follows from
\[ \int_{-\infty}^{\infty} \sin (\mu x) \, e^{-x^2 /2} \,H_{2k+1} (x)\,{\text d}x = (-1)^k \sqrt{2\pi} \, e^{-\mu^2 /2} H_{2k+1} (\mu ) . \]
To check the answer, we plot the Hermite approximation with 10 and 30 terms along with function sin[2_x_):
sin10[x_] = Exp[-1]*Sum[(-1)^n *HermiteH[2*n + 1, x]/(2*n + 1)! , {n, 0, 10}];
sin[x_] = Exp[-1]*Sum[(-1)^n *HermiteH[2*n + 1, x]/(2*n + 1)! , {n, 0, 30}];
Plot[{Sin[2*x], sin[x]}, {x, 0, 2*Pi}, PlotStyle -> {Thick}, PlotLabel -> "Approximation with 30 terms"]
Plot[{Sin[2*x], sin10[x]}, {x, 0, 2*Pi}, PlotStyle -> {Thickness[0.01]}, PlotLabel -> "Approximation with 10 terms"]
From the formulas above, we derive the expansion for the Dirichlet kernel:
\[ \frac{\sin (ts)}{\pi s} = e^{-s^2 /4} \sum_{n\ge 1} (-1)^{n-1} \frac{s^{2n-2}}{\pi \left( 2n-1 \right) !} \, H_{2n-1} (t) , \qquad t\in\mathbb{R} \quad s\in \mathbb{R} \setminus \{ 0 \} . \]
For the Fejér kernel, we have
\[ \frac{1 - \cos (st)}{\pi s^2} = \frac{1 -e^{-s^2 /4} }{\pi s^2} + e^{-s^2 /4} \sum_{n\ge 1} (-1)^{n-1} \frac{s^{2n-2}}{4^n \pi \left( 2n \right) !}\, H_{2n} (t) \]
Example 4: Let us consider a characteristic function of a symmetric finite interval:
\[ \chi_{[-a,a]}(x) = \begin{cases} 1, & \quad \mbox{for} \quad |x| < a , \\ 0, & \quad\mbox{for} \quad |x| > a . \end{cases} \]
It is assumed that 𝑎 is a positive number. Since the given function is an even function, we get the Hermite expansion:
\[ \chi_{[-a,a]}(x) = \sum_{n\ge 0} c_{2n} H_{2n} (x) , \qquad c_{2n} = \frac{1}{2^{2n} (2n)!\,\sqrt{\pi}} \int_{-a}^a e^{-x^2} \,H_{2n} (x)\,{\text d} x . \]
Using the recurrence relation
\[ e^{-x^2} \,H_{2n} (x) = - \frac{\text d}{{\text d}x} \left[ e^{-x^2} \,H_{2n-1} (x) \right] , \qquad n\in \mathbb{N} ; \]
we evaluate coefficients:
\begin{align*} c_{2n} &= \frac{-1}{\| H_{2n} \|^2} \int_{-a}^a \frac{\text d}{{\text d}x} \left[ e^{-x^2} \,H_{2n-1} (x) \right] \\ &= \frac{-1}{\| H_{2n} \|^2} \left[ e^{-x^2} \,H_{2n-1} (x) \right]_{x=-a}^{x=a} = \frac{2}{2^{2n} (2n)!\,\sqrt{\pi}}\, e^{-a^2} \, H_{2n-1} (a) . \end{align*}
In particular,
\begin{align*} c_0 &= \frac{1}{\sqrt{\pi}} \int_{-a}^a e^{-x^2} {\text d} x = \mbox{erf}(a) , \\ c_2 &= \frac{1}{\sqrt{\pi}} \, e^{-a^2} \frac{a}{4} . \end{align*}
End of Example 4
Example 5: Consider piecewise step function
\[ \mbox{sign}(x-a) = \begin{cases} \phantom{-}1 , & \ a < x , \\ -1 , & \ x < a , \end{cases} \]
where 𝑎 is a positive number. We set 𝑎 = 0 for simplicitely, and expand the signum function into Hermite series:
\[ \mbox{sign}(x) = \frac{1}{\sqrt{\pi}} \sum_{k\ge 0} \frac{(-1)^k}{k!\left( 2k+1 \right) k^{2k}}\, H_{2k+1} (x) . \]
End of Example 5
Example 6: Consider piecewise step function, known as signum:
\[ \mbox{sign}(x-a) = \begin{cases} \phantom{-}1 , & \ a < x , \\ -1 , & \ x < a , \end{cases} \]
where 𝑎 is a positive number. We set 𝑎 = 0 for simplicitely, and expand the signum function into Hermite series:
\[ \mbox{sign}(x) = \sum_{k\ge 0} a_{2k+1} H_{2k+1} (x) , \]
where
\[ c_{2k+1} = \frac{1}{\| H_{2k+1} \|^2} \int_{-\infty}^{+\infty} e^{-x^2} \mbox{sign}(x)\, H_{2k+1} (x)\,{\text d} x = \frac{2}{2^{2k+1} (2k+1)!\,\sqrt{\pi}} \int_0^{\infty} e^{-x^2} H_{2k+1} (x)\,{\text d} x . \]
Using the recurrence relation
\[ e^{-x^2} H_{2k+1} (x) = - \frac{\text d}{{\text d}x} \left[ e^{-x^2} H_{2k} (x) \right] , \]
we find
\[ c_{2k+1} = \frac{2}{2^{2k+1} (2k+1)!\,\sqrt{\pi}} \, H_{2k} (0) \]
Taking into accound the formula
\[ H_{2k} (0) = (-1)^k \frac{(2k)!}{k!} , \]
we obtain
\[ c_{2k+1} = \frac{(-1)^k}{2^{2k} (2k+1)\,k! \,\sqrt{\pi}} \]
Then the Hermite expansion of the signum function becomes
\[ \mbox{sign}(x) = \frac{1}{\sqrt{\pi}} \sum_{k\ge 0} \frac{(-1)^k}{2^{2k} (2k+1)\,k!}\, H_{2k+1} (x) . \]
End of Example 6
Example 7:
There are known two sinc functions: unnormalized (which is used by Mathematica)
\[ \mbox{sinc}(x) = \frac{\sin x}{x} \]
and normalized one. The Hermite series for the normalized sinc function is
\[ \mbox{sinc}(x) = \frac{\sin \pi x}{\pi x} = \sqrt{\frac{2}{\pi}} \sum_{n\ge 0} \psi_n (x) \,\frac{\sqrt{(2n)!}}{2^n \pi^{1/4}} \,\sum_{k=0}^n \frac{(-1)^k}{(2k)!\left( n-k \right)!} \,2^{3k-1/2} \,\gamma \left( k + \frac{1}{2}, \frac{\pi^2}{2} \right) , \]
where ψn(x) are Hermite functions \eqref{EqHermite.3} and Gamma[ν, 0, _x_] denotes the lower incomplete gamma function:
\[ \gamma (\nu , x) = \int_0^x t^{\nu -1} e^{-t} {\text d}t . \]
| 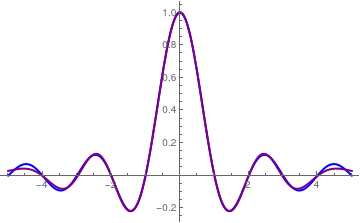 | | | We plot Hermite approximation (in purple) with six terms for the sinc function (in blue):Plot[{Sinc[Pi*x], Sum[Sqrt[2/ Pi] Sqrt[(2 n)!]/(2^n Pi^(1/4)) Sum[(-1)^ k/((2 k)! (n - k)!) 2^(3 k - 1/2) Gamma[k + 1/2, 0, Pi^2/2], {k, 0, n}]* HermiteH[2*n, x]* Exp[-x^2 /2]/2^n /Pi^(1/4)/Sqrt[(2*n)!], {n, 0, 6}]}, {x, -5, 5}, PlotRange -> All, Evaluated -> True, PlotStyle -> {{Thick, Blue}, {Thick, Purple}}] |
| --------------------------------------------------------------------------------------- | | | ------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------ |
| | | We plot Hermite approximation (in purple) with six terms for the sinc function (in blue):Plot[{Sinc[Pi*x], Sum[Sqrt[2/ Pi] Sqrt[(2 n)!]/(2^n Pi^(1/4)) Sum[(-1)^ k/((2 k)! (n - k)!) 2^(3 k - 1/2) Gamma[k + 1/2, 0, Pi^2/2], {k, 0, n}]* HermiteH[2*n, x]* Exp[-x^2 /2]/2^n /Pi^(1/4)/Sqrt[(2*n)!], {n, 0, 6}]}, {x, -5, 5}, PlotRange -> All, Evaluated -> True, PlotStyle -> {{Thick, Blue}, {Thick, Purple}}] |
| --------------------------------------------------------------------------------------- | | | ------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------ |
End of Example 7
Example 8: Let us consider the Heaviside function:
\[ H(t) = \begin{cases} 1, & \ \mbox{ for} \quad t > 0 , \\ 1/2, & \ \mbox{ for} \quad t = 0, \\ 0, & \ \mbox{ for} \quad t < 0. \end{cases} \]
Upon expanding it into Hermite series, we obtain
\[ H(t) = \sum_{n\ge 0} c_n H_n (t) , \]
where
\[ c_n = \frac{1}{\| H_n \|_w^2} \,\left\langle f(x), H_n (x) \right\rangle = \frac{1}{2^n n! \,\sqrt{\pi}}\, \int_{-\infty}^{\infty} f(x)\, e^{-x^2} \,H_n (x) \,{\text d} x = \frac{n-2}{n \Gamma \left( - \frac{n}{2} \right) n!} \, -@F_1 \left( 1, \frac{1-n}{2}, \frac{1}{2} , 1 \right) . \]
Mathematica expresses the value of the integral through hypergeometric function:
Integrate[Exp[-x^2]*HermiteH[n, x], {x, 0, Infinity}]
2^n (-2 + n) Sqrt[\[Pi]] Hypergeometric2F1[1, (1 - n)/2, 1/2, 1]
Since the gamma function is unbounded at negative integer values, we get
\[ H(t) = \sum_{k\ge 0} c_{2k+1} H_{2k+1} (t) , \]
where
\[ c_{2k+1} = \frac{k-1}{k\, (2k+1)! \Gamma \left( - k - \frac{1}{2} \right)}\,_2F_1 \left( 1, -k , \frac{1}{2} , 1 \right) = \frac{(k-1)\,(-1)^{k+1}}{k\,(2k)!!\,\sqrt{\pi} 2^{k+1}} \,_2F_1 \left( 1, -k , \frac{1}{2} , 1 \right) , \qquad k=0,1,2,\ldots . \]
End of Example 8
Example 9: Let us expand into Hermite's series the following piecewise continuous function:
\[ f(x) = \begin{cases} x, & \ \mbox{ for} \quad 0 < x < 1, \\ (x-1)^2 , & \ \mbox{ for} \quad 1 < x < 2, \\ 0, & \ \mbox{ otherwise}. \end{cases} \]
The corresponding coefficients _c_n of the Hermite series
\[ f(x) = \sum_{n\ge 0} c_n H_n (x) \]
can be determined only numerically
\[ c_n = \frac{1}{\| H_n \|_w^2} \,\left\langle f(x), H_n (x) \right\rangle = \frac{1}{2^n n! \,\sqrt{\pi}}\, \int_{-\infty}^{\infty} f(x)\, e^{-x^2} \,H_n (x) \,{\text d} x = \frac{1}{2^n n! \,\sqrt{\pi}}\left[ \int_0^1 e^{-x^2} \,x \,{\text d} x + \int_1^2 e^{-x^2} \,(x-1)^2 \,{\text d} x \right] . \]
Mathematica is very helpful wuth their evaluations. Despite that Mathematica provides analytic formulas,
Integrate[x*Exp[-x^2]*HermiteH[n, x], {x, 1, 2}]
-((Gamma[(1 - n)/2] Hypergeometric1F1[1 + n/2, 5/2, -1])/( 3 Gamma[-n])) - ( 2^(-2 + n) Sqrt[\[Pi]] (-1 + Hypergeometric1F1[1/2 (-1 + n), -(1/2), -1]))/ Gamma[3/2 - n/2]
Integrate[(x - 1)^2*Exp[-x^2]*HermiteH[n, x], {x, 1, 2}]
they are too complicated for numerical utilization. Therefore, we prefer to use numerical integration:
Do[c[n] == Pi^(-1/2)/(2^n * n!)*(NIntegrate[ x*Exp[-x^2]*HermiteH[n, x], {x, 0, 1}] + NIntegrate[(x - 1)^2*Exp[-x^2]*HermiteH[n, x], {x, 1, 2}]), {n, 0, 10}]
S30[x_] = Sum[c[n]*HermiteH[n, x], {n, 0, 30}];
f[x_] = Piecewise[{{x, 0 < x < 1}, {(x - 1)^2, 1 < x < 2}, {0, x > 2}}]
Plot[{f[x], S30[x]}, {x, 0, 3}, PlotStyle -> {{Thickness[0.01], Blue}, {Thick, Red}}, PlotRange -> {-0.2, 1.2}]
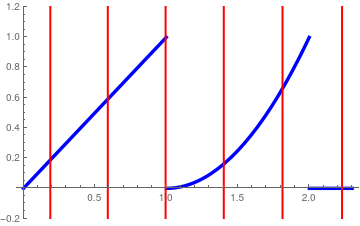
Hermite approximation with 30 terms (in red).
Tempered distributions
Since Hermite and Chebyshev--Hermite polynomials are defined on real axis ℝ, they are unbounded and therefore do not belong to Hilbert space 𝔏²[ℝ]. However, this Hilbert space contains a subspace of rapidly decreasing functions that is commonly called the Schwartz space and it is denoted by 𝒮(ℝ) or S(ℝ). Then polynomials can be considered as functionals on this space.
A smooth function f : ℝ ↦ ℝ on the Euclidean space ℝ has rapidly decreasing derivatives if the absolute value of the product of any derivative of f with with any polynomial function is a bounded function. The set of all rapidly decreasing function is denoted by 𝒮(ℝ) or S(ℝ).
A tempered distribution on ℝ is a continuous linear functional on the Schwartz space 𝒮(ℝ). The set of tempered distributions is denoted by 𝒮'(ℝ) or 𝒮*(ℝ) or S'(ℝ).
A Hermite polynomial or Chebyshev--Hermite polynomial provides an example of tempered distribution, so they belong to 𝒮*(ℝ). On the other hand, every Hermite function or Chebyshev--Hermite function belongs to 𝒮(ℝ).
Every distribution T : 𝒮(ℝ) → ℂ can be written as
\[ T(\phi ) = \langle T, \phi \rangle = \int_{\mathbb{R}} \phi (x)\,T(x)\,{\text d}x \]
for some function T(x) and any test function ϕ from the Schwartz space.
Theorem 6 (Fourier transform is linear automorphism of Schwartz space): Fourier transform is well defined on all smooth functions on ℝ with rapidly decreasing derivatives and indeed constitutes a linear isomorphism from the Schwartz space 𝒮(ℝ) to itself.
For f ∈ 𝔏²(ℝ), its Fourier transform can be expanded as
\begin{equation} \label{EqHermite.8} f^F (\xi ) = 𝔉_{x\to\xi}\left[ f (x) \right] (\xi ) = \mbox{P.V.} \int_{-\infty}^{\infty} e^{{\bf j}x\xi} f (x)\,{\text d}x = \sqrt{2\pi} \sum_{n\ge 0} {\bf j}^n c_n \psi_n (x) , \end{equation}
where coefficients _c_n are determined from Eq.\eqref{EqHermite.9}.
Theorem 7: Every test function ϕ∈𝒮(ℝ) posesses Fourier--Hermite expansion
\[ \phi (x) = \sum_{n\ge 0} c_n \psi_n (x) , \qquad c_n = \langle \phi \,\vert\, \psi_n \rangle , \tag{T.1} \]
or
\[ \phi (x) = \sum_{n\ge 0} d_n h_n (x) , \qquad d_n = \langle \phi \,\vert\, h_n \rangle . \tag{T.2} \]
Moreover,
\[ \sum_{n\ge 0} c_n n^p < \infty , \qquad \sum_{n\ge 0} d_n n^p < \infty \tag{T.3} \]
for any p ∈ ℕ = {0, 1, 2, …}. Conversely, if _c_n or _d_n satisfies (T.3), then the right-hand side of Eq.(T.1) or (T.2) converges to some function ϕ∈𝒮(ℝ).
Similarly, for trmpered distribution _T_∈𝒮'(ℝ), the series
\[ T(x) = \sum_{n\ge 0} c_n \psi_n (x) = \sum_{n\ge 0} d_n h_n (x) \tag{T.4} \]
weakly converge and
\[ |c_n | < C\,|n|^k , \qquad |d_n | < C\,|n|^k \tag{T.5} \]
for some positive constants C and k.
Conversely, if _c_n or _d_n satisfies (T.5), then the right-hand side of (T.4) converges to some _T_∈𝒮'(ℝ).
The corresponding expansion for Dirac delta function is
\[ \delta (x-a) = \frac{1}{\sqrt{\pi}}\,e^{-(x^2 + a^2 )/2} \, \sum_{n\ge 0} \frac{1}{2^n \, n!}\, H_n (x)\, H_n (a) = \sum_{n\ge 0} \psi_n (x)\,\psi_n (a) . \]
From this relation, it follows
\[ \sum_{n\in\mathbb{N}} \psi_{2n} (x)\,\psi_{2n} (a) = \frac{1}{2} \left[ \delta (x-a) + \delta (x+a) \right] , \qquad \sum_{n\in\mathbb{N}} \psi_{2n+1} (x)\,\psi_{2n+1} (a) = \frac{1}{2} \left[ \delta (x-a) - \delta (x+a) \right] , \]
and
\[ \sum_{n\in\mathbb{N}} \psi_{4n} (x)\,\psi_{4n} (a) = \frac{1}{2\sqrt{2\pi}}\,\cos (ax) + \frac{1}{4} \left[ \delta (x-a) + \delta (x+a) \right] , \qquad \sum_{n\in\mathbb{N}} \psi_{4n+1} (x)\,\psi_{4n+1} (a) = \frac{1}{2\sqrt{2\pi}}\,\sin (ax) + \frac{1}{4} \left[ \delta (x-a) - \delta (x+a) \right] . \]
Then for even and odd functions, we get
\[ \frac{f(x) + f(-x)}{2} = \sum_{n\in\mathbb{N}} c_{2n} \psi_{2n} (x) = \sum_{n\in\mathbb{N}} c_{4n} \psi_{4n} (x) + \sum_{n\in\mathbb{N}} c_{4n+2} \psi_{4n+2} (x) \]
and
\[ \frac{f(x) - f(-x)}{2} = \sum_{n\in\mathbb{N}} c_{2n+1} \psi_{2n+1} (x) . \]
Example 1T:
End of Example 1T
Example 2T: For arbitrary positive real p, we consider the general power function
\[ x_{+}^p = \begin{cases} x^p , & \ \mbox{ for} \quad x \ge 0, \\ 0, & \ \mbox{ for} \quad x \le 0. \end{cases} \]
Expanding this function into Hermite series, we get
\[ x_{+}^p = \sum_{n\ge 0} a_{p,n} H_n (x) = \sum_{n\ge 0} b_{p,n} He_n (x) = \sum_{n\ge 0} c_{p,n} \psi_n (x) = \sum_{n\ge 0} d_{p,n} h_n (x) , \]
where
\[ a_{p,n} = \frac{1}{\| H_n \|^2} \left\langle x_{+}^p , H_n (x) \right\rangle = \frac{1}{2^n n!\,\sqrt{\pi}} \int_0^{\infty} x^p H_n (x) \, e^{-x^2} {\text d} x , \qquad n=0,1,2,\ldots , \]
\[ b_{p,n} = \frac{1}{\| He_n \|^2} \left\langle x_{+}^p , He_n (x) \right\rangle = \frac{1}{n!\,\sqrt{2\pi}} \int_0^{\infty} x^p He_n (x) \, e^{-x^2 /2} {\text d} x , \qquad n=0,1,2,\ldots , \]
and
\[ c_{p,n} = \left\langle x_{+}^p , \psi_n (x) \right\rangle = \int_0^{\infty} x^p \psi_n (x) \,{\text d} x = \left( 2^n n!\,\sqrt{\pi} \right)^{-1/2} \int_0^{\infty} x^p H_n (x) \, e^{-x^2 /2} {\text d} x , \qquad n=0,1,2,\ldots ; \]
\[ d_{p,n} = \left\langle x_{+}^p , h_n (x) \right\rangle = \int_0^{\infty} x^p h_n (x) \,{\text d} x = \left( n!\,\sqrt{2\pi} \right)^{-1/2} \int_0^{\infty} x^p H_n (x) \, e^{-x^2 /4} {\text d} x , \qquad n=0,1,2,\ldots . \]
First, we check some initial values with Mathematica:
Assuming[p > 0, Integrate[x^p*Exp[-x^2], {x, 0, Infinity}]]
1/2 Gamma[(1 + p)/2]
\[ c_{p,0} = \frac{1}{\sqrt{\pi}}\,\left\langle x_{+}^p , H_0 (x) \right\rangle = \frac{1}{\sqrt{\pi}} \int_0^{\infty} x^p H_0 (x) \,{\text d} x = \frac{1}{2\sqrt{\pi}}\,\Gamma \left( \frac{1+p}{2} \right) . \]
Assuming[p > 0, Integrate[x^p*Exp[-x^2]*HermiteH[1, x], {x, 0, Infinity}]]/2/ Sqrt[Pi]
Gamma[1 + p/2]/(2 Sqrt[\[Pi]])
\[ c_{p,1} = \frac{1}{\| H_1 \|^2}\,\left\langle x_{+}^p , H_1 (x) \right\rangle = \frac{1}{2\sqrt{\pi}} \int_0^{\infty} x^p H_1 (x) \,{\text d} x = \frac{1}{2\sqrt{\pi}}\,\Gamma \left( \frac{2+p}{2} \right) . \]
Assuming[p > 0, Integrate[x^p*Exp[-x^2]*HermiteH[2, x], {x, 0, Infinity}]]/2^3/ Sqrt[Pi]
(p Gamma[(1 + p)/2])/(8 Sqrt[\[Pi]])
\[ c_{p,2} = \frac{1}{\| H_2 \|^2}\,\left\langle x_{+}^p , H_2 (x) \right\rangle = \frac{1}{2^3 \sqrt{\pi}} \int_0^{\infty} x^p H_2 (x) \,{\text d} x = \frac{p}{8\sqrt{\pi}}\,\Gamma \left( \frac{1+p}{2} \right) . \]
Assuming[p > 0, Integrate[x^p*Exp[-x^2]*HermiteH[3, x], {x, 0, Infinity}]]/2^3/6/ Sqrt[Pi]
((-1 + p) p Gamma[p/2])/(48 Sqrt[\[Pi]])
\[ c_{p,3} = \frac{1}{\| H_3 \|^2}\,\left\langle x_{+}^p , H_3 (x) \right\rangle = \frac{1}{2^3 3!\,\sqrt{\pi}} \int_0^{\infty} x^p H_3 (x) \,{\text d} x = \frac{p\left( p-1 \right)}{48\sqrt{\pi}}\,\Gamma \left( \frac{p}{2} \right) = \frac{p-1}{24\sqrt{\pi}}\,\Gamma \left( \frac{p}{2} +1 \right) . \]
Multipying the recurrence
\[ H_{n+1} (x) = 2x\,H_n (x) - 2n\,H_{n-1} (x) \]
by _x_p and integrating, we obtain
\[ \int_0^{\infty} x^p H_{n+1} (x)\, e^{-x^2} {\text d}x = 2\int_0^{\infty} x^{p+1} H_n (x) \, e^{-x^2} {\text d}x - 2n\,\int_0^{\infty} x^p H_{n-1} (x) \, e^{-x^2} {\text d}x . \]
This yields the recurrence:
\[ c_{p, n+1} = 2 c_{p+1,n} - 2n\,c_{p, n-1} , \qquad n=1,2,\ldots . \]
Similarly, multiplying the recurrence
\[ H_{n+1} (x) = 2x\,H_n (x) - H'_{n} (x) \]
by _x_p+1 and integrating, we obtain
End of Example 2T
Example 3T: Consider piecewise step function, known as signum:
\[ \mbox{sign}(x-a) = \begin{cases} \phantom{-}1 , & \ a < x , \\ -1 , & \ x < a , \end{cases} \]
where 𝑎 is a positive number. We set 𝑎 = 0 for simplicitely, and expand the signum function into Hermite series:
\[ \mbox{sign}(x) = \sum_{k\ge 0} a_{2k+1} H_{2k+1} (x) , \]
where
\[ c_{2k+1} = \frac{1}{\| H_{2k+1} \|^2} \int_{-\infty}^{+\infty} e^{-x^2} \mbox{sign}(x)\, H_{2k+1} (x)\,{\text d} x = \frac{2}{2^{2k+1} (2k+1)!\,\sqrt{\pi}} \int_0^{\infty} e^{-x^2} H_{2k+1} (x)\,{\text d} x . \]
Using the recurrence relation
\[ e^{-x^2} H_{2k+1} (x) = - \frac{\text d}{{\text d}x} \left[ e^{-x^2} H_{2k} (x) \right] , \]
we find
\[ c_{2k+1} = \frac{2}{2^{2k+1} (2k+1)!\,\sqrt{\pi}} \, H_{2k} (0) \]
Taking into accound the formula
\[ H_{2k} (0) = (-1)^k \frac{(2n)!}{n!} , \]
we obtain
\[ c_{2k+1} = \frac{(-1)^k}{2^{2k} (2k+1)\,k! \,\sqrt{\pi}} \]
Then the Hermite expansion of the signum function becomes
\[ \mbox{sign}(x) = \frac{1}{\sqrt{\pi}} \sum_{k\ge 0} \frac{(-1)^k}{2^{2k} (2k+1)\,k!}\, H_{2k+1} (x) . \]
End of Example 5
Signum function is related to the Heaviside function:
\[ H(x) = \frac{1}{2}\left[ \mbox{sign}(x) +1 \right] . \]
It is known that signum is the Fourier transform of reciprocal of x:
\[ 𝔉_{x\to\xi}\left[ \mbox{P.V.} \frac{1}{x} \right] (\xi ) = \mbox{P.V.} \int_{-\infty}^{+\infty} e^{{\bf j} x\xi} \frac{{\text d}x}{x} = {\bf j} \pi\,\mbox{sign}(\xi ) . \]
Integrate[Sin[t*x]/x, {x, 0, Infinity}]
(\[Pi] t)/(2 Sqrt[t^2])
Since the Fourier transform of the Heaviside function is known to be
\[ 𝔉_{x\to\xi}\left[ H(x) \right] (\xi ) = \int_0^{\infty} e^{{\bf j} x\xi} {\text d}x = \frac{\bf j}{\xi + {\bf j} 0} , \]
we can establish the well-known Lippmann-Schwinger formula:
\[ \frac{1}{x\pm {\bf j}0} = \mbox{P.V.} \frac{1}{x} \mp{\bf j}\,\delta (x) . \]
Example 4T: Let us consider the Heaviside function:
\[ H(t) = \begin{cases} 1, & \ \mbox{ for} \quad t > 0 , \\ 1/2, & \ \mbox{ for} \quad t = 0, \\ 0, & \ \mbox{ for} \quad t < 0. \end{cases} \]
Upon expanding it into Hermite series, we obtain
\[ H(t) = \sum_{n\ge 0} c_n H_n (t) , \]
where
\[ c_n = \frac{1}{\| H_n \|_w^2} \,\left\langle f(x), H_n (x) \right\rangle = \frac{1}{2^n n! \,\sqrt{\pi}}\, \int_{-\infty}^{\infty} f(x)\, e^{-x^2} \,H_n (x) \,{\text d} x = \frac{n-2}{n \Gamma \left( - \frac{n}{2} \right) n!} \, -@F_1 \left( 1, \frac{1-n}{2}, \frac{1}{2} , 1 \right) . \]
Mathematica expresses the value of the integral through hypergeometric function:
Integrate[Exp[-x^2]*HermiteH[n, x], {x, 0, Infinity}]
2^n (-2 + n) Sqrt[\[Pi]] Hypergeometric2F1[1, (1 - n)/2, 1/2, 1]
Since the gamma function is unbounded at negative integer values, we get
\[ H(t) = \sum_{k\ge 0} c_{2k+1} H_{2k+1} (t) , \]
where
\[ c_{2k+1} = \frac{k-1}{k\, (2k+1)! \Gamma \left( - k - \frac{1}{2} \right)}\,_2F_1 \left( 1, -k , \frac{1}{2} , 1 \right) = \frac{(k-1)\,(-1)^{k+1}}{k\,(2k)!!\,\sqrt{\pi} 2^{k+1}} \,_2F_1 \left( 1, -k , \frac{1}{2} , 1 \right) , \qquad k=0,1,2,\ldots . \]
- Boyd, J. P., The Rate of Convergence of Hermite Function Series, Mathematics of Computation, Vol. 35, No. 152, Oct., 1980, American Mathematical Society. doi: 10.2307/2006396
- Davis, Philip, Interpolation and Approximation, Dover, 1975, ISBN: 0-486-62495-1, LC: QA221.D33
- Kagawa, T.: Hermite function expansions of Heaviside function. Journal of Pseudo-Differential Operators and Applications, 2015, 6, No. 1, pp. 21–32.
- Kagawa, T. & Yoshino, K., The Hermite expansion of the characteristic functions, Journal of Pseudo-Differential Operators and Applications, 2017, Vol. 8, No. 2, pp. 255–273. https://doi.org/10.1007/s11868-017-0188-x
- Lewandowska, S. and Mikusiński J., On Hermite expansions of 1/x and 1/|x|, Annales Polonici mathematici, 1974, Vol. 28, pp. 167--172.
- Muckenhoupt, B., Mean convergence of Hermite and Laguaerre series, Transactions of the American Mathematical Society, 1970, Volume 147, February, pp. 419--431.
- Sadlok, Z. On Hermite expansion of x+p, Annales Polonici mathematici, 1980, 38, Issue 2, pp. 159--162. doi: 10.4064/ap-38-2-159-162
- Sadlok, Z. and Tyc, Z., On Hermite expansions of _x_k and ln |x|, Annales Polonici mathematici, 1977, Vol. 34, pp. 63--68.
Return to Mathematica page
Return to the main page (APMA0340)
Return to the Part 1 Matrix Algebra
Return to the Part 2 Linear Systems of Ordinary Differential Equations
Return to the Part 3 Non-linear Systems of Ordinary Differential Equations
Return to the Part 4 Numerical Methods
Return to the Part 5 Fourier Series
Return to the Part 6 Partial Differential Equations
Return to the Part 7 Special Functions