DGGS - Flexible Structures (original) (raw)
| We investigate polyhedra and frameworks which are flexible or infinitesimally flexible, and problems related to the bellows conjecture. | |
 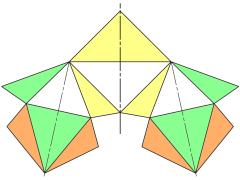 R. Connelly constructed a flexible polygonal embedding of the 2-sphere into the E³ in 1977. A simplified flexing sphere was presented by K. Steffen in 1978. The unfolding of Steffen's polyhedra is given above. Note that both flexing spheres are compound of Bricard octahedra which all have self-intersections. R. Connelly constructed a flexible polygonal embedding of the 2-sphere into the E³ in 1977. A simplified flexing sphere was presented by K. Steffen in 1978. The unfolding of Steffen's polyhedra is given above. Note that both flexing spheres are compound of Bricard octahedra which all have self-intersections.  R. Bricard proved in 1897 that there are three types of flexible octahedra in E³. Here both flat poses of a Bricard octahedron of type 3 are illustrated. Note that Bricard octahedra keep their volume constant during the flex. This is due to the Bellows Conjecture which was proven by I. Sabitov in the year 1996. R. Bricard proved in 1897 that there are three types of flexible octahedra in E³. Here both flat poses of a Bricard octahedron of type 3 are illustrated. Note that Bricard octahedra keep their volume constant during the flex. This is due to the Bellows Conjecture which was proven by I. Sabitov in the year 1996. |
Flexible Polyhedra and Frameworks in Different Spaces This project is part of an international cooperation funded by the Austrian Science Fund (FWF) and the Russian Foundation for Basic Research (RFBR). FWF grant no. I 408-N13 RFBR grant no. 10-01-91000-ANF Duration: 2010-2013 FWF-Funding: € 298 966.50 Austrian Members Hellmuth Stachel, TU Vienna, Institute of Discrete Mathematics and Geometry Email: stachel@geometrie.tuwien.ac.at Hans-Peter Schröcker, University of Innsbruck, Unit Geometry and CAD Email: hans-peter.schroecker@uibk.ac.at Georg Nawratil, TU Vienna, Institute of Discrete Mathematics and Geometry Email: nawratil@geometrie.tuwien.ac.at Ekaterina Zaputryaeva, TU Vienna, Institute of Discrete Mathematics and Geometry Email: katya@geometrie.tuwien.ac.at Russian Members Idzhad Sabitov, Moscow State University, Faculty of Mechanics and Mathematics Email: isabitov@mail.ru Victor Alexandrov, Novosibirsk State University, Sobolev Institute of Mathematics Email: alex@math.nsc.ru Sergey Mikhalev, Moscow State University, Faculty of Mechanics and Mathematics Email: mikhalev@bk.ru Dmitriy Slutskiy, Novosibirsk State University, Sobolev Institute of Mathematics Email: dmitry.slutsky@gmail.com Aims and Scope The joint project is devoted to the study of flexible structures, like polyhedra and overconstrained frameworks. Which conditions are necessary and sufficient for flexibility, which metric or combinatorial properties must change or remain constant under flexing? To recall, a polyhedron - or more precisely, a polyhedral surface - is said to be flexible if its spatial shape can be changed continuously due to changes of its dihedral angles only, i.e., in such a way that every face remains congruent to itself during the flex. The question whether the edge lengths of a framework determine its planar or spatial shape uniquely, is also important for many engineering applications - not only for mechanical or constructional engineers, but also for biologists in protein modelling or for the analysis of isomers in chemistry. Project Publications Journal Articles V. Alexandrov: The Dehn invariants of the Bricard octahedra. Journal of Geometry 99 (1-2) 1-13 (2010) arXiv:0901.2989v1 [math.MG] DOI: 10.1007/s00022-011-0061-7 V. Alexandrov: New manifestations of the Darboux's rotation and translation fields of a surface. New Zealand Journal of Mathematics 40 59-65 (2010) G. Nawratil: Flexible octahedra in the projective extension of the Euclidean 3-space. Journal for Geometry and Graphics 14 (2) 147-169 (2010) V. Alexandrov: Algebra versus analysis in the theory of flexible polyhedra. Aequationes Mathematicae 79 (3) 229-235 (2010) arXiv:0902.0186v2 [math.MG] DOI: 10.1007/s00010-010-0024-3 Erratum: Aequationes Mathematicae 81 (1) 199 (2011) DOI: 10.1007/s00010-010-0065-7 D. Slutskiy: An infinitesimally nonrigid polyhedron with nonstationary volume in the Lobachevsky 3-space. Siberian Mathematical Journal 52 (1) 131-138 (2011) arXiv:1002.3884v3 [math.MG] DOI: 10.1134/S0037446606010149 G. Nawratil: Reducible compositions of spherical four-bar linkages with a spherical coupler component. Mechanism and Machine Theory 46 (5) 725-742 (2011) DOI: 10.1016/j.mechmachtheory.2010.12.004 G. Nawratil: Self-motions of TSSM manipulators with two parallel rotary axes. ASME Journal of Mechanisms and Robotics 3 (3) 031007 (2011) DOI: 10.1115/1.4004030 H. Stachel:What lies between the flexibility and rigidity of structures. Serbian Architectural Journal 3 (2) 102-115 (2011) H. Stachel:On the Rigidity of Polygonal Meshes. South Bohemia Mathematical Letters 19 (1) 6-17 (2011) G. Nawratil: Planar Stewart Gough platforms with a type II DM self-motion. Journal of Geometry 102 (1) 149-169 (2011) DOI: 10.1007/s00022-012-0106-6 V. Alexandrov: On a differential test of homeomorphism, found by N.V. Efimov (in Russian). Contemporary Problems of Mathematics and Mechanics (Sovremennye Problemy Matematiki i Mekhaniki) 6 (2) 18-26 (2011) ISBN: 978-5-211-05652-7, arXiv:1010.3637v1 [math.DG] I.Kh. Sabitov: Algebraic methods for solution of polyhedra. Russian Math. Surveys 66 (3) 445-505 (2011) DOI: 10.1070/RM2011v066n03ABEH004748 V. Alexandrov and R. Connelly:Flexible suspensions with a hexagonal equator. Illinois Journal of Mathematics 55 (1) 127-155 (2011) D.I. Sabitov and I.Kh. Sabitov:Volume Polynomials for Some Polyhedra in Spaces of Constant Curvature (in Russian). Modelling and analysis of Information systems 19 (6) 161-169 (2012) G. Nawratil: Reducible compositions of spherical four-bar linkages without a spherical coupler component. Mechanism and Machine Theory 49 87-103 (2012) DOI: 10.1016/j.mechmachtheory.2011.11.003 G. Nawratil: Necessary conditions for type II DM self-motions of planar Stewart Gough platforms. Journal for Geometry and Graphics 16 (2) 139-151 (2012) V. Alexandrov:Around the A. D. Alexandrov's theorem on a characterization of a sphere (in Russian). Siberian Electronic Mathematical Reports 9 639-652 (2012) G. Nawratil: Comments on "Architectural singularities of a class of pentapods". Letter to the Editor, Mechanism and Machine Theory 57 139 (2012) DOI: 10.1016/j.mechmachtheory.2012.06.007 H. Stachel:A Flexible Planar Tessellation with a Flexion Tiling a Cylinder of Revolution. Journal for Geometry and Graphics 16 (2) 153-170 (2012) D. Slutskiy:A necessary flexibility condition of a nondegenerate suspension in Lobachevsky 3-space. Sbornik: Mathematics 204 (8) 1195-1214 (2013) arXiv:1208.2793v1 [math.MG] DOI: 10.1070/SM2013v204n08ABEH004336 G. Nawratil: Types of self-motions of planar Stewart Gough platforms. Meccanica 48 (5) 1177-1190 (2013) DOI: 10.1007/s11012-012-9659-6 G. Hegedüs, J. Schicho and H.-P. Schröcker: Factorization of Rational Curves in the Study Quadric and Revolute Linkages. Mechanism and Machine Theory 69 142�152 (2013) arXiv:1202.0139v4 [math.RA] DOI: 10.1016/j.mechmachtheory.2013.05.010 G. Hegedüs, J. Schicho and H.-P. Schröcker: The Theory of Bonds: A New Method for the Analysis of Linkages. Mechanism and Machine Theory 70 407-424 (2013) arXiv:1206.4020v5 [math.AG] DOI: 10.1016/j.mechmachtheory.2013.08.004 D. Slutskiy: A ratio between the lengths of the edges of the equator of a flexible suspension in the Lobachevsky 3-space. Doklady Mathematics 87 (2) 140-143 (2013) DOI: 10.1134/S106456241302004X E. Zaputryaeva: Flexions of equilateral polygons with keeping the index (in Russian). Modelling and analysis of Information systems 20 (1) 138-159 (2013) G. Nawratil: On equiform Stewart Gough platforms with self-motions. Journal for Geometry and Graphics 17 (2) 163-175 (2013) G. Nawratil: On elliptic self-motions of planar projective Stewart Gough platforms. Transactions of the Canadian Society for Mechanical Engineering 37 (4) 1057-1071 (2013) V. Alexandrov:Continuous deformations of polyhedra that do not alter the dihedral angles. Geometriae Dedicata 170 (1) 335-345 (2014) DOI: 10.1007/s10711-013-9884-8 G. Nawratil: Correcting Duporcq's theorem. Mechanism and Machine Theory 73 282�295 (2014) DOI: 10.1016/j.mechmachtheory.2013.11.012 [Open Access] G. Nawratil: Introducing the theory of bonds for Stewart Gough platforms with self-motions. ASME Journal of Mechanisms and Robotics 6 (1) 011004 (2014) DOI: 10.1115/1.4025623 H. Stachel:On the flexibility and symmetry of overconstrained mechanisms. Philosophical Transactions of the Royal Society A 372 20120040 (2014) DOI: 10.1098/rsta.2012.0040 G. Nawratil: On Stewart Gough manipulators with multidimensional self-motions. Computer Aided Geometric Design 31 (7-8) 582-594 (2014) DOI: 10.1016/j.cagd.2014.02.012 Contributions to Books/Proceedings G. Nawratil and H. Stachel: Composition of spherical four-bar-mechanisms. New Trends in Mechanisms Science (D. Pisla et al. eds.), pages 99-106, Springer, 2010, ISBN: 978-90-481-9688-3, DOI: 10.1007/978-90-481-9689-0_12 H. Stachel: The Influence of Geometry on the Rigidity or Flexibility of Structures. Proc. IWSSIP 2010 - 17th Internat. Conf. on Systems, Signals and Image Processing, Rio de Janeiro/Brazil 2010, pages 24-2, ISBN: 978-85-228-0565-5 G. Nawratil: Stewart Gough platforms with linear singularity surface. Proc. 19th IEEE Internat. Workshop on Robotics in Alpe-Adria-Danube Region (RAAD), Budapest/Hungary 2010, pages 231-235, ISBN: 978-1-4244-6884-3, DOI: 10.1109/RAAD.2010.5524579 G. Nawratil: Basic result on type II DM self-motions of planar Stewart Gough platforms. Mechanisms, Transmissions and Applications (E.Ch. Lovasz, B. Corves eds.), pages 235-244, Springer, 2011, ISBN: 978-94-007-2726-7, DOI: 10.1007/978-94-007-2727-4_21 H. Stachel:Remarks on flexible quad meshes. Proc. 11th Internat. Conf. on Engineering Graphics - BALTGRAF-11, Tallinn/Estonia 2011, pages 84-92, ISBN: 978-9949-23-112-6 G. Nawratil: Self-motions of planar projective Stewart Gough platforms. Latest Advances in Robot Kinematics (J. Lenarcic, M. Husty eds.), pages 27-34, Springer, 2012, ISBN: 978-94-007-4619-0, DOI: 10.1007/978-94-007-4620-6_4 G. Nawratil: Review and recent results on Stewart Gough platforms with self-motions. Applied Mechanics and Materials 162 151-160 (2012) DOI: 10.4028/www.scientific.net/AMM.162.151 G. Hegedüs, J. Schicho and H.-P. Schröcker: Construction of Overconstrained Linkages by Factorization of Rational Motions. Latest Advances in Robot Kinematics (J. Lenarcic, M. Husty eds.), pages 213-220, Springer, 2012, ISBN: 978-94-007-4619-0, DOI: 10.1007/978-94-007-4620-6_27 G. Hegedüs, J. Schicho and H.-P. Schröcker: Bond Theory and Closed 5R Linkages. Latest Advances in Robot Kinematics (J. Lenarcic, M. Husty eds.), pages 221-228, Springer, 2012, ISBN: 978-94-007-4619-0, DOI: 10.1007/978-94-007-4620-6_28 H. Stachel:A flexible quadrangular mesh tiling a cylinder of revolution. Proc. 15th Internat. Conf. on Geometry and Graphics, Montreal/Canada 2012, pages 711-719, ISBN: 978-0-7717-0717-9 G. Nawratil: Non-existence of planar projective Stewart Gough platforms with elliptic self-motions. Computational Kinematics (F. Thomas, A. Perez Garcia eds.), pages 49-57, Springer, 2013, ISBN: 978-94-007-7213-7, DOI: 10.1007/978-94-007-7214-4_6 G. Nawratil: Kinematic Mapping of SE(4) and the Hypersphere Condition. Advances in Robot Kinematics (J. Lenarcic, O. Khatib eds.), pages 11-19, Springer, 2014, ISBN: 978-3-319-06697-4, DOI: 10.1007/978-3-319-06698-1_2 [Open Access,Erratum] G. Nawratil: Congruent Stewart Gough platforms with non-translational self-motions. Proc. 16th Internat. Conf. on Geometry and Graphics (H.-P. Schröcker, M. Husty eds.), Innsbruck/Austria 2014, pages 204-215, ISBN: 978-3-902936-46-2 Abstracts S.N. Mikhalev: On a method for the construction of flexible suspensions with even number of equatorial edges (in Russian). Abstracts of Internat. Conf. "Metric Geometry of Surfaces and Polyhedra" dedicated to the Centennial Anniversary of N. V. Efimov, Moscow/Russia 2010, pages 47-48, ISBN: 978-5-317-03369-9 H. Stachel:Comments on flexible Kokotsakis meshes. Abstracts of Internat. Conf. "Metric Geometry of Surfaces and Polyhedra" dedicated to the Centennial Anniversary of N. V. Efimov, Moscow/Russia 2010, pages 82-83, ISBN: 978-5-317-03369-9 I.Kh. Sabitov: Volume polynomials for some Polyhedra in n-Space (Abstract of 10th Internat. Conf. on Geometry and Applications, Varna/Bulgaria 2011). Journal of Geometry 103 363 (2012) DOI: 10.1007/s00022-012-0130-6 G. Nawratil: Self-motions of parallel manipulators associated with flexible octahedra. Extended Abstract and Slides in Proc. of the Austrian Robotics Workshop (M. Hofbaur, M. Husty eds.), Hall in Tyrol/Austria 2011, pages 232-248, ISBN: 978-3-9503191-0-1 I.Kh. Sabitov: Two new classes of rigid polyhedra and their volume polynomials in the spaces of constant curvature. Abstracts of The Fourth Geometry Meeting Dedicated to the Centenary of A. D. Alexandrov, St. Petersburg/Russia 2012, pages 26-27, ISBN: 978-5-9651-0668-4 I.Kh. Sabitov: Volume polynomials for pyramids in the spaces of constant curvature (in Russian). Abstracts of Internat. Conf. "Geometry in Odessa-2012", Odessa/Ukraine 2012, page 65, ISBN: 978-966-389-171-2 I.Kh. Sabitov: Some new classes of rigid polyhedra. Abstracts of the Internat. Topological Conf. "Alexandroff Readings", Moscow/Russia 2012, pages 64-65 Theses written within the frame of the project G. Nawratil: Flexible octahedra in the projective extension of the Euclidean 3-space and their application. Habilitation thesis, Institute of Discrete Mathematics and Geometry, TU Vienna, 2011 D. Slutskiy: Metriques polyedrales sur les bords de varietes hyperboliques convexes et flexibilite des polyedres hyperboliques. These en vue de l'obtention du doctorat de l'Uneversite de Toulouse, Universite Toulouse III Paul Sabatier, 2013, Directeurs de these: J-M. Schlenker, V. Alexandrov All publications of G. Nawratil can be downloaded from his homepage. |
| | Related Publications H. Stachel: Zur Einzigkeit der Bricardschen Oktaeder. J. Geom. 28 (1987), 41-56. [Zbl], [MR]. I. Sabitov: Local theory on bendings of surfaces. In Geometry III. Theory of surface, volume 48 of Encycl. Math. Sci., pages 179-250. 1992. translation from Itogi Nauki Tekh., Ser. Sovrem. Probl. Mat., Fundam. Napravleniya 48, 196-270 (1989). [MR]. V. Alexandrov: An example of a flexible polyhedron with nonconstant volume in the spherical space.Beitr. Algebra Geom. 38/1 (1997), 11-18. [MR]. R. Connelly, I. Sabitov, and A. Walz: The Bellows conjecture.Beitr. Algebra Geom. 38/1 (1997), 1-10. [MR]. V. Alexandrov: Sufficient conditions for the extendibility of an n-th order flex of polyhedra.Beitr. Algebra Geom. 39/2 (1998), 367-378. [MR]. I. Sabitov: On some recent results in the metric theory of polyhedra. Rend. Circ. Mat. Palermo, II. Ser., Supplemento 65/II (2000), 167-177, Proceedings of the Third international conference in stochastic geometry, convex bodies and empirical measures, held in Mazara del Vallo, Italy, May 24-29, 1999. P. M. Gruber (Ed.). [MR]. H. Stachel: Higher order flexibility of octahedra. Period. Math. Hungar. 39 (1999), 225-240, Discrete geometry and rigidity (Budapest, 1999). [Zbl], [MR]. S. Mikhalev: Some necessary metric conditions for flexibility of suspensions. Mosc. Univ. Math. Bull. 56/3 (2001), 14-20. [MR]. H. Stachel: Flexible cross-polytopes in the Euclidean 4-space. J. Geom. Graphics 4 (2000), 159-167. [Zbl], [MR]. H. Stachel: Remarks on Bricard's flexible octahedra of type 3. In Proc. 10th ICGG, volume 1, pages 8-12, Kyiv, 2002. ISBN: 966-96185-2-5. held in Kiev (Ukraine), July 28 -- Aug. 3, 2002. H. Stachel: Ivory's theorem in the Minkowski plane. Math. Pannon. 13 (2002), 11-22. [Zbl], [MR]. H. Stachel and J. Wallner: Ivory's theorem in hyperbolic spaces. Sib. Math. J. 45 (2004), 785-794. [Zbl], [MR]. H. Stachel:Flexible Octahedra in the Hyperbolic Space. Proc. Janos Bolyai Conference in Hyperbolic Geometry, July 8-12, 2002, Budapest. In A. Prekopa, E. Molnár (eds.), Non-euclidean geometries: János Bolyai memorial volume, Mathematics and its applications, vol. 581, Springer, New York 2006 (ISBN: 0-387-29554-2) [MR]: pages 209-225, 2006. [MR]. V. Alexandrov, I.Kh. Sabitov and H. Stachel (eds.): Rigidity and Related Topics in Geometry. Special issue of European J. Combinatorics 31 (4) 1035-1204 (2010). H. Stachel: A kinematic approach to Kokotsakis meshes. Comput. Aided Geom. Des., 27 428-437 (2010). | | ---------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------- |
Quick Links
Stewart Gough Platforms with Self-Motions
Singularity Closeness of Stewart-Gough Platforms
External Links
 Russian Foundation for Basic Research (RFBR)
Russian Foundation for Basic Research (RFBR)
Copyright © 1996-2021 by Differential Geometry and Geometric Structures. All rights reserved.
Web design: Hans Havlicek, Udo Hertrich-Jeromin
(W3C) Last modified on Mon 26 Jul 2021, 09:42:59 CEST