The sources of Schwinger's Green's functions (original) (raw)

Abstract

Julian Schwinger's development of his Green's functions methods in quantum field theory is placed in historical context. The relation of Schwinger's quantum action principle to Richard Feynman's path-integral formulation of quantum mechanics is reviewed. The nonperturbative character of Schwinger's approach is stressed as well as the ease with which it can be extended to finite temperature situations.

In his introduction to the volume containing the addresses that were made at the three memorial symposia held after Julian Schwinger's death in 1994 (1), Ng observed that few physicists have matched Schwinger's contribution to, and influence on, the development of physics in the 20th century. As Paul C. Martin and Sheldon L. Glashow, two of Schwinger's most outstanding students, noted: “[Schwinger] set standards and priorities single-handedly...His ideas, discoveries, and techniques pervade all areas of theoretical physics” (1). Indeed in the post-World War II period Schwinger set the standards and priorities of theoretical physics. Schwinger was the first to make use of the renormalization ideas that had been advanced by Hendrik Kramers to formulate a fully relativistic version of quantum electrodynamics (QED) that was finite to the orders of perturbation theory that could be readily calculated. Frank Yang in his memorial address put it thus: Schwinger not only climbed the peak but in the process also fashioned the tools that made it possible for others to climb the peak. In the two articles under review (2, 3) Schwinger indicated how the perturbative, diagrammatic approach to the representation of quantum field theories (QFTs) that had been given by Richard Feynman and Freeman Dyson, could be generated from the functional equations satisfied by what Schwinger called the Green's functions. The Green's functions are vacuum expectation values of time-ordered Heisenberg operators, and the field theory can be defined nonperturbatively in terms of these functions. In a lecture delivered on the occasion of receiving an honorary degree from Nottingham University in 1993, Schwinger related his coming to these Green's functions (4). In the following I give a brief account of the background of these two articles (2, 3).

“Renormalization was one of the great peaks of the development of fundamental physics in this century. Scaling the peaks was a difficult enterprise. It required technical skill, courage, subtle judgments, and great persistence. Many people had contributed to this enterprise. Many people can climb the peak now. But the person who first conquered the peak was Julian Schwinger” (1).

QFT 1927–1945

The formulation of nonrelativistic quantum mechanics by Werner Heisenberg, Erwin Schrödinger, Paul Dirac, Pascual Jordan, and Max Born from 1925 to 1927 was a revolutionary achievement. Its underlying metaphysics was atomistic. Its success derived from the confluence of a theoretical understanding, the representation of the dynamics of microscopic particles by quantum mechanics, and the apperception of a quasi-stable ontology, namely, electrons, protons, and nuclei, the building blocks of the entities (atoms, molecules, and simple solids) that populated the domain that was being carved out. Quasi-stable meant that under normal terrestrial conditions electrons, protons, and nuclei could be treated as ahistoric objects, whose physical characteristics were seemingly independent of their mode of production and whose lifetimes could be considered as essentially infinite. Electrons, protons, and nuclei could be specified by their mass, spin, number, and electromagnetic properties such as charge and magnetic moment. In addition, the formalism could readily incorporate the consequence of the strict identity and indistinguishabilty of these “elementary” entities. Their indistinguishability implied that an assembly of them obeyed characteristic statistics depending on whether their spin is an integer or half odd integer multiple of Planck's constant, h.

In 1927 Dirac extended the formalism to include the interactions of charged particles with the electromagnetic field by describing the electromagnetic field as an assembly of photons. For Dirac, particles were the “fundamental” substance. In contradistinction, Jordan argued that fields were fundamental and advocated a unitary view of nature in which both matter and radiation were described by wave fields, with particles appearing as excitations of the field. Jordan's view was that the electromagnetic field was to be described by field operators that obeyed Maxwell equations and satisfied certain commutation relations. In practice this meant exhibiting the electromagnetic field as a superposition of harmonic oscillators, whose dynamical variables were then required to satisfy the quantum rules [_qm, pn_] = i_ℏδ_mn. These commutation rules in turn implied that in any small volume of space there would be fluctuations of the electric and magnetic field even in the absence of any free photons, and that the rms value of these fluctuations became larger and larger as the volume element one probed became smaller and smaller. Jordan advanced a unitary view of nature in which both matter and radiation were described by wave fields. The quantization of these wave fields then exhibited the particle nature of their quanta and thus elucidated the mystery of the particle-wave duality. An immediate consequence of Jordan's approach was an answer to the question: “Why are all particles of a given species (as characterized by their mass and spin) indistinguishable from one another?” The answer was that the field quantization rules made them automatically thus, because the “particles” were excitations of the same underlying field. (For references to the articles of the authors cited in this and the next section, see refs. 5 and 6.)

The creation and annihilation of particles, first encountered in the description of the emission and absorption of photons by charged particles, was a novel feature of QFT. Dirac's “hole theory,” the theory he constructed to describe relativistic spin ½ particles involved the creation and annihilation of matter. Dirac had recognized that the equation he had obtained in 1928 to describe relativistic spin ½ particles, besides possessing solutions of positive energy, also admitted solutions with negative energy. To avoid transitions to negative energy states, Dirac postulated that the state of lowest energy, the vacuum, be the state in which all of the negative energy states were occupied and noted that an unoccupied negative energy state (what Dirac called a hole) would correspond to “a new kind of particle, unknown to experimental physics, having the same mass and opposite charge to an electron” (7). Evidence that such “positrons” existed was given by Carl Anderson, Patrick Blackett, and Giuseppe Occhialini soon thereafter.

The field theoretic description of β-decay phenomena given by Enrico Fermi in 1933–1934 was an important landmark in the developments of QFT. The process wherein a radioactive nucleus emits an electron (β-ray) had been studied extensively experimentally. Wolfgang Pauli in the early 1930s had suggested the existence of a neutral, very light spin ½ particle that was emitted in the β-decay process to maintain energy and angular momentum conservation. Fermi then demonstrated that the simplest model of a theory of β-decay based on Pauli's suggestion implied that electrons do not exist in nuclei before β-emission occurs, but acquire their existence when emitted in the same way as photons in an atomic transition.

The discovery of the neutron by James Chadwick in 1932 suggested that atomic nuclei are composed of protons and neutrons. The existence of the neutron made possible the application of quantum mechanics to elucidate the structure of the nucleus. Heisenberg, Ettore Majorana, Eugene Wigner, and others formulated models of nuclear structure based on nucleon–nucleon interactions. Nuclear forces had to be strong and of very short range. In 1935 Hideki Yukawa proposed a QFT model of nuclear forces. Just as in QED in which the electromagnetic force between charged particles was conceptualized as caused by the exchange of “virtual” photons between them (called virtual because these photons do not obey the relation E = _h_ν valid for free photons) the exchange of a “meson” mediated the force between nucleons. Photons being massless made the range of electromagnetic forces infinite. In Yukawa's theory, the exchanged quanta are massive, resulting in interactions of finite range. The association of interactions with exchanges of quanta is a feature of all QFTs.

QED, Fermi's theory of β-decay, and Yukawa's theory of nuclear forces established the model on which subsequent developments were based. It postulated “impermanent” particles to account for interactions and assumed that relativistic QFT was the proper framework for representing processes at ever smaller distances. But relativistic QFTs are beset by divergence difficulties manifested in perturbative calculations beyond the lowest order. Higher orders yield infinite results. These difficulties stem from the fact that (i) the description is in term of local fields (i.e., fields that are defined at a point in space-time), which are systems with an infinite number of degrees of freedom, and (ii) the interaction between fields is assumed to be local. The locality of the interaction is expressed by interaction terms in the Hamiltonian of the form _j_μ(x)_A_μ(x) in QED, where _j_μ(x) is the current operator of the matter field and _A_μ(x) is that of the electromagnetic field.

In QED the local interaction terms imply that photons will couple with (virtual) electron-positron pairs of arbitrarily high momenta and result in divergences, and similarly electrons and positrons will couple with (virtual) photons of arbitrary high momenta and give rise to divergences. These problems impeded progress throughout the 1930s, and most of the workers in the field doubted the correctness of QFT in view of these divergence difficulties. Numerous proposals to overcome these problems were advanced during the 1930s, but all ended in failure. The pessimism of the leaders of the discipline (Niels Bohr, Pauli, Heisenberg, Dirac, and J. Robert Oppenheimer) was partly responsible for the lack of progress. They had witnessed the overthrow of the classical concepts of space-time and were responsible for the rejection of the classical concept of determinism in the description of atomic phenomena. They had brought about the quantum mechanical revolution and were convinced that only further conceptual revolutions would solve the divergence problem in QFT. In 1938 Heisenberg proposed that the next revolution be associated with the introduction of a fundamental unit of length, which would delineate the domain in which the concept of fields and local interactions would be applicable. The _S_-matrix theory that he developed in the early 1940s was an attempt to make this approach concrete. He observed that all experiments can be viewed as scattering experiments. In the initial configuration the system is prepared in a definite state. The system then evolves and the final configuration is observed after a time that is long compared with the characteristic times pertaining in the interactions. The _S_-matrix is the operator that relates initial and final states. Its knowledge allows the computation of scattering cross sections and other observable quantities. As in 1925, Heisenberg again suggested that only variables referring to experimentally ascertainable quantities enter theoretical descriptions. Because local field operators were not measurable, fundamental theories should find new modes of representations, and with his _S_-matrix theory he opened a new chapter in the development of QFTs.

QED 1947–1950

Shortly after the end of World War II an important advance in QFT stemmed from the attempt to explain quantitatively the discrepancies between the predictions of the Dirac equation for the level structure of the hydrogen atom and for the value it ascribed to the magnetic moment of the electron and the empirical data. These deviations had been measured in reliable and precise molecular beam experiments carried out by Willis Lamb and Isidor Rabi and their coworkers at Columbia University in New York and were reported at the Shelter Island Conference in June 1947. Shortly after the conference, it was shown by Hans Bethe that the Lamb shift (the deviation of the 2_s_ and 2_p_ levels of hydrogen from the values given by the Dirac equation) was of quantum electrodynamical origin, and that the effect could be computed by making use of what became known as mass renormalization, an idea that had been put forward by Hendrik Kramers.

The parameters for the mass, mo, and that for the charge, eo, that appear in the Lagrangian-defining QED are not the observed charge and mass of an electron. The observed mass, m, of the electron is to be introduced in the theory by the requirement that the energy of the physical state corresponding to a free electron moving with momentum p be equal to (_p_2 + _m_2)1/2. Similarly, the observed charge should be defined by the requirement that the force between two electrons (at rest) separated by a large distance r, be described by Coulomb's law, _e_2/_r_2, with e the observed charge of an electron. It was shown by Schwinger and Feynman that all of the divergences encountered in the low orders of perturbation theory could be eliminated by reexpressing the parameters mo and eo in terms of the observed values m and e, a procedure that became known as mass and charge renormalization. [For Schwinger's contributions to these developments, see refs. 6 and 8–12.] Feynman's contribution was a technique by which the perturbative content of a QFT could readily be visualized in terms of diagrams and that for a given physical process the contribution of each of the diagrams could readily be written down by virtue of certain correspondence rules. Furthermore, these diagrams furnished what Feynman called the “machinery” of the particular processes: they not only indicate the mechanism that explains why certain processes take place in particular systems but also how so by the exchange of quanta. Feynman's approach is defined by a limiting procedure. A cut-off is introduced into the theory so that the physics at momenta higher than some momentum (or equivalently the physics at distances shorter than some cut-off length) is altered, and all calculated quantities thereby rendered finite but cut-off dependent. The parameters of the cut-off theory are then expressed in terms of physically measurable quantities (such as the mass and charge of the particles that are described by the theory). Renormalized QED is the theory that is obtained by letting the cut-off length go to zero. The success of renormalized QED in accounting for the Lamb shift, the anomalous magnetic moment of the electron and the muon, the radiative corrections to the scattering of photons by electrons, to pair production and bremsstrahlung, was spectacular.

In 1948 Dyson was able to show that such charge and mass renormalizations were sufficient to absorb all of the divergences of the scattering matrix (_S_-matrix) in QED to all orders of perturbation theory. More generally, Dyson demonstrated that only for certain kinds of QFTs is it possible to absorb all the infinities by a redefinition of a finite number of parameters. He called such theories renormalizable. Renormalizability thereafter became a criterion for theory selection. Perhaps the most important legacy of the 1947–1952 period was providing the theoretical physics community with a firm foundation for believing that local QFT was the framework best suited for the unification of quantum theory and special relativity and the description of nature at a foundational level.

The challenge on how to formulate local QFTs based ab initio on a quantum action principle was taken up by Schwinger in a series of articles starting in 1950 (refs. 13–15; see also ref. 16). He wanted to get away from quantization procedures that were expressed as a set of operator prescriptions imposed on a classical description based on a Hamiltonian. This approach for the field theoretic case produced an asymmetry in the treatment of time and space and resulted in a formulation that was noncovariant. Additionally, it placed the existence of anticommuting Fermi–Dirac fields on a purely empirical basis to be introduced into the formalism on an ad hoc basis. Schwinger's point of departure was the articles of Feynman in which he had formulated quantum mechanics by exhibiting the probability amplitude connecting the state of a system at one time to that at a later time as a sum of complex unit amplitudes (one for each possible trajectory of the system connecting the initial and final state), the phase of each amplitude being determined by the value of the action, ∫ L dt, for that trajectory. [See ref. 15.] Furthermore, because action is a relativistic invariant the formulation could thus be made covariant. But whereas Feynman gave a global solution, for Schwinger,

“The idea from the beginning was not, as Feynman would do, to write down the answer, but to continue in the grand tradition of classical mechanics, but only as a historical model, to find a differential, an action principle formulation. What is Hamilton's principle or its generalization in quantum physics? If you want the time transformation function, do not ask what it is but how it infinitesimally changes. The distinction [with Feynman's approach] comes [because] this deals with all kinds of quantum variations, on exactly the same footing, which means from a field point of view not only do Bose–Einstein fields appear naturally but Fermi–Dirac fields. Whereas with the path integral approach with its clear connection to the correspondence principle, the anticommuting Fermi system appears out of nowhere, there is no logical reason to have it except that one knows one has to. It does not appear as a logical possibility as it does with the differential.”

Schwinger (ref. 12, p. 316)

Recall that a classical field theory can be fully specified by its Lagrangian density

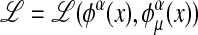graphic file with name M1.gif [1]

the field, or fields, being described by variables φα(x) that are defined at every point of space-time x, with φαμ(x) = ∂φα(x)/∂_x_μ. The dynamics of the field system is then determined by an action principle that stipulates that the functional

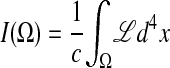graphic file with name M2.gif [2]

defined over the space-time volume Ω is stationary for the physically realizable field configurations. I(Ω) is said to be stationary if the variation φα → φα + δφα for arbitrary δφα that vanish on the boundary of Ω produces no change in δ_I_ to first order in δφα. The action principle then yields the fields' equations of motion:

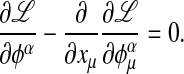graphic file with name M3.gif [3]

It is usually assumed that ℒ depends only quadratically on 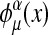Inline graphic so that the field equations are at most of second order. For physically interesting situations the space-time region Ω is bounded by two space-like surfaces, σ1 and σ2. [A space-like surface is one on which every two points, _x, x_′ are separated by a space-like distance, i.e., (_x_μ – _x_μ′)2 > 0.] The state of the field system is then completely specified by giving the values of φα(x) on σ1 and σ2 or the values of φα(x) and _n_μφαμ(x) on σ1, _n_μ being the normal to σ1.

One can consider more general kinds of variation whereby not only the φα are varied but also the boundary of Ω, each point thereof being moved from _x_μ to _x_μ + δ_x_μ; δφα is then defined as

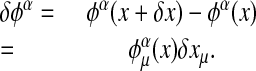graphic file with name M5.gif [4]

Under this more general variation

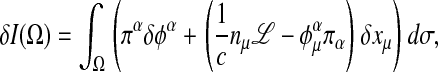graphic file with name M6.gif [5]

where

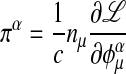graphic file with name M7.gif [6]

is the momentum conjugate to φα defined at x and on σ. Quantization is then imposed by stipulating commutation rules between π α(x) and φα(_x_′) for _x, x_′ on σ1:

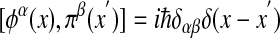graphic file with name M8.gif [7]

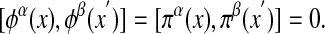
graphic file with name M9.gif

In the quantized theory the operators φα(x) obey the same equations of motion as the classical fields. But because the field operators satisfy commutation rules a state of the field system is specified by only giving the eigenvalues, φ′α, of the field operators on one space-like surface, the latter forming a complete set of commuting operators on that surface, because fields at different points of a spacelike surface commute with one another as indicated by the commutation rules [φα(x), φβ(_x_′)] = 0 for _x, x_′ on σ. Such a state is denoted by the Dirac ket vector |φ′α, σ〉. 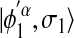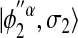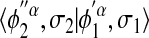The transition probability from the state Inline graphic to the state Inline graphic is given by the modulus squared of the amplitude Inline graphic.

Feynman, based on some previous work of Dirac, derived a readily visualizable expression for the transition amplitude for systems with a finite number of degrees of freedom. For a single particle it reduces to the following prescription for evaluating the probability amplitude 〈_q_″, _t_2|_q_′, _t_1〉 for finding the particle at _q_″ at time _t_2 if it was at _q_′ at time _t_1:

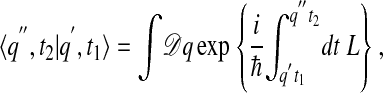graphic file with name M13.gif [8]

where the 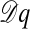Inline graphic integration is understood as a summation over all curves with fixed end points _q_″ at _t_2 and _q_′ at _t_1. Each curve (path) contributes to the sum of a unit amplitude, the phase of which being determined by the value of the action

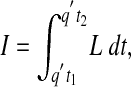
graphic file with name M15.gif

evaluated for that path, with L being the classical Lagrangian for the system

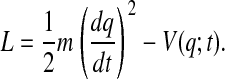graphic file with name M16.gif [9]

The correspondence between Feynman's formulation and the usual formulation is established by defining the Heisenberg operator q(t) by

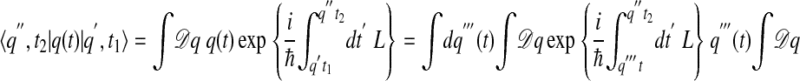graphic file with name M17.gif [10]

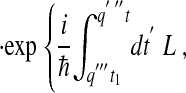
graphic file with name M18.gif

where on the right side of Eq. 10 q(t) is a c–number, whereas on the left side it is a Heisenberg picture operator.

The formalism allows a very useful extension. (See chapter 1 of ref. 18.) If in addition to V(q, t) an external force is taken to act on the system and the Lagrangian is assumed to be of the form

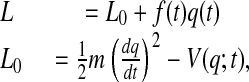graphic file with name M19.gif [11]

then under those circumstances the functional derivative of 〈_q_″, _t_2|_q_′, _t_1〉 with respect to the external force coupled to q(t) yields the matrix element of the Heisenberg operator q(t)

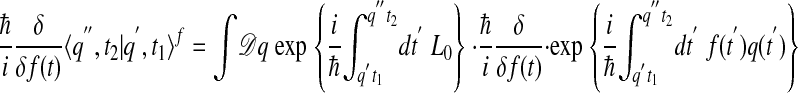graphic file with name M20.gif [12]

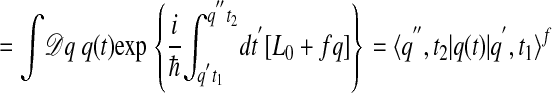
graphic file with name M21.gif

for _t_2 > t > _t_1. Similarly one readily derives that

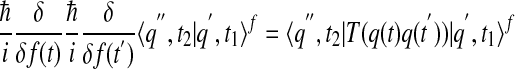graphic file with name M22.gif [13]

in which T(----) is the time-ordered product defined by

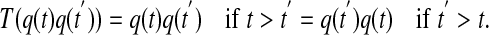graphic file with name M23.gif [14]

This result generalizes to

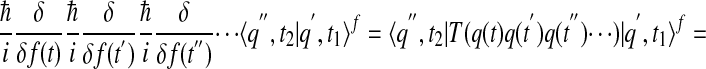graphic file with name M24.gif [15]

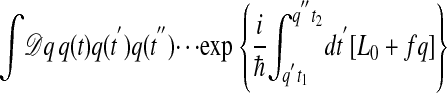
graphic file with name M25.gif

with T rearranging the operators appearing within the parenthesis in an ordered form with ascending times to the left:

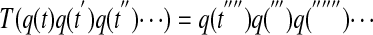graphic file with name M26.gif [16]

Feynman's formulation can readily be formally extended to the case of a field system. (See in particular ref. 19.)

The transition amplitude 〈φ2″α, σ2|φ1′α, σ1〉 is then written as

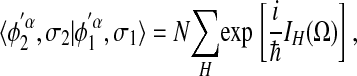graphic file with name M27.gif [17]

where the sum on the right extends over all possible histories of the fields between σ1 and σ2. A history H is specified by φ′α(x) taking on the values φ2″α on σ2 and the values φ1′α on σ1 and any value in between. In the limit over all paths, the path-integral is a continuously infinite sum to which can be given a rigorous mathematical meaning in some cases, e.g., some field theories in one space and one time dimension. N is a normalization factor designed to guarantee that

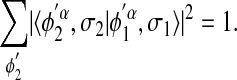graphic file with name M28.gif [18]

Again to make explicit the connection between the path-integral formulation and the usual formulation of QFT, the (Heisenberg picture) operator corresponding to φα is defined by the formula

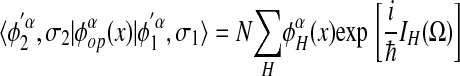graphic file with name M29.gif [19]

with φα_H_ being the value φα(x) takes for the particular path.

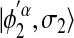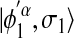Schwinger's point of departure was the observation that the states Inline graphic and Inline graphic are related by a unitary transformation:

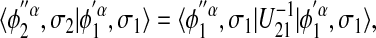graphic file with name M32.gif [20]

which for infinitesimal changes becomes

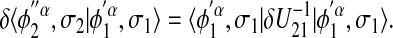graphic file with name M33.gif [21]

Because 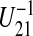Inline graphic is unitary (i/ℏ) _U_21 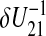Inline graphic is hermitian, and Schwinger thus wrote

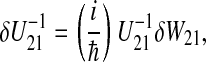graphic file with name M36.gif [22]

where δ_W_21 is an infinitesimal hermitian operator whose composition law is readily established to be given by

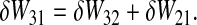graphic file with name M37.gif [23]

Schwinger's basic postulate is that δ_W_21 can be obtained by variations of the quantities contained in the Hermitian operator

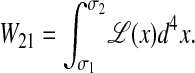graphic file with name M38.gif [24]

The result

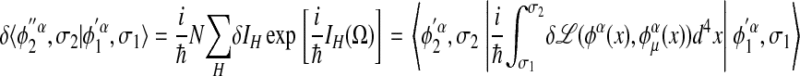graphic file with name M39.gif [25]

is, of course, the same as would be obtained formally from Feynman's sum over histories approach, with the right side of the first line being defined by classical variables and the second line defining the corresponding operators.

If the parameters of the system are not altered, the variation of the transformation function

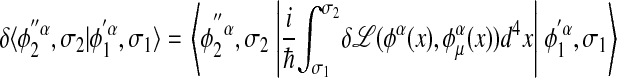graphic file with name M40.gif [26]

arises only from infinitesimal changes of 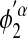Inline graphic, σ2 and 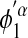Inline graphic, σ1. These are generated by operators associated with the surfaces σ1 and σ2, respectively,

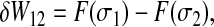graphic file with name M43.gif [27]

which is the operator principle of stationary action as it states that the action integral operator is not changed by infinitesimal variations of the field operators in the interior of the region bounded by σ1 and σ2. The field equations follow

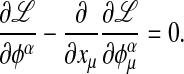graphic file with name M44.gif [28]

Conservation laws are associated with variations that leave the action integral unchanged, and Eq. 27 then implies that the generators that induce the variations are constant. Infinitesimal displacements and rotation of the surface are generated by the momentum and angular momentum operators, respectively, and as they leave the action invariant they are constants of the motion. Schwinger was also able to deduce the commutation rules that hold on a plane surface σ

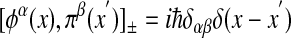graphic file with name M45.gif [29]

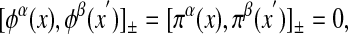
graphic file with name M46.gif

where the commutator applies for Boson fields and the anticommutator for Fermion fields. (For details see ref. 16; also available at www.nobel.se/nobel/nobel-foundation/publications/lectures.)

Schwinger's 1951 PNAS Articles

Schwinger's article “The Theory of Quantized Fields,” which was submitted to Physical Review in March of 1951 and appeared in June of 1951 (15), was one of four in which nonperturbative methods play a central role. His article on “Gauge Invariance and Vacuum Polarization,” which he sent to Physical Review in December 1951 (14), deals with the description of the quantized electron-positron field in an external field. He there introduced a description in terms of Green's functions, what Feynman had called propagators, but one that did not depend on a perturbative expansion. The one-particle Green's function

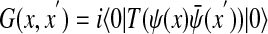graphic file with name M47.gif [30]

is defined in terms of Heisenberg operators that satisfy the equation of motion

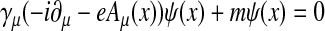graphic file with name M48.gif [31]

and the equal-time anticommutation rules

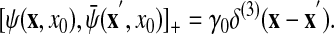graphic file with name M49.gif [32]

In Eq. 30 the state |0〉 is the vacuum state, the lowest energy state of the interacting system. In Eq. 30 the T product for anticommuting operators is defined as

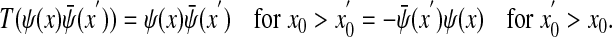graphic file with name M50.gif [33]

In the two articles entitled “On the Green's Functions of Quantized Fields I and II,” which Schwinger submitted in May 1951 to PNAS (2, 3), he gave a preliminary account of a general theory of Green's function “in which the defining property is taken to be the representation of the fields of prescribed sources.” This is the generalization to field systems of the introduction of external forces coupled linearly to q(t) in the case of particle motion as described in Eqs. 12–15. Thus, Schwinger took the gauge-invariant Lagrangian for the coupled Dirac and Maxwell field to include external sources linearly coupled to each field so that

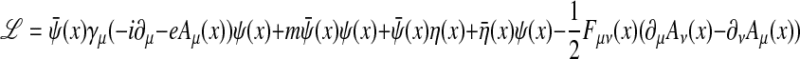graphic file with name M51.gif [34]

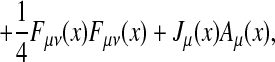
graphic file with name M52.gif

where the source spinor η(x) anticommutes with the Dirac field operators. In keeping with his requirement that only first-order variables appear in a quantum Lagrangian, F_μ_v(x) and _A_μ(x) are treated as independent fields. The equations of motion are then

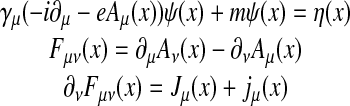graphic file with name M53.gif [35]

with

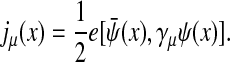graphic file with name M54.gif [36]

Schwinger restricted himself to changes in the transformation function that arise from variations of the external sources. In terms of the notation

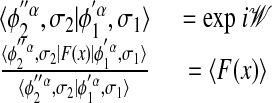graphic file with name M55.gif [37]

the dynamical principle can be written as

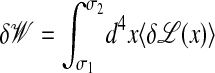graphic file with name M56.gif [38]

with

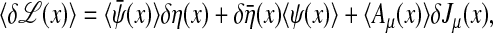
graphic file with name M57.gif

and the one-particle Green's function

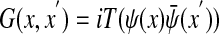graphic file with name M58.gif [39]

can be formally defined as

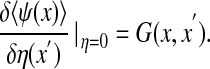graphic file with name M59.gif [40]

Schwinger then proceeded to derive a functional equation for the one-particle Dirac Green's function G(_x, x_′),

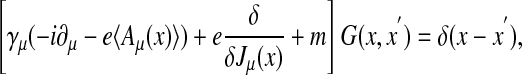graphic file with name M60.gif [41]

and for the one-photon Green's function

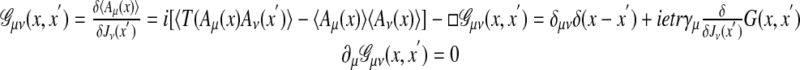graphic file with name M61.gif [42]

as well as for the two-particle Green's function

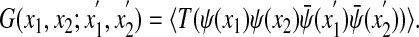graphic file with name M62.gif [43]

The equation satisfied by the two-particle Green's function

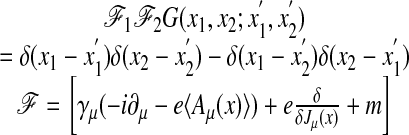graphic file with name M63.gif [44]

is known as the Bethe–Salpeter equation and was first derived by Yoichiro Nambu.

In his second PNAS article (3) Schwinger determined the boundary conditions that the Green's functions satisfied when the initial and final states on σ1 and σ2 were the vacuum state. When the external fields vanish in the neighborhood of σ1 and σ2, the one-particle Green's function, now denoted by G+, contains only positive frequency for x on σ2 and only negative frequencies for x on σ1. By letting the surfaces σ1 and σ2 recede to the infinite past and infinite future, respectively, and introducing an integral representation for the action of the functional derivatives

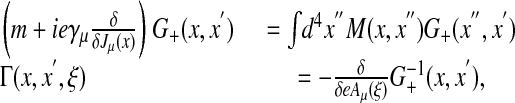graphic file with name M64.gif [45]

the equations that the one-particle Green's functions for the electron and the photon, now denoted by G+ and 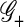Inline graphic, can be written in closed form:

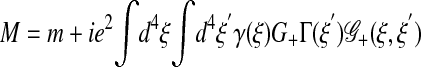
graphic file with name M66.gif

and

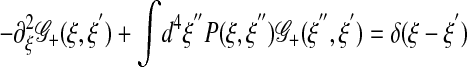graphic file with name M67.gif [46]

with

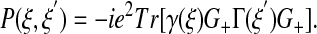
graphic file with name M68.gif

(See equations 30–43 of ref. 3 and ref. 16 for a clarification of the notation.) Eqs. 45 and 46 are “closed” functional differential equations for the electron and photon one-particle propagators and for the vertex and polarization function. They describe the theory and lead directly to a perturbation expansions in terms of “true” propagators. Similarly, the graphical, perturbative version of QED that Feynman and Dyson had elaborated can readily be obtained from them.

Schwinger's formulation of relativistic QFTs in terms of Green's functions was a major advance in theoretical physics. It was a representation in terms of elements (the Green's functions) that were intimately related to real physical observables and their correlation. It gave deep structural insights into QFTs; in particular, it allowed the investigation of the structure of the Green's functions when their variables are analytically continued to complex values, thus establishing deep connections with statistical mechanics. The extension of the methods to treat nonrelativistic multiparticle systems from a QFT viewpoint, as presented by Martin and Schwinger (20), has likewise proven deeply influential.

This Perspective is published as part of a series highlighting landmark papers published in PNAS. Read more about this classic PNAS article online at www.pnas.org/classics.shtml.

References