cos - Cosine of argument in radians - MATLAB (original) (raw)

Cosine of argument in radians

Syntax

Description

[Y](#bt5p4al-1-Y) = cos([X](#bt5p4al-1-X)) returns the cosine for each element of X. The cos function operates element-wise on arrays. The function accepts both real and complex inputs.

For real values of X, cos(X) returns real values in the interval [-1, 1].
For complex values of X,cos(X) returns complex values.

Examples

collapse all

Plot the cosine function over the domain -π≤x≤π.

x = -pi:0.01:pi; plot(x,cos(x)) grid on

Figure contains an axes object. The axes object contains an object of type line.

Calculate the cosine of the complex angles in vector x.

x = [-i pi+ipi/2 -1+i4]; y = cos(x)

y = 1×3 complex

1.5431 + 0.0000i -2.5092 - 0.0000i 14.7547 +22.9637i

Input Arguments

collapse all

Input angle in radians, specified as a scalar, vector, matrix, multidimensional array, table, or timetable.

Data Types: single | double | table | timetable
Complex Number Support: Yes

Output Arguments

collapse all

Cosine of input angle, returned as a real-valued or complex-valued scalar, vector, matrix, multidimensional array, table, or timetable.

More About

collapse all

The cosine of an angle, α, defined with reference to a right triangle is

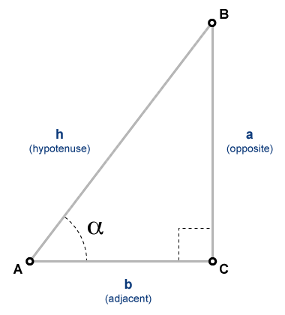
Right triangle with vertices A, B, and C. The vertex A has an angle α, and the vertex C has a right angle. The hypotenuse, or side AB, is labeled as h. The opposite side of α, or side BC, is labeled as a. The adjacent side of α, or side AC, is labeled as b. The cosine of α is defined as the adjacent side b divided by the hypotenuse h.

The cosine of a complex argument, α, is

Tips

To compute cos(X*pi) accurately, without usingpi as a floating-point approximation of π, you can use the cospi function instead. For example,cospi(m/2) is exactly zero for odd integersm and cospi(n) is +1 or –1 for integers n.

Extended Capabilities

expand all

Thecos function fully supports tall arrays. For more information, see Tall Arrays.

The cos function fully supports GPU arrays. To run the function on a GPU, specify the input data as a gpuArray (Parallel Computing Toolbox). For more information, see Run MATLAB Functions on a GPU (Parallel Computing Toolbox).

Version History

Introduced before R2006a

expand all

The cos function can calculate on all variables within a table or timetable without indexing to access those variables. All variables must have data types that support the calculation. For more information, see Direct Calculations on Tables and Timetables.