wavefronts (original) (raw)
Author: the photonics expert
Definition: surfaces connecting points with the same phase of a wave
Alternative term: phase fronts
DOI: 10.61835/l8a Cite the article: BibTex plain textHTML Link to this page LinkedIn
Wavefronts are surfaces connecting points with the same phase of a monochromatic wave. For example, one may select all those points where the maximum of the oscillating quantity within an oscillation cycle (for example, the maximum air pressure for a sound wave or the maximum positive electric field strength for an optical wave) is reached. Any other phase value could also be chosen, however.
Wavefronts are sometimes also called phase fronts; the terms can be used interchangeably. For ultrashort pulses, the term phase fronts may emphasize more the difference to pulse fronts. The latter are indicating maxima of the optical power rather locations of same optical phase.
The mathematically simplest example of wavefronts is that of a plane wave, where the wavefronts are planes. As a physically more realistic example, which is also a quite common case in optics, we can take a Gaussian beam. Figure 1 shows its color-coded complex amplitude profile:

Figure 1: Color-coded complex amplitude profile of a Gaussian beam.
Figure 2 shows the corresponding wavefronts, chosen to be the points with the darkest red:
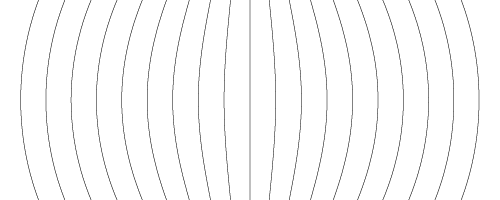
Figure 2: Wavefronts of a Gaussian beam for a particular moment of time.
These wavefronts are valid only for a certain moment in time; they propagate with the phase velocity. (For light propagating in vacuum or air, the phase velocity is a little less than 300 000 km/s.) In a medium, that velocity is in most cases lower, in a few cases higher (→ superluminal transmission), and it may deviate from the group velocity, which is the velocity of moving wave packets.
In our simple example, the wavefronts are exactly flat in the beam waist (in the middle of the diagram). This is not the case for light beams with non-perfect beam quality (see below).
In contrast to the amplitude image, the wavefronts do not contain any information on wave amplitudes, but only on the phase structure. In practice, one often stops drawing them in outer regions of a beam where the optical intensity becomes very weak, or where there are no longer of interest.
Generally, the spacing between subsequent wavefronts is taken to be the wavelength. Precisely, however, this holds only for plane waves, not e.g. for Gaussian beams.
Various Details
Wavefronts play an important role in wave optics. Note, however, that wavefronts are generally defined only for monochromatic waves, since they are based on the concept of the phase, which is not clearly defined for polychromatic waves. In such cases, one can still consider the wavefronts for particular optical frequency components.
The wave vector is always perpendicular to the wavefronts. For general beams with curved wavefronts, its direction and magnitude may depend on the position.
In isotropic optical materials, the optical energy always propagates in a direction parallel to the wave vector, i.e., perpendicular to the wavefronts. This is generally not true in anisotropic media, where one may have a spatial walk-off. That effect is relevant for nonlinear frequency conversion processes in certain nonlinear crystal materials; generally, those need to be anisotropic.
The essential function of various types of optical elements is to modify the wavefronts of light beams. For example, a lens can change the wavefront curvature such that an originally collimated beam is focused, or an originally divergent beam is collimated or refocused. Strong wavefront changes can also occur in the context of refraction and diffraction of light. For example, wavefronts generally modify their direction at optical interfaces where refraction occurs.
In many cases, it is technically preferable to maintain wavefronts with a smooth and simple shape, e.g. close to a parabolic or spherical shape. This together with a smooth amplitude profile of a laser beam, for example, results in a high beam quality, which implies that such a wave can be relatively well focused to a small area. Wavefront quality is also associated with the degree of spatial coherence.
Many lasers can produce beams with very high beam quality. However, various effects (e.g. thermal lensing in laser crystals or poor-quality optical elements) can cause serious wavefront distortions, also called wavefront aberrations or wavefront errors. Optical aberrations are also an important topic in the context of optical imaging.
Wavefronts and wavefront errors are of particular importance in the area of interference phenomena, where the optical phase is essential.
There are various types of optical wavefront sensors (e.g. Shack–Hartmann wavefront sensors) with which one can measure the shape of wavefronts. adaptive optics deals with methods for correcting wavefronts based on measuring them and acting on them, for example with deformable mirrors.
Wavefronts can be strongly scrambled using various kinds of optical diffusers.
More to Learn
Encyclopedia articles:
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him, e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
 general optics
general optics