Calculating Modes of a Germanosilicate Fiber With the Software RP Fiber Power (original) (raw)

RP Fiber Power – Simulation and Design Software for Fiber Optics, Amplifiers and Fiber Lasers
Power Form: Mode Properties of a Germanosilicate Fiber
This Power Form allows to conveniently calculate properties of guided modes of a germanosilicate fiber with given GeO2 concentration profile.
Germanosilicate is a mixture of fused silica (SiO2) and germania (GeO2), specified by the molar concentration (percentage value) of germania (considered as the dopant). The higher that concentration, the higher is the resulting refractive index. Many silica fibers simply have a germanosilicate fiber core within a pure silica cladding. The radial concentration profile can be tailored to optimize certain mode properties.
Demo Video
One of our case studies has been produced using the Power Form described here:
Your browser does not support the video tag. However, you can download the video file.
Defining the GeO2 Concentration Profiles
You have three options:
Single Step Profile
When you choose the profile type single step, you can simply enter core diameter and the GeO2 concentration of core and cladding:

Although the cladding will usually not contain any GeO2, the form works as well for doped claddings.
Multi-step Profile
When you choose the profile type multiple steps, you can enter up to eight radial segments with different doping concentrations:

Concentration Expression
For still more flexibility, you can define the doping concentration vs. radial position r in the fiber core with an expression:
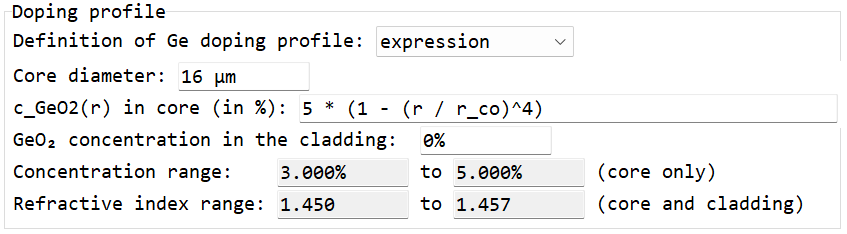
In this example case, we have 5% doping at the center (r = 0) and a smooth transition to zero doping at the core/cladding interface.
Display of Mode Properties
Below the index definition area, the form displays a table with calculated mode properties for a given wavelength:

For each mode, you get the following:
- mode name (according to the naming convention for LP modes)
- phase constant <$\beta$> (imaginary part of the propagation constant), telling you how fast the optical phase changes during propagation
- effective refractive index <$n_{\rm eff}$>: calculated from the phase constant
- group index <$n_{\rm g}$>: related to the group velocity of light in the fiber
- effective mode area <$A_{\rm eff}$>
- fraction (percentage) of optical power which propagates within the fiber core
Besides, the _M_2 factor of the fundamental mode is displayed. It is often close to 1, but in some cases with substantial deformed mode profile it may also be substantially higher.
Diagrams
The form offers several diagrams for displaying mode properties:

Just select those which you need, and (in the right column) select certain options.
The following screenshots show you for our last example case (graded concentration profile) what you can get from this form:



Remark: Above we show that diagram within the window where it is presented. There, you can choose one diagram version for each fiber mode.




Remark: the highest-order mode (LP12) “explodes” when approaching its cut-off; it then extends far into the fiber cladding. To some extent, this is already seen in a screenshot above (for 1064 nm).

You see that the highest-order mode has only weak overlap with the fiber core near its cut-off.

The effective indices are somewhere between the refractive index of the material composition with minimum and maximum GeO2 content.

Note that while higher-order modes often have higher group indices (lower group velocities) than low-order modes, LP12 is a remarkable exception in this case. It becomes relatively fast, since it propagates mostly within the fiber cladding. However, it also shows strong group velocity dispersion:


The last diagram shows how much time pulses would require to pass through 1 km of fiber, and what the resulting pulse duration is if we start with unchirped 10-ps Gaussian pulses.

Further Extensions
If you need similar calculations for a different material system – for example, fibers containing fluorinated fused silica for an index-depressed fiber cladding –, it will be no problem to make extended versions of the form, assuming that we can find the required material data. If the extension is of sufficiently broad interest, we may develop it ourselves, following your suggestion. Otherwise, you can do the extension yourself, or let us do it within the technical support.
Case Studies
The following case studies are available, where we used this Power Form:
Case Study: Dispersion Engineering for Telecom Fibers
We explore different ways of optimizing refractive index profile for specific chromatic dispersion properties of telecom fibers, resulting in dispersion-shifted or dispersion-flattened fibers. This also involves automatic optimizations.
#modes#dispersion#telecom#video
See also: overview on Power Forms
